A simple pendulum is constructed by attaching a bob of mass m to a string of length L fixed at its upper end. The bob oscillates in a vertical circle. It is found that the speed of the bob is v when the string makes an angle with the vertical. Find the tension in the string at this instant.
To find the tension in the string of a simple pendulum when the bob is at an angle θ with the vertical and moving with speed v, we can follow these steps:
1. Identify Forces Acting on the Bob:
- The forces acting on the bob are:
- The gravitational force (weight) acting downwards: Fg=mg
- The tension in the string acting along the string towards the pivot point: T
2. Resolve the Gravitational Force:
- The gravitational force can be resolved into two components:
- A component acting along the direction of the tension: Fg,∥=mgcos(θ)
- A component acting perpendicular to the tension (towards the center of the circular path): F_{g,\perpendicular} = mg \sin(\theta)
3. Apply Newton's Second Law:
- For circular motion, the net force towards the center of the circle is given by the centripetal force, which is provided by the difference between the tension and the component of the weight acting along the string:
T−mgcos(θ)=mv2L
- Here, mv2L is the centripetal force required to keep the bob moving in a circular path of radius L.
4. Rearranging the Equation:
- To find the tension T, rearrange the equation:
T=mgcos(θ)+mv2L
5. Final Expression for Tension:
- The final expression for the tension in the string when the bob makes an angle θ with the vertical and has a speed v is:
T=mgcos(θ)+mv2L
- The forces acting on the bob are:
- The gravitational force (weight) acting downwards: Fg=mg
- The tension in the string acting along the string towards the pivot point: T
- The gravitational force can be resolved into two components:
- A component acting along the direction of the tension: Fg,∥=mgcos(θ)
- A component acting perpendicular to the tension (towards the center of the circular path): F_{g,\perpendicular} = mg \sin(\theta)
- For circular motion, the net force towards the center of the circle is given by the centripetal force, which is provided by the difference between the tension and the component of the weight acting along the string:
T−mgcos(θ)=mv2L
- Here, mv2L is the centripetal force required to keep the bob moving in a circular path of radius L.
- To find the tension T, rearrange the equation:
T=mgcos(θ)+mv2L
- The final expression for the tension in the string when the bob makes an angle θ with the vertical and has a speed v is:
T=mgcos(θ)+mv2L
Topper's Solved these Questions
- BOOK - HC VERMA ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Objective -116videos
CIRCULAR MOTION
- BOOK - HC VERMA ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Objective -26videos
CIRCULAR MOTION
- BOOK - HC VERMA ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Question for short Answer10videos
CIRCULAR MOTION
- BOOK - HC VERMA ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONEXERCISE - Objective -211videos
CENTRE OF MASS, LINEAR MOMENTUM, COLLISION
- BOOK - HC VERMA ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - All Questions90videos
FLUID MECHANICS
Similar Questions
A stone tied to a string of length l is whirled in a horizontal circle at a constant angular speed ω in a circle of radius r. If the string makes an angle θ with vertical then the tension in the string is :
View SolutionA pendulum bob of mass m charge q is at rest with its string making an angle θ with the vertical in a uniform horizontal electric field E. The tension in the string in
View SolutionA simple pendulum is released from rest with the string in horizontal position. The vertical component of the velocity of the bob becomes maximum, when the string makes an angle θ with the vertical. The angle θ is equal to
View SolutionA pendulum bob of mass m hangs from one end of a horizontal rod which is rotating about vertical axis with an unknown angular speed ω such that the light inextensible string of length l of the pedulum makes a constant angle θ with vertical. Find the
Q. tension in the string
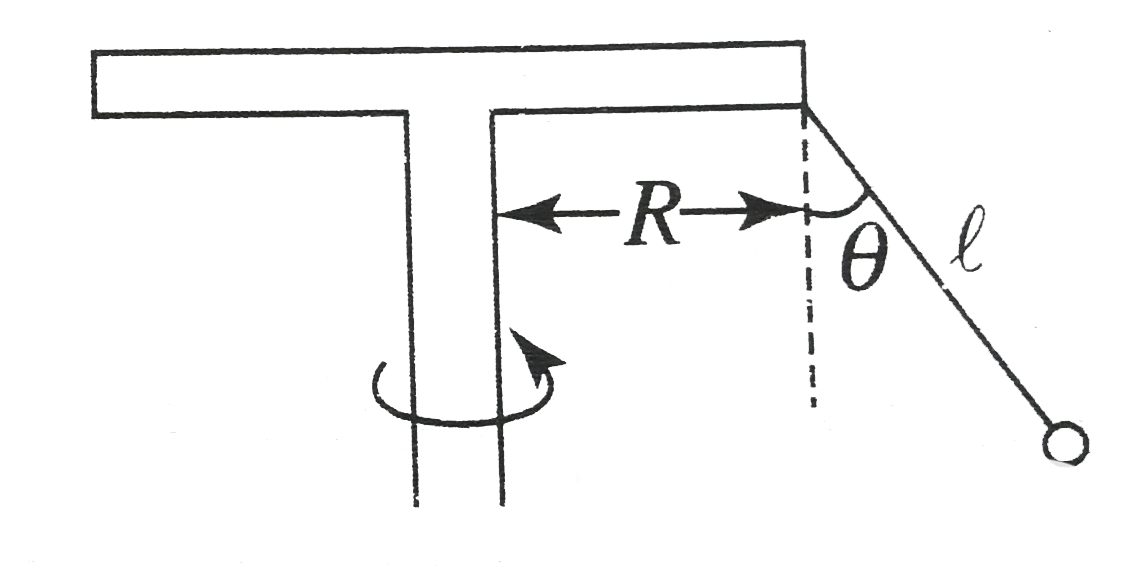 View Solution
View SolutionThe string of a pendulum is horizontal. The mass of the bob is m. Now the string is released. The tension in the string in the lowest position is -
View SolutionA particle of mass m is attached to the celling of a cabin with an inextensible light string of length l. The cabin is moving upward with an acceleration a . The particle is taken to a position such that string makes an angle θ with vertical. When string becomes vertical, find the tension in the string.
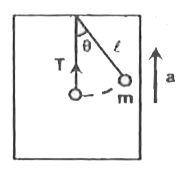 View Solution
View SolutionA pendulum consists of a small mass m at the end of a string of length L. The pendulum is pulled aside making an angle θ with the vertical and released. Taking the suspension point as the axis, at the instant of release
View SolutionLet θ denote the angular displacement of a simple pendulum oscillating in a vertical plane. If the mass of the bob is m, the tension in the string is mgcosθ
View SolutionA bob of mass m attached to an inextensible string of length I is suspended from a vertical support. The bob rotates in a horizontal circle with an angular speed ω red/s about the vertical. About the point of suspension:
View SolutionWhat is the torque acting on the bob of mass m of a simple pendulum Of length l, when the string of the pendulum makes an angle θ with the vertical ? When is the torque (i) zero and (ii) maximum.
View Solution
