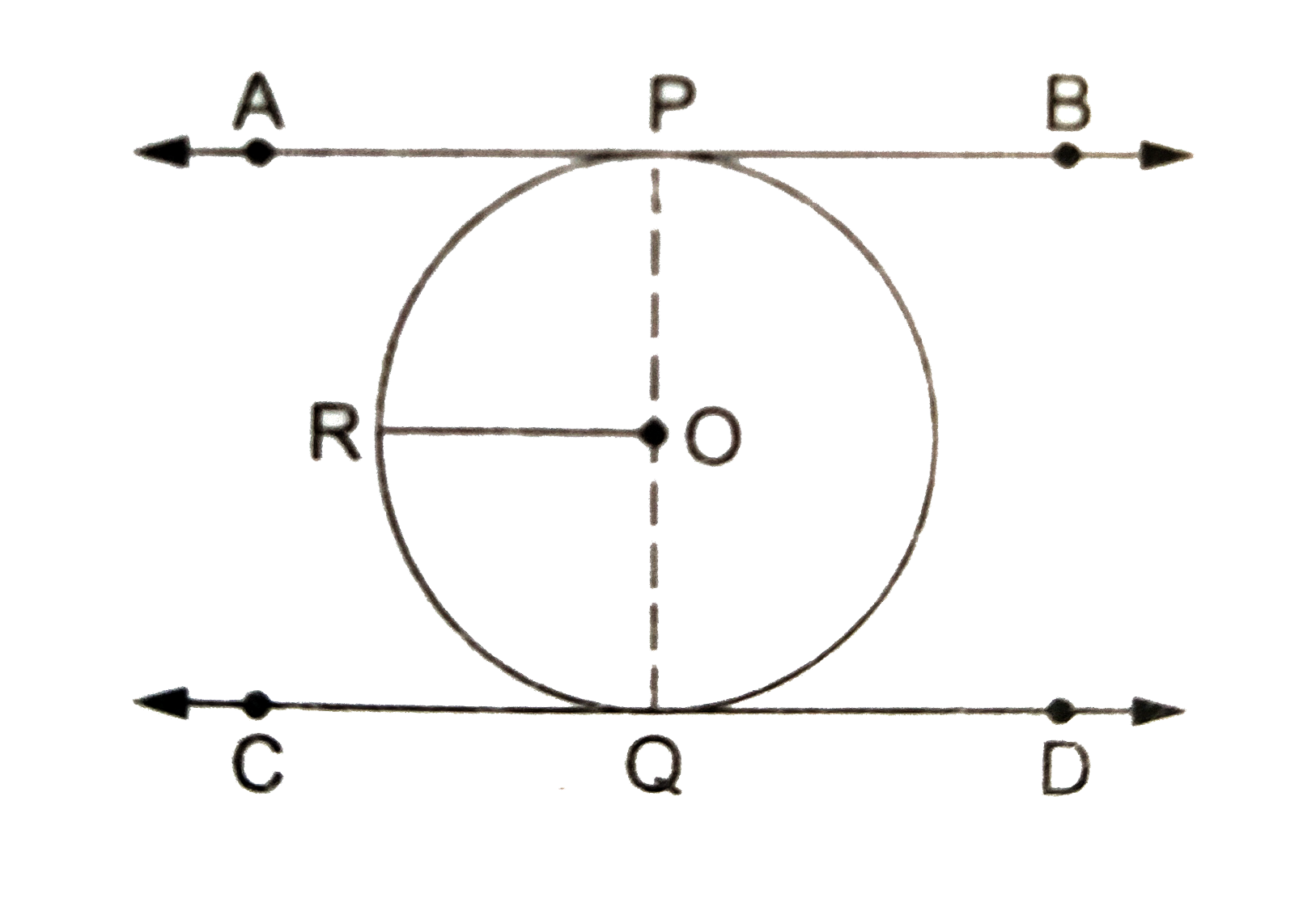
Prove that the line segment joining the points of contact of two parallel tangents of a circle, passes through its centre.

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

Let A P B and C Q D be two parallel tangents to a circle with centre O .
Join O P and O Q . Draw R O ∣ ∣ A B .
Now, ∠ A P O + ∠ R O P = 180 ∘ [ co-interior angles]
⇒ ∠ R O P = 90 ∘ [ ∵ ∠ A O P = 90 ∘ ]
Similarly , ∠ C Q O + ∠ R O Q = 180 ∘ [co-interiror angles]
⇒ ∠ R O Q = 90 ∘ [ ∵ ∠ C Q O = 90 ∘ ]
∴ ∠ P O Q = ∠ R O P + ∠ R O Q = 180 ∘ .
Hence, P O Q is a straight line passing through O
ocr_image

Updated on:21/07/2023
|
Class 10MATHSCIRCLES

Topper's Solved these Questions

Similar Questions