Number of integral points (integral points means both the co-ordinates should be integer) exactly in the interior of the triangle with vertices (0,0),(0,15)andOr(15,0) is
133
190
233
105
The correct Answer is:B
Clearly ,
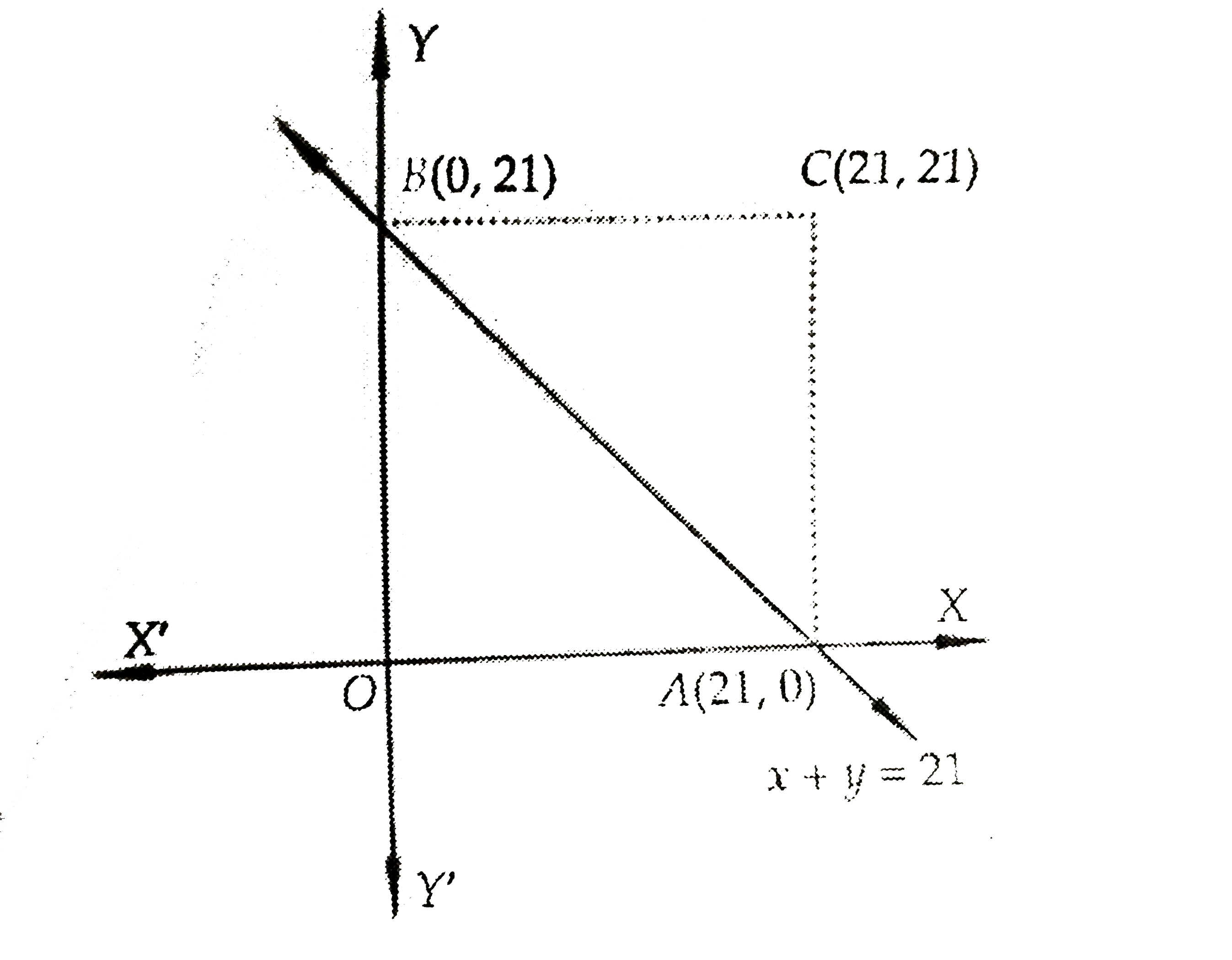
Required number of integral points
= 12 (Number of integral points in the interior of square OACB - Number of integral points on line AB)
Now , Number of integral points on AB
= Number of positive integral solutions of x + y = 21
=21−1C2−1=20C1=20
Hence , required number of points = 12(20×20−20)=190
ALTER Coordinates of integral points inside the triangle OAB are :
(1,1),(2,1)...,(1,18),(1,19)
(2,1) , (2,2) ..., (2,18)
(3,1) , (3,2) ..., (3,17)
: :
(17 , 1) , (17, 2) , (17 , 3)
(18 , 1) , (18,2)
(19,1)
∴ Total number of integral points
=19+18+17+...+2+1=19×202=190
Topper's Solved these Questions
- STRAIGHT LINESBOOK - OBJECTIVE RD SHARMACHAPTER - STRAIGHT LINESEXERCISE - Section-1 Solved MCQs (Example)
1 Video
- STRAIGHT LINESBOOK - OBJECTIVE RD SHARMACHAPTER - STRAIGHT LINESEXERCISE - SECTION - II (ASSERTION - REASON TYPE MCQs)
14 Videos
- STRAIGHT LINESBOOK - OBJECTIVE RD SHARMACHAPTER - STRAIGHT LINESEXERCISE - ILLUSTRATION 18
1 Video
- SETSBOOK - OBJECTIVE RD SHARMACHAPTER - SETSEXERCISE - Chapter Test
30 Videos
Similar Questions
The number of points, having both co-ordinates as integers, that lie in the interior of the triangle with vertices (0,0), (0,41) and (41,0) is (1) 901 (2) 861 (3) 820 (4) 780.
View SolutionThe number of points, having both co-ordinates as integers, that lie in the interior of the triangle with vertices (0, 0), (0, 41) and (41, 0), is: (4) 780 (3) 820 (2) 861 (1) 901
View Solution
Knowledge Check
The number of integral points (integral point means both the coordinates should be integer) exactly in the interior of the triangle with vertices (0,0), (0,21) and (21,0) is
A133B190C233D105The number of points, having both co-ordinates as integers, that line in the interior of the triangle with vertices (0, 0), (0, 41) and (41,0), is :
A901B861C820D780The number of points, having both coordinates are integers, that lie in the interior of the triangle with vertices (0,0), (0,41) and (41, 0) is
A901B861C820D780
Using integration, find the area of the triangle whose vertices are (1, 0), (4, 0) and (4, 4).
View SolutionUsing integration, find the area of the triangle whose vertices are (−1,0)(1,3) and (3, 2).
View SolutionUsing integration, find the area of the triangle ABC whose vertices are A(−1,1),B(0,5) and C(3,2).
View SolutionThe nine point centre of the triangle with vertices (1,√3),(0,0) and (2, 0) is
View SolutionThe number of integral points that lie exactly in the interior of the triangle with vertices O (0,0) A (21,0) B (0,21) is
View Solution