For a point $P$ in the plane, let $d_{1} (P) a n d d_{2} (P)$ be the distances of the point $P$ from the lines $x - y = 0 a n d x + y = 0$ respectively. The area of the region $R$ consisting of all points $P$ lying in the first quadrant of the plane and satisfying $2 \leq d_{1} (P) + d_{2} (P) \leq 4,$ is

A

4 sq.units

B

6 units

C

8 sq. units

D

2 sq. units

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

Let P $(α, β)$ be a point in the first quadrant . Then two cases arise .
CASE I When P lies in the region satisfying $y \leq x$ :
In this case , $β \leq α$
So , $d_{1} (P) + d_{2} (P) = \frac{| α - β |}{\sqrt{2}} + \frac{| α + β |}{\sqrt{2}}$
= $\frac{α - β}{\sqrt{2}} + \frac{α + β}{\sqrt{2}} = \frac{2 α}{\sqrt{2}} = \sqrt{2} α$
$∴ 2 \leq d_{1} (P) + d_{2} (P) \leq 4 \Rightarrow 2 \leq \sqrt{2} α \leq 4 \Rightarrow \sqrt{2} \leq α \leq 2 \sqrt{2} ... (i)$

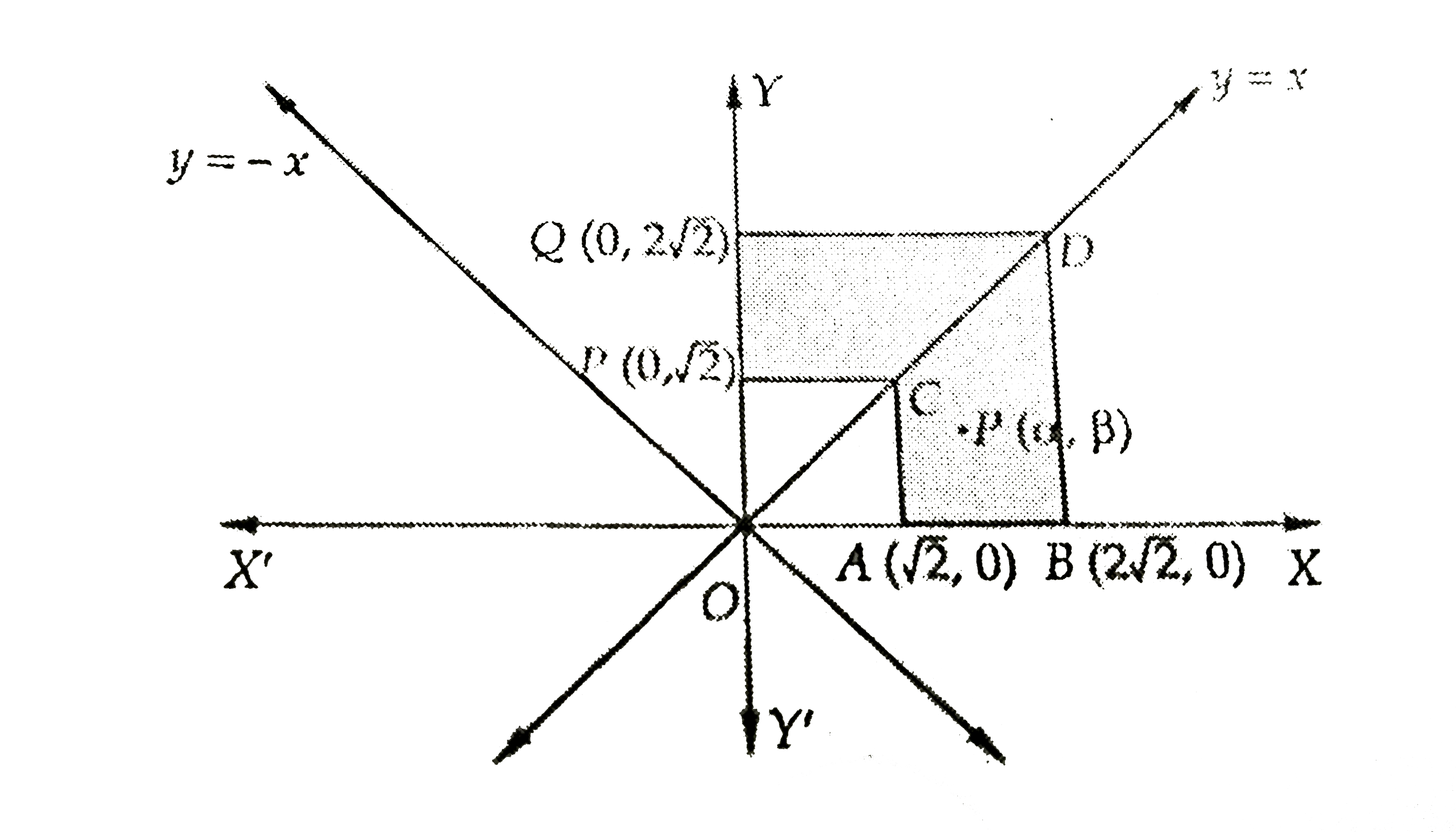
CASE II When P lies in the region satisfying $y \geq x$ :
In this case , we have $β \geq α$ .
So , $d_{1} (P) + d_{2} (P) = \frac{| α - β |}{\sqrt{2}} + \frac{| α + β |}{\sqrt{2}} = \frac{β - α}{\sqrt{2}} + \frac{α + β}{\sqrt{2}} = \sqrt{2} β$
$∴ 2 \leq d_{1} (P) + d_{2} (P) \leq 4 \Rightarrow 2 \leq \sqrt{2} β \leq 4 \Rightarrow \sqrt{2} \leq β \leq 2 \sqrt{2}$ ... (ii)
The shaded region shown in fig satisfies (i) and (ii) . So , region R is the shaded region in Fig.
Area of region R = Area of square OBDQ - Area of square OACP
$= {(2 \sqrt{2})}^{2} - {(\sqrt{2})}^{2} = 6$ sq. units
Hence , the locus of Q is ${(x - 1)}^{2} + {(y - 2)}^{2} = {(\sqrt{2})}^{2}$ which is a circle of radius $\sqrt{2}$ .

Updated on:21/07/2023

|

Class 11 MATHS STRAIGHT LINES

Topper's Solved these Questions

Similar Questions

Knowledge Check

Let P be the set of points $(x, y)$ such that $x^{2} \leq y \leq - 2 x + 3$ . Then area of region bounded by points in set P is
A $\frac{16}{3}$
B $\frac{32}{3}$
C $\frac{29}{3}$
D $\frac{20}{3}$

OBJECTIVE RD SHARMA-STRAIGHT LINES-Section I - Solved Mcqs

Home
Ask Doubt
Profile