If a vertex of an equilateral triangle is the origin and the side opposite to it has the equation x+y=1, then orthocentre of the triangle is :
(1/3 , 1/3)
(√2/3,√2/3)
(2/3 , 2/3)
none of these
Let OAB be the equilateral triangle . In an equilateral triangle the orthocentre and centroid coincide . So , the orthocentre H divides OL in the ratio 2:1.
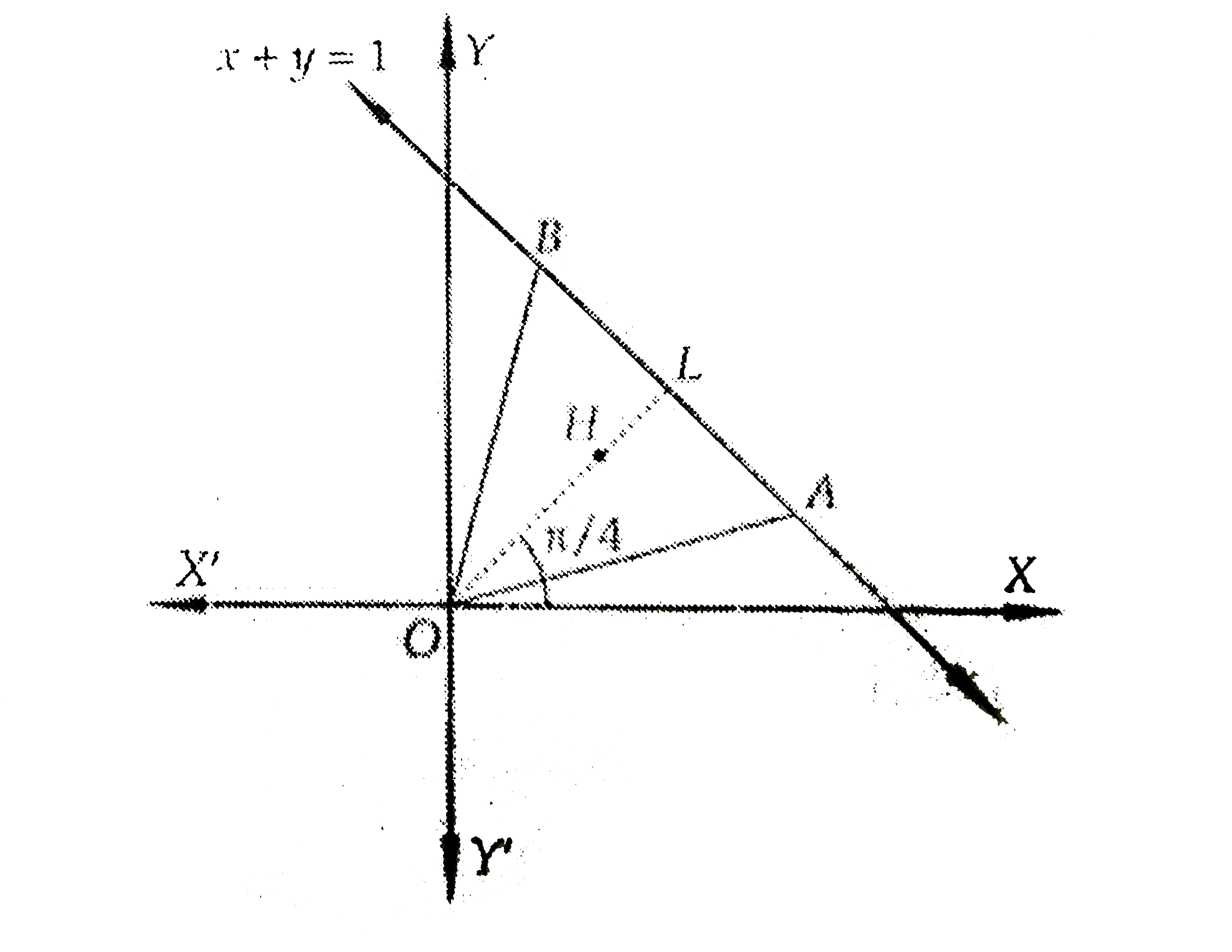
We have ,
OL = Length of the ⊥ from (0,0) on x + y - 1 = 0
⇒OL=1√2
∴OH=23×OL=√23
So , the coordinates of H are
bot(√23cos(π)4,√23sinπ4)=(1/3,1/3)
Topper's Solved these Questions
- STRAIGHT LINESBook:OBJECTIVE RD SHARMAChapter:STRAIGHT LINESExercise:Section-1 Solved MCQs (Example)
Explore 1 Video
- STRAIGHT LINESBook:OBJECTIVE RD SHARMAChapter:STRAIGHT LINESExercise:SECTION - II (ASSERTION - REASON TYPE MCQs)
Explore 14 Videos
- STRAIGHT LINESBook:OBJECTIVE RD SHARMAChapter:STRAIGHT LINESExercise:ILLUSTRATION 18
Explore 1 Video
- SETSBook:OBJECTIVE RD SHARMAChapter:SETSExercise:Chapter Test
Explore 30 Videos
Similar Questions
Knowledge Check
A vertex of an equateral triangle is (2,3) and the opposite side is x + y =2. The equaiton of other sides are
A(2±√3)x−y=1±2√3B(2±√3)x+y=1±2√3C(1±2√3)x−y=2±√3D(2±√3)x+y=1±2√3If one vertex of an equilateral triangle of side a lies at the oringin an d the other lies on the line x−√3y=0, the co ordinates of the third vertex are
A(0,a)B(√3a/2,−a/2)C(0,−a)D(−√3a/2,a/2)One vertex of the equilateral triangle with centroid at the origin and one side as x+y−2=0 is
A(-1,-1)B(2,2)C(-2,2)DNone of these
If one vertex of an equilateral triangle of side 'a' lie at the origin and the other lies on the line x−√3y=0, the co-ordinates of the third vertex are:
View SolutionIf the incentre of an equilateral triangle is (1,1) and the equation of its one side is 3x+4y+3=0 then the equation of the circumcircle of the triangle is
View SolutionOne vertex of an equilateral triangle is (2,3) and the equation of one side is x−y+5=0. Then the equations to other sides are
View SolutionOne vertex of the equilateral triangle with centroid at the origin and one side as x+y−2=0 is :
View SolutionLet A (3,2) and B (5,1). ABP is an equilateral triangle is constructed one the side of AB remote from the origin then the orthocentre of triangle ABP is:
View Solution