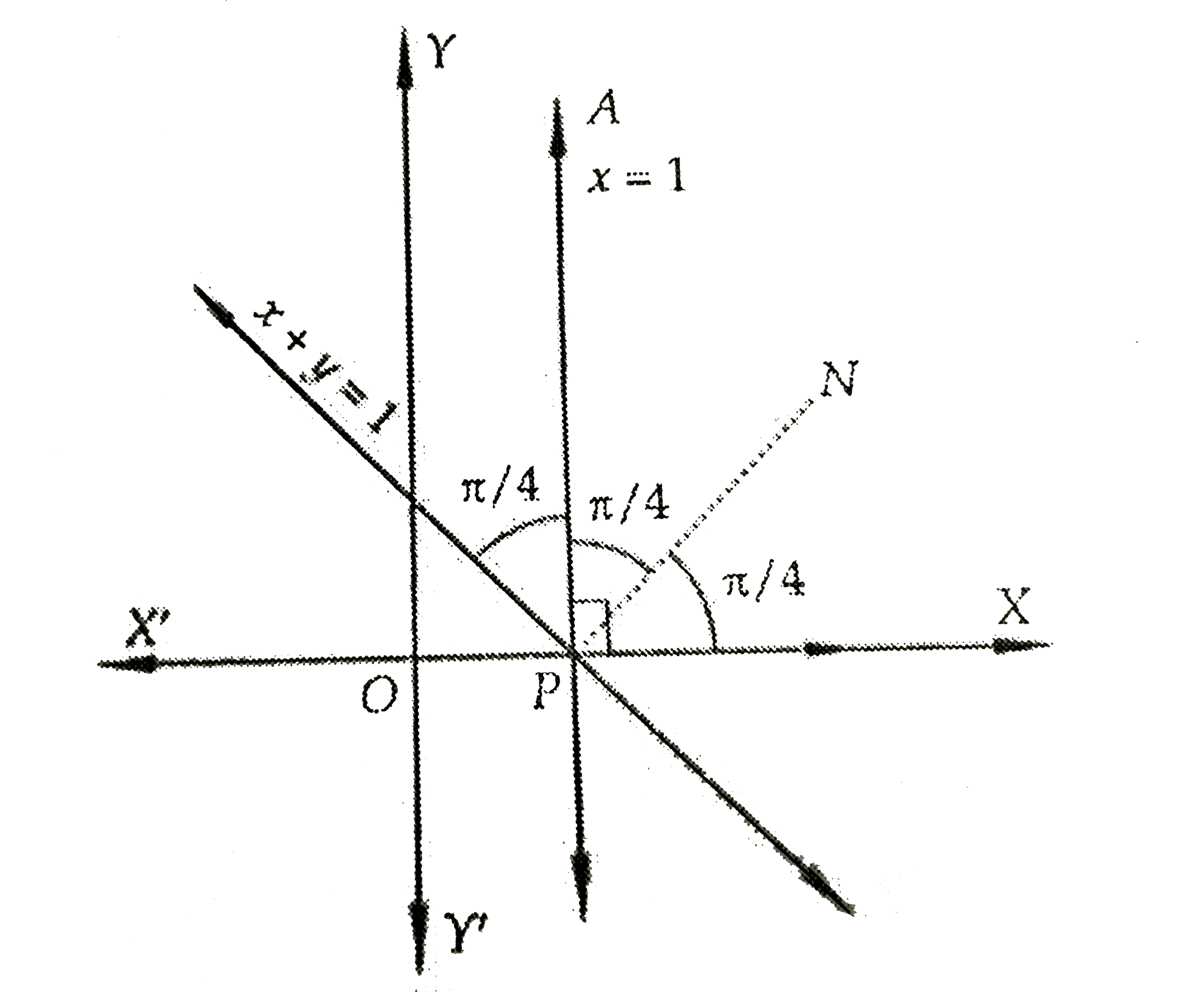
If a ray travelling along the line x = 1 gets reflected from the line

A ray travelling along the line 3x - 4y = 5 after being reflected from a line l travels along the line 5x + 12y = 13. Then, the equation of the line l is
Ax+8y= 0
Bx=8y
CX + 4y = 65
D32x - 4y = -65
A ray of light is sent along the line $x - 2 y - 3 = 0$ on reaching the line $3 x - 2 y - 5 = 0$ the ray is reflected from it, then the equation of the line containing the reflected ray is
A(a) 2x-29y-30=0
B(b) 29x-2y-31=0
C(c)3x-39y+37=0
D(d)31x-3y+37=0
A ray of light coming along the line 3x + 4y - 5 = 0 gets reflected from the line ax + by - 1= 0 and goes along the line 5x - 12y - 10 = 0, then
A $a = \frac{64}{115}, b = \frac{112}{15}$
B $a = - \frac{64}{115}, b = \frac{8}{15}$
C $a = \frac{64}{115}, b = - \frac{8}{15}$
D $a = - \frac{64}{115}, b = - \frac{8}{15}$

If a ray travelling along the line $x = 1$ gets reflected from the line x + y = 1, then the eqaution the line along which the reflected ray travels is