Question

${\displaystyle A(3,4),B(0,0)\text{and}c(3,0)}$ are vertices of ${\displaystyle \Delta ABC.}$ If 'P' is the point inside the ${\displaystyle \Delta ABC}$, such that ${\displaystyle d(P,BC)\le }$ min. ${\displaystyle \{d(P,AB),d(P,AC)\}}$. Then the maximum of ${\displaystyle d(P,BC)}$ is.(where ${\displaystyle d(P,BC)}$ represent distance between ${\displaystyle P\text{and}BC)}$.

Options

A. 1
B. `1//2`
C. 2
D. none of these

Explanation

We have ,
d (P , BC) `le` min {d (P, AB) , d(P, AC)}
`implies` d (P , BC) `le` d (P , AB) and d (P, BC) `le` d(P , AC)
Thus , d (P , BC) is maximum when
d (P , BC ) = d (P, AB) = d(P , AC)
`implies ` P coincides with the incentre of `Delta ABC`.

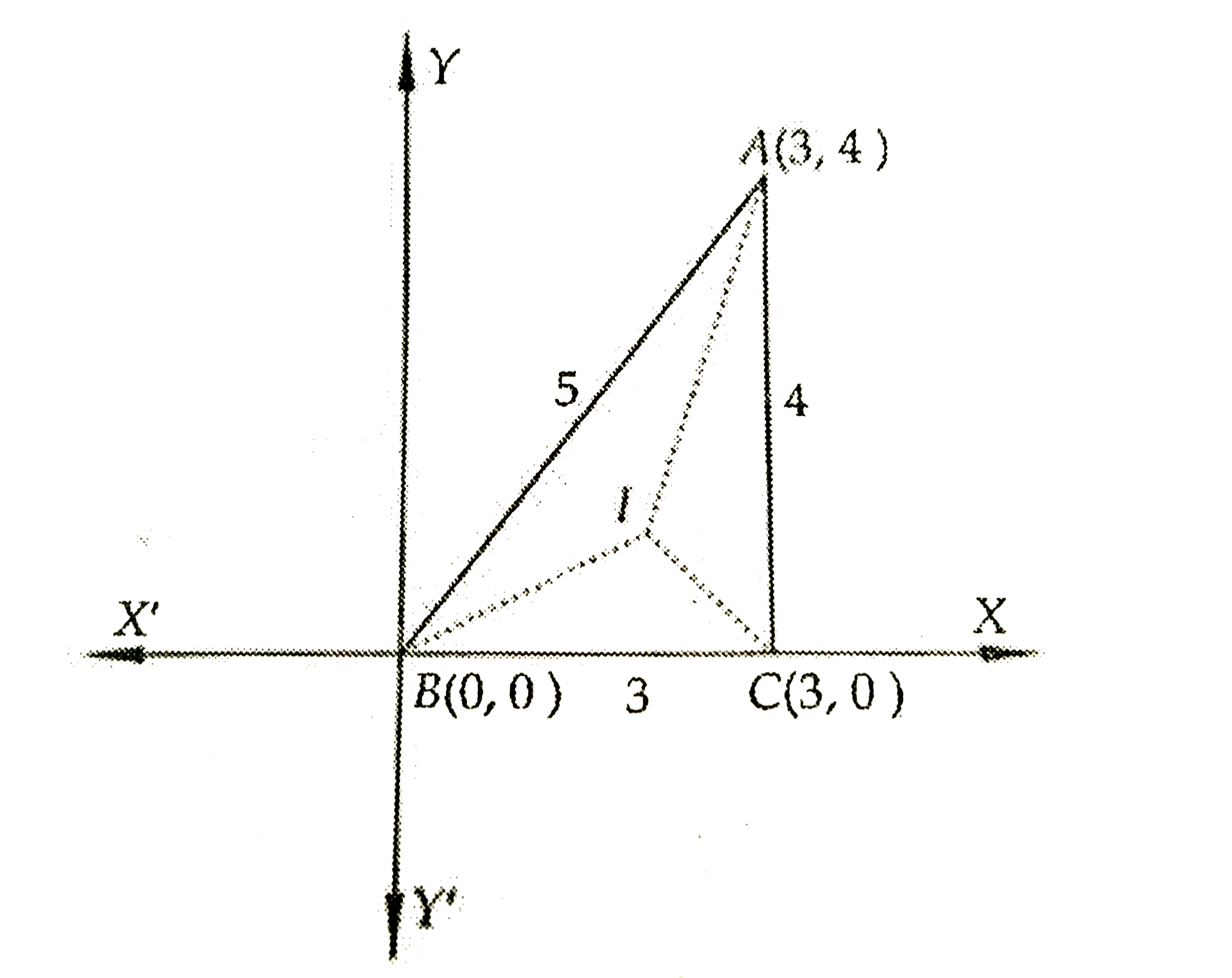
`therefore` Maximum value of d (P , BC)
= y-coordinate of the incentre of `Delta ABC`
`= (3 xx 4 + 4 xx 0 + 5 xx 0)/(3 + 4 + 5) = 1`