A right angled isosceles triangle is inscribed in the circle x2+y2_4x−2y−4=0 then length of its side is
A
3√2
B
2√2
C
√2
D
4√2
Video Solution
Text Solution
The correct Answer is:1
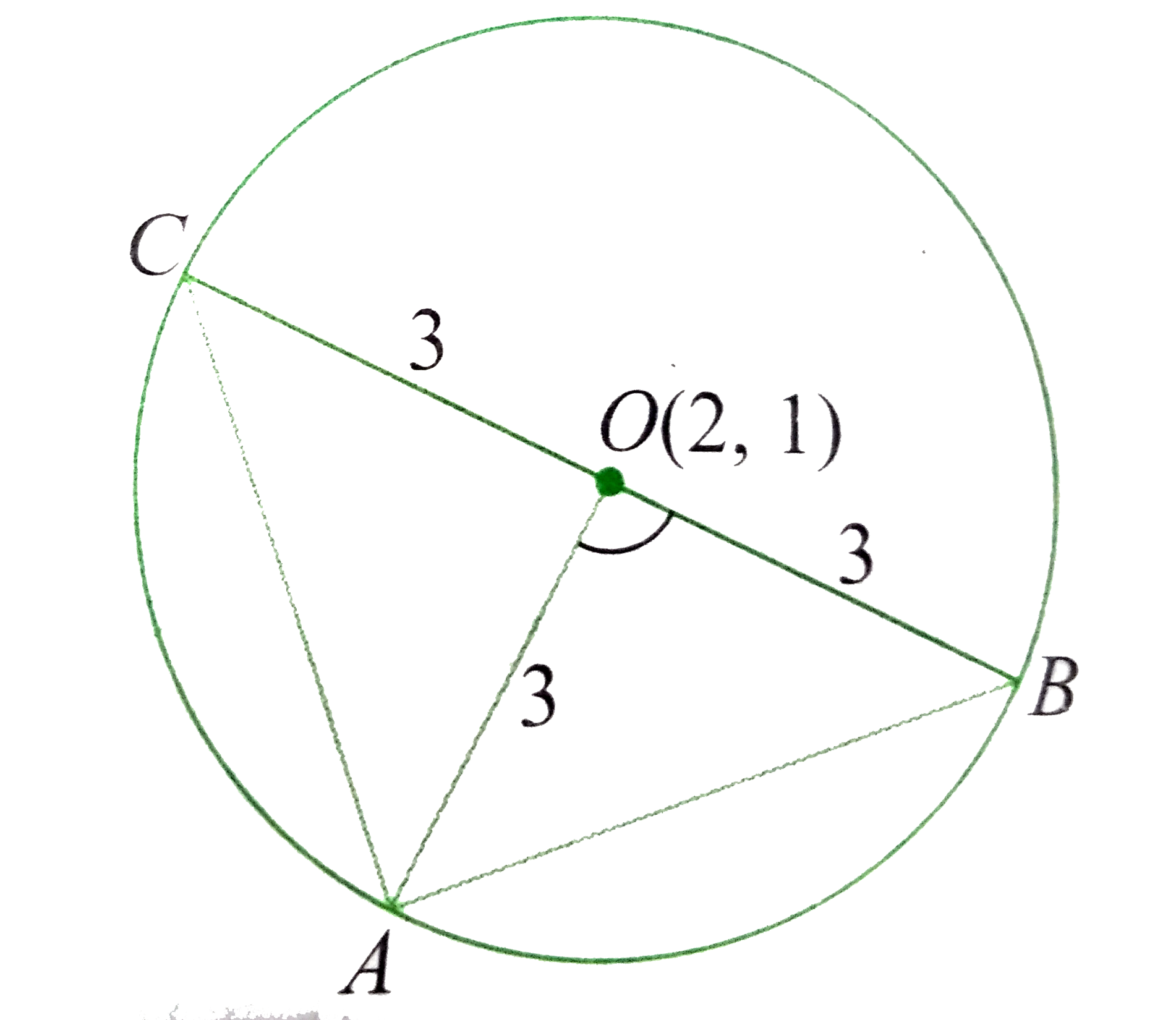
From the figure length of side to triangle is 3√2
|
Topper's Solved these Questions
- CIRCLE Book:CENGAGEChapter:CIRCLE Exercise:Exercise (Multiple)
Explore 25 Videos
- CIRCLE Book:CENGAGEChapter:CIRCLE Exercise:Exercise (Comprehension)
Explore 46 Videos
- CIRCLE Book:CENGAGEChapter:CIRCLE Exercise:Exercise 4.6
Explore 5 Videos
- BINOMIAL THEORMBook:CENGAGEChapter:BINOMIAL THEORMExercise:Question Bank
Explore 31 Videos
- CIRCLESBook:CENGAGEChapter:CIRCLESExercise:Question Bank
Explore 32 Videos
Similar Questions
Knowledge Check
If a square is inscribed in the circle x2+y2+2gx+2fy+c=0
then the length of each side of the square isA2rB4rC√2rDnoneAn isosceles triangle is inscribed in the circle x2+y2−6x−8y=0 with vertex at the origin and one of the equal sides along the axis of x. Equation of the other side through the origin is
A7x−24y=0B24x−7y=0C7x+24=0D24x+7y=0Area of the equilateral triangle inscribed in the circle x2+y2−7x9y+5=0 is
A1558√3squnitsB1658√3squnitsC1758√3squnitsD1858√3squnits
Area of the equilateral △ inscribed in the circle x2+y2−7x+9y+5=0, is …………
View SolutionThe area of an equilateral triangle inscribed in the circle x2+y2−2x=0 is
View SolutionFind the area of an equilateral triangle inscribed in the circle x2+y2−6x+2y−28=0
View SolutionIf a square with length of each side equal to a is inscribed in the circle x2+y2+4x+10y+21=0, then a is equal to
View SolutionAn equilateral triangle is inscribed in the circle x2+y2=1 which one vertex at the point (1,0). Length of each side of the tringle is
View Solution
