From a point R(5, 8) two tangents RP and RQ are drawn to a given circle s=0 whose radius is 5. If circumcentre of the triangle PQR is (2, 3), then the equation of circle S = 0 is
x2+y2+2x+4y−20=0
x2+y2+x+2y−10=0
x2+y2−x−2y−20=0
x2+y2−4x−6y−12=0
The correct Answer is:1
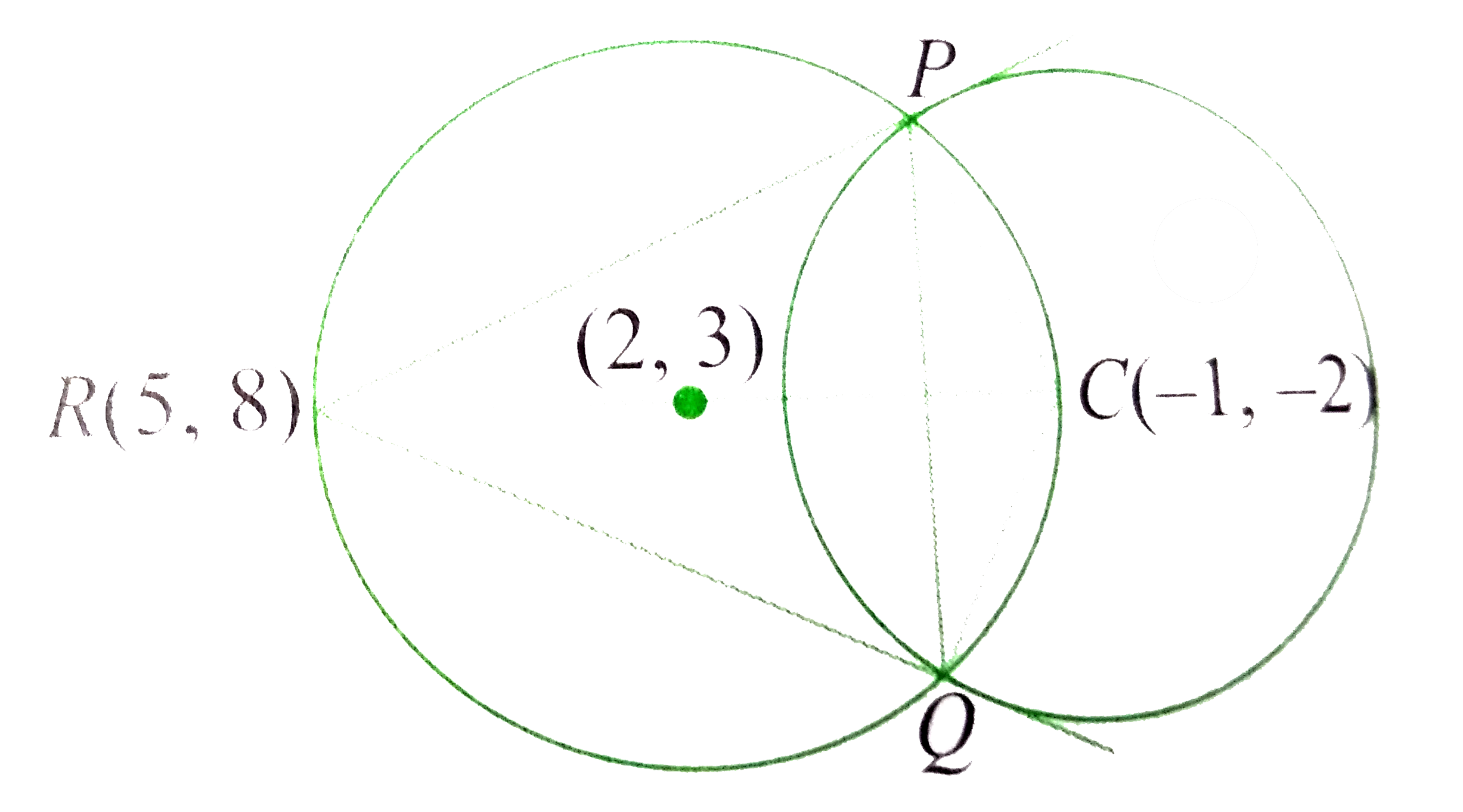
Let C be the center of the given circle.
Then, the circumcircle of ΔRPQ passes through C.
Therefore, (2,3) is the midpoint of RC.
Thus, the coordinates of C are (−1,−2).
Hence, the equation of the circle is x2+y2+2x+4y−20=0
Topper's Solved these Questions
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise (Multiple)
25 Videos
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise (Comprehension)
46 Videos
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise 4.6
5 Videos
- BINOMIAL THEORMBOOK - CENGAGECHAPTER - BINOMIAL THEORMEXERCISE - Question Bank
31 Videos
- CIRCLESBOOK - CENGAGECHAPTER - CIRCLESEXERCISE - Question Bank
32 Videos
Similar Questions
From a point R(5,8) , two tangents RPandRQ are drawn to a given circle S=0 whose radius is 5. If the circumcenter of triangle PQR is (2, 3), then the equation of the circle S=0 is x2+y2+2x+4y−20=0 x2+y2+x+2y−10=0 x2+y2−x+2y−20=0 x2+y2+4x−6y−12=0
View SolutionFrom a point A(−3,5) tangents are drawn to the circle. If BC is the contact, then the circumcentre of triangle ABC is
View Solution
Knowledge Check
From a point P perpendicular tangents PQ and PR are drawn to ellipse x2+4y2=4, then locus of circumcentre of triangle PQR is
Ax2+y2=165(x2+4y2)2Bx2+y2=516(x2+4y2)2Cx2+4y2=165(x2+y2)2Dx2+4y2=165(x2+y2)2The length of the tangent drawn to a circle of radius 4 cm from a point 5 cm away from the centre of the circle is
A3cmB4√2 cmC5√2cmD3√2 cmIf line 3x+y=0 be a tangent to a circle drawn from origin to a circle centred at the point (2,- 1) then the equation of other tangent through the origin is
Ax−3y=0Bx+2y=0Cx+3y=0D3x−y=0
Two tangents OP & OQ are drawn from origin to the circle x2+y2−x+2y+1=0 then the circumcentre of ΔOPQ is
View SolutionLength of a tangent drawn to a circle with radius 3 cm from a point 4 cm from the centre of the circle is
View SolutionFind the length of tangent segment drawn to a circle with radius 5 cm from a point 13 cm from the centre of the circle.
View SolutionFind the length of a tangent drawn to a circle with radius 5 cm, from a point 13 cm from the centre of the circle.
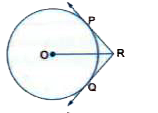
View SolutionIn Fig. two tangents RQ and RP are drawn from an external point R to the circle with centre O. If ∠PRQ=120∘ , then prove that OR = PR + RQ .
View Solution
