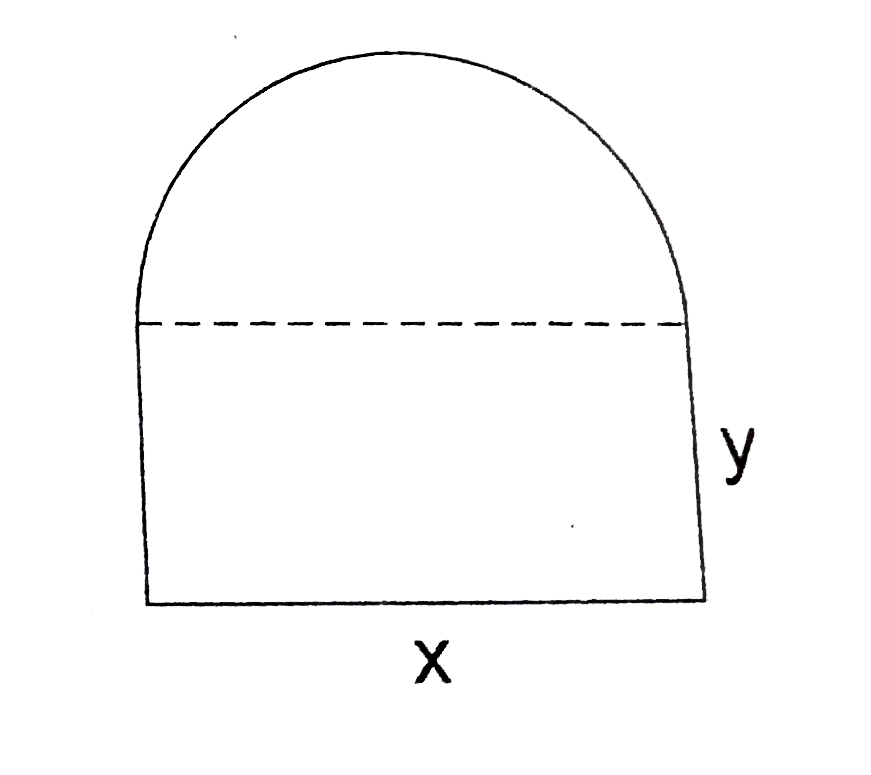
A window is in the form of a rectangle surmounted by a semi-circular opening. The total perimeter of the window is 10 meters. Find the dimensions of the rectangle so as to admit maximum light through the whole opening.

Video Solution

Text Solution

Verified by Experts

The correct Answer is:breadth $= \frac{20}{(π + 4)} m$ , height $= \frac{10}{(π + 4)} m$

Let the length and breadth of the rectangle be x and y metres respectively. Then, radius of the semicircle $= (x / 2)$ metres
So, the perimeter of the window is given by
$10 = x + 2 y + \frac{π x}{2} \Rightarrow y = 5 - (\frac{2 + π}{4}) x$
$∴ A = x y + \frac{1}{2} π {(\frac{x}{2})}^{2} \Rightarrow A = x [5 - \frac{(2 + π)}{4} . x] + \frac{π x^{2}}{8}$
$∴ \frac{d A}{d x} = (5 - x - \frac{π x}{4}) and \frac{d^{2} A}{{d x}^{2}} = - (1 + \frac{π}{4}) < 0$
Now, $\frac{d A}{d x} = 0 \Rightarrow x = \frac{20}{(π + 4)}$ .
$∴$ A is maximum when $x = \frac{20}{(π + 4)} and y = \frac{10}{(π + 4)}$

Updated on:21/07/2023

|

Class 12 MATHS APPLICATION OF DERIVATIVES

Topper's Solved these Questions

Similar Questions

RS AGGARWAL-APPLICATIONS OF DERIVATIVES-Exercise 11F

Home
Ask Doubt
Profile