Prove that the perimeter of a right-angled triangle of given hypotenuse is maximum when the triangle is isosceles

Video Solution

Text Solution

Verified by Experts

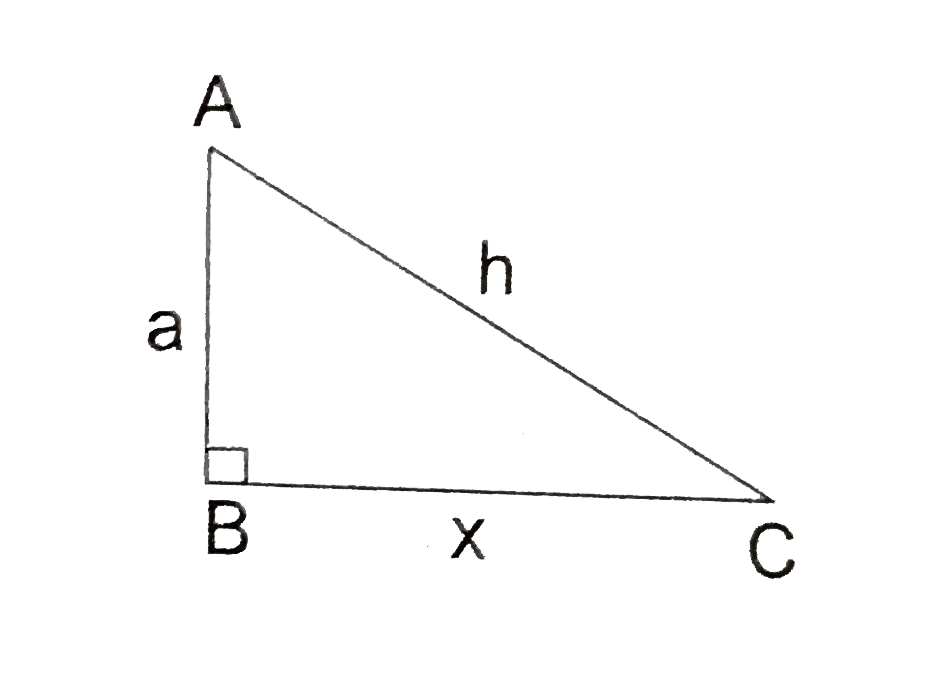
Let ABC be the right triangle with given hypotenuse h
Let, base BC = x and altitude $B A = a$
Then, $h^{2} = x^{2} + a^{2} \Rightarrow a = \sqrt{h^{2} - x^{2}}$
$∴ P = a + x + h \Rightarrow P = \sqrt{(h^{2} - x^{2})} + x + h$
So, $\frac{d P}{d x} = \frac{- x}{\sqrt{h^{2} - x^{2}}} + 1 and \frac{d^{2} P}{{d x}^{2}} = \frac{- h^{2}}{{(h^{2} - x^{2})}^{3 / 2}}$
Now, $\frac{d P}{d x} = 0 \Rightarrow x = \frac{h}{\sqrt{2}}$
And for $x = \frac{h}{\sqrt{2}}, \frac{d^{2} P}{{d x}^{2}} = \frac{- 2^{3 / 2}}{h} < 0$
$∴$ P is maximum when $x = \frac{h}{\sqrt{2}} and a = \frac{h}{\sqrt{2}}$ i.e., when x = a

Updated on:21/07/2023