If the area (in sq. units) of the region ${(x, y) : y^{2} \leq 4 x, x + y \leq 1, x \geq 0, y \geq 0}$ is $a \sqrt{2} + b$ , then $a - b$ is equal to :

A

$\frac{10}{3}$

B

6

C

$\frac{8}{3}$

D

$- \frac{2}{3}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

Given region is $(x, y) : y^{2} \leq 4 x, x + y \leq 1, x \geq 0, y \geq 0}$

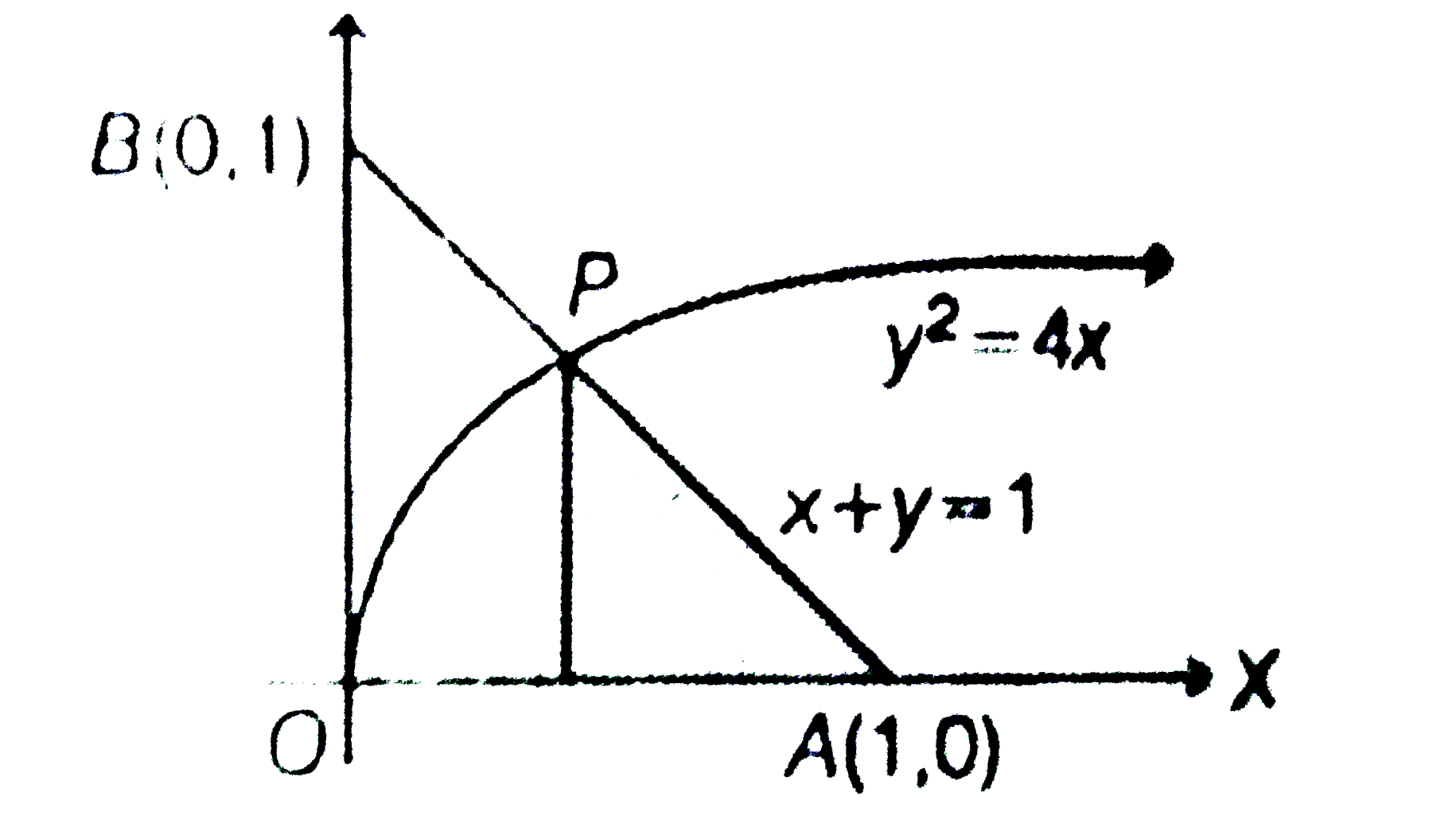
Now, for point P, put value of $y = 1 - x to y^{2} = 4 x,$ we get
${(1 - x)}^{2} = 4 x \Rightarrow x^{2} + 1 - 2 x = 4 x$
$\Rightarrow x^{2} - 6 x + 1 = 0$
$\Rightarrow x = \frac{6 \pm \sqrt{36 - 4}}{2}$
$= 3 \pm 2 \sqrt{2}$ .
Since., x-coordinate of P less than x-coordinate of point A(1, 0).
$∴ x = 3 - 2 \sqrt{2}$
Now, required area
$= \int_{0}^{3 - 2 \sqrt{2}} 2 \sqrt{2} d x + \int_{3 - 2 \sqrt{2}}^{1} (1 - x) d x$
$= 2 {∣ ∣ ∣ \frac{x^{3 / 2}}{3 / 2} ∣ ∣ ∣}_{0}^{3 - 2 \sqrt{2}} + {[x - \frac{x^{2}}{2}]}_{3 - 2 \sqrt{2}}^{1}$
$= \frac{4}{3} {(3 - 2 \sqrt{2})}^{3 / 2} + (1 - \frac{1}{2}) - (3 - 2 \sqrt{2}) + \frac{{(3 - 2 \sqrt{2})}^{2}}{2}$
$= \frac{4}{3} {[{(\sqrt{2} - 1)}^{2}]}^{3 / 2} + \frac{1}{2} - 3 + 2 \sqrt{2} + \frac{1}{2} (9 + 8 - 12 \sqrt{2})$
$= \frac{4}{3} {(\sqrt{2} - 1)}^{3} - \frac{5}{2} + 2 \sqrt{2} + \frac{17}{2} - 6 \sqrt{2}$
$= \frac{4}{3} (2 \sqrt{2} - 3 (2) + 3 (\sqrt{2}) - 1) - 4 \sqrt{2} + 6$
$= \frac{4}{3} (5 \sqrt{2} - 7) - 4 \sqrt{2} + 6 = \frac{8 \sqrt{2}}{3} - \frac{10}{3}$
$= a \sqrt{2} + b (given)$
So, on comparing $a = \frac{8}{3} and b = - \frac{10}{3}$
$∴ a - b = \frac{8}{3} + \frac{10}{3} = 6$

Updated on:21/07/2023

|

Class 12 MATHS APPLICATION OF INTEGRALS

Topper's Solved these Questions

Similar Questions

Knowledge Check

The area (in sq. units)of the region $A = {(x, y) : x^{2} \leq y \leq x + 2}$ is :
A $\frac{10}{3}$
B $\frac{9}{2}$
C $\frac{31}{6}$
D $\frac{13}{6}$
The area (in sq units) of the region $A = {(x, y) : x^{2} \leq y \leq x + 2}$ is
A $\frac{13}{6}$
B $\frac{9}{2}$
C $\frac{31}{6}$
D $\frac{10}{3}$
The area (in sq. units) of the region $A = {(x, y) : x^{2} \leq y \leq x + 2}$ is :
A $\frac{13}{6}$
B $\frac{9}{2}$
C $\frac{10}{2}$
D $\frac{31}{6}$

IIT JEE PREVIOUS YEAR-AREA-AREA USING INTEGRATION

Home
Ask Doubt
Profile