In a ΔABC, the median to the side BC is of length 1√11−6√3 and it divides the ∠A into angles 30∘ and 45∘. Find the length of the side BC.
The correct Answer is:2
Let AD be the median to the base BC of a of ΔABCandlet∠ADC=θ, then
(a2+b2)cotθ=a2cos30∘−a2cot45∘
⇒cotθ=√3−12
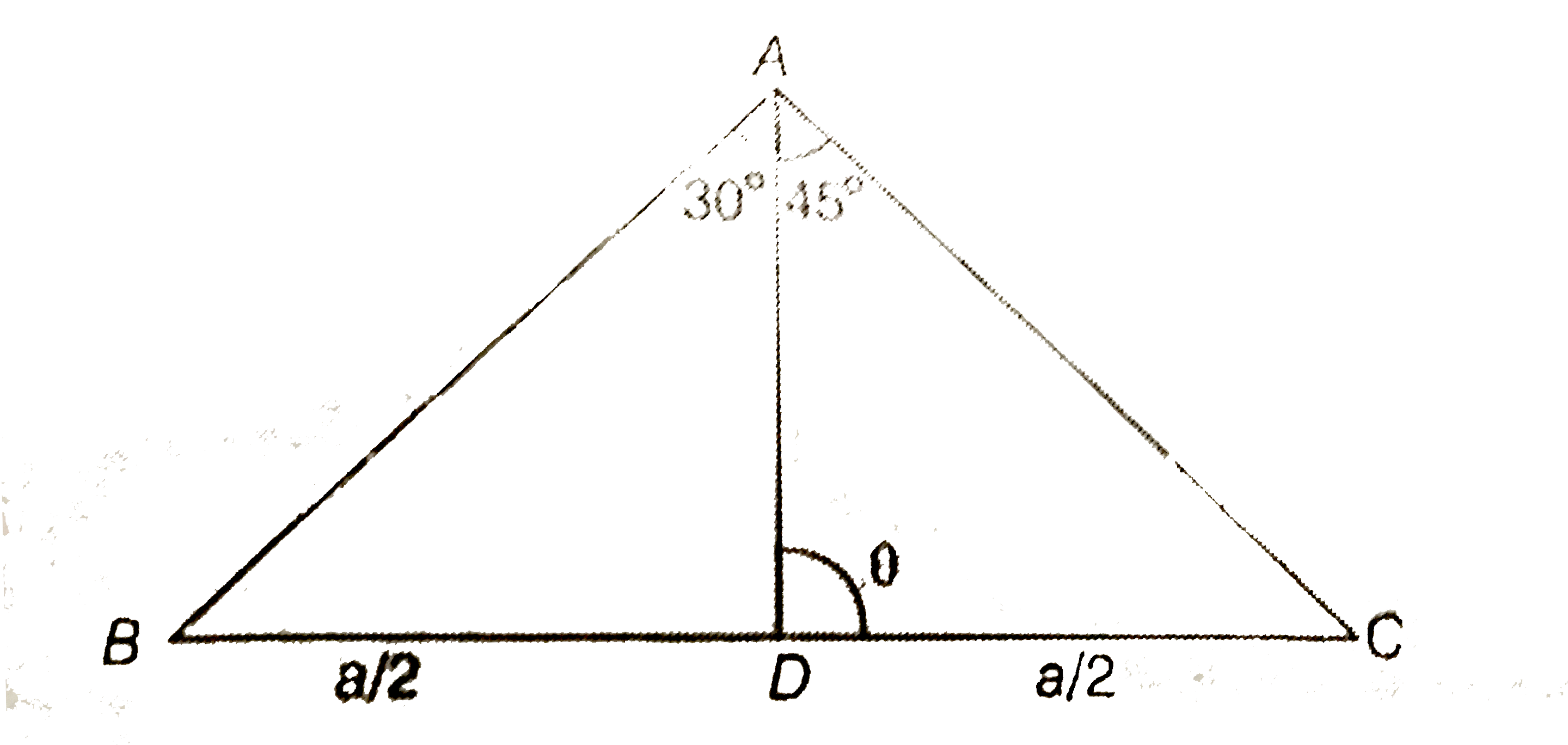
Applying sine rule in ΔABC, we get
ADsin(π−θ−45∘)=DCsin45∘
⇒ADsin(θ+45∘)a21√2
⇒AD=a√2(sin45∘cosθ+cos45∘sinθ)
⇒AD=a√2(cosθ+sinθ√2)=a2(cosθ+sinθ)
⇒1√11−6√3=a2(√3−1√8−2√3+2√8−2√3)
⇒a=2√8−2√3(√3+1)√11−6√3=2√8−2√3√(√3+1)2√11−6√3
⇒a=2√8−2√3(√4+2+√3)√11−6√3
⇒a=2√8−2√3(√44−24√3+22√3−36)
=2√8−2√3√8−2√3=2
Topper's Solved these Questions
- PROPERTIES OF TRIANGLESBOOK - IIT JEE PREVIOUS YEARCHAPTER - PROPERTIES OF TRIANGLESEXERCISE - APPLICATIONS OF AREA, NAPIERS ANALOGY AND SOLUTION OF A TRIANGLE
17 Videos
- PROPERTIES OF TRIANGLESBOOK - IIT JEE PREVIOUS YEARCHAPTER - PROPERTIES OF TRIANGLESEXERCISE - CIRCUMCIRCLE, INCIRCLE, ESCRIBED CIRCLE, ORTHOCENTRE,INCENTRE AND CIRCUMCENTRE
20 Videos
- 3D GeometryBOOK - IIT JEE PREVIOUS YEARCHAPTER - 3D GeometryEXERCISE - Equation of Plane Objective Question II (Integer Answer Type Question)
1 Video
Similar Questions
Knowledge Check
In a ΔABC if the length of the sides are √2,√6 and √8, then the measures of the angles are
A30∘,60∘,90∘B45∘,75∘,60∘C45∘,30∘,105∘Dnone of theseIn a !ABC the length of the median AD to the side BC is 4 units. If ∠A=60∘ and the area of the triangle is 2√3 sq. units. The length of side BC, is
A2√3B4√3C6D8
In ΔABC, angle A is 120∘,BC+CA=20,andAB+BC=21 Find the length of the side BC
View SolutionIn a ΔABC , Median AD perpendicular to side BC . Find the value of tanA/tanB
View SolutionTwo sides of a triangle are of lengths √6and4 and the angle opposite to smaller side is 30. How many such triangles are possible? Find the length of their third side and area.
View SolutionTwo sides of a triangle are of lengths √6and4 and the angle opposite to smaller side is 30. How many such triangles are possible? Find the length of their third side and area.
View Solutionthe length of side AB and side BC a scalene triangle ABC are 12cm and 8cm respectively. the value of angle C is 59. find the length of side AC
View SolutionIf the lengths of the sides BC, CA and AB of ΔABC are a,b, and c repspectively and AD is the bisector of ∠A then find the length of BD and DC.
View Solution
