Calculate the magnetic field at distance y from the centre of the axis of a disc of radius r and unifrom surface charge density σ, If the disc spins with angular velocity ω ?
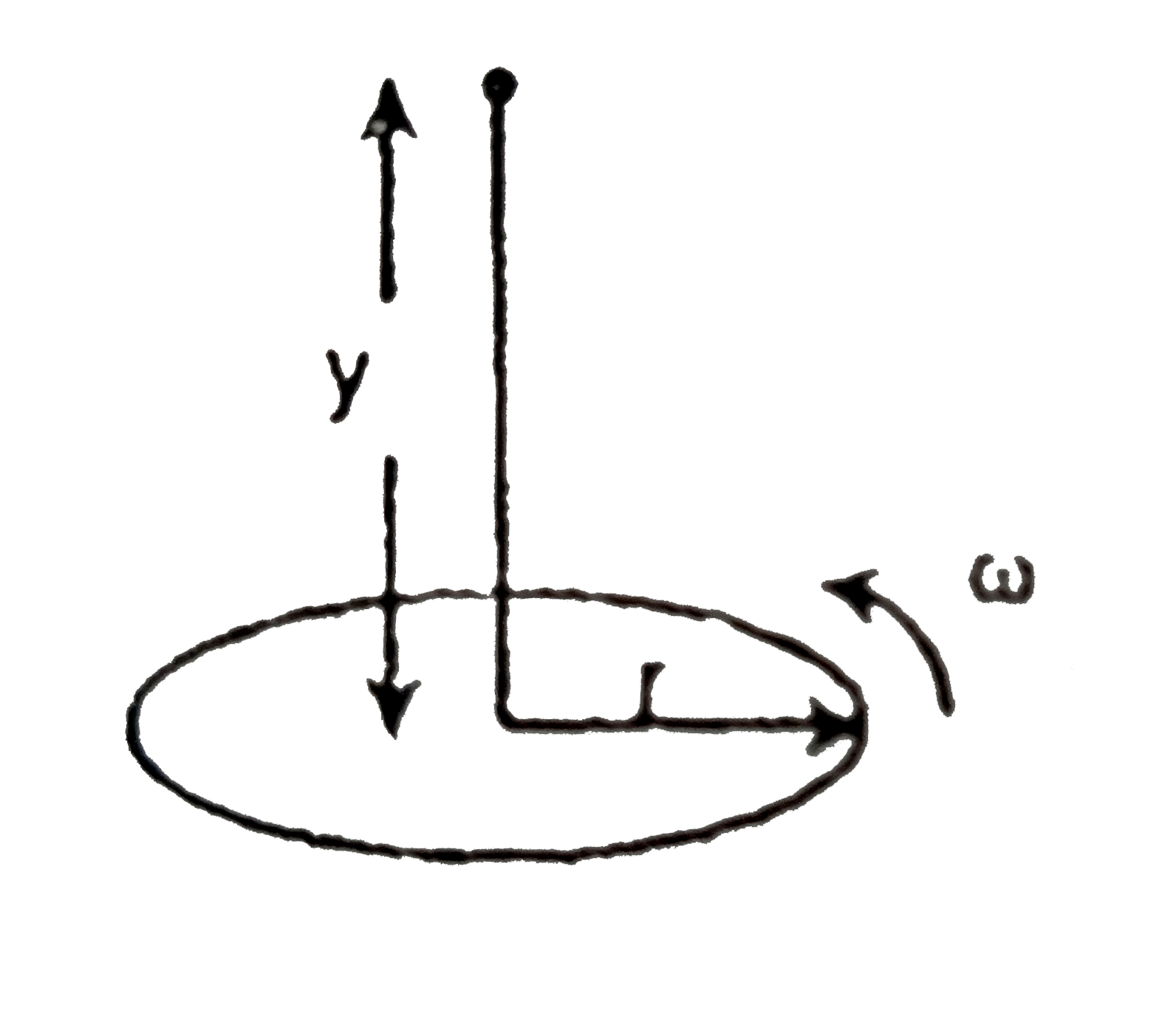
μ0σω3(r2−2y2√r2−y2+2y)
μ0σω2(r2+y2√r2+y2)
μ0σω2(r2+2y2√r2+y2−2y)
2μ0σω3(r2+2y2√r2+y2−2y)
The correct Answer is:C
Charge on a ring of radius x and width dx
dq=(2πxdx)σ
current, dI=dqdt=2πxσdxdt=σxdx
dB=μ0dIr22(X2+y2)3/2
B=μ0σω2(r2+2y2√r2+y2−2y)
Topper's Solved these Questions
- MAGNETICBook:FIITJEEChapter:MAGNETICExercise:Comprehension-1
Explore 3 Videos
- MAGNETICBook:FIITJEEChapter:MAGNETICExercise:Comprehension-2
Explore 2 Videos
- MAGNETICBook:FIITJEEChapter:MAGNETICExercise:Solved Problems (Subjective)
Explore 10 Videos
- LAWS OF MOTIONBook:FIITJEEChapter:LAWS OF MOTIONExercise:COMPREHENSION-III
Explore 2 Videos
- MAGNETISMBook:FIITJEEChapter:MAGNETISMExercise:Example
Explore 12 Videos
Similar Questions
Knowledge Check
A charge q is placed at the some distance along the axis of a uniformly charged disc of surface charge density σ. The flux due to the charge q through the disc is ϕ. The electric force on charge q exerted by the disc is
AσϕBσϕ4πCσϕ2πDσϕ3π
Find the potential φ at the edge of a thin disc of radius R carrying the uniformly distributed charge with surface density σ.
View SolutionCalculate electric field at a point on axis, which at a distance x from centre of uniformly charged disc having surface charge density σ and R which also contains a concentric hole of radius r.
View SolutionA disc of radius R rotates at an angular velocity ω about the axis perpendicular to its surface and passing through its centre. If the disc has a uniform surface charge density σ, find the magnetic induction on the axis of rotation at a distance x from the centre. (Given R=3m, x=4m and μ0σω=20Tesla/m.)
View SolutionA thin copper ring of radius a is charged with q units of electricity. Calculate the electric field at a point at a distance x from the centre and on the axis of the ring. Use the result to find the electirc field due to a charged disc of surface density of charge ε.
View SolutionFind the electric field caused by a disc of radius a with a uniform surfce charge density σ (charge per unit area) at a point along the axis of the disc a distance x from its centre.
View SolutionThe diagram shows a part of disc of radius R carrying uniformly distributed charge of density σ. Electric potential at the centre O of parent disc is
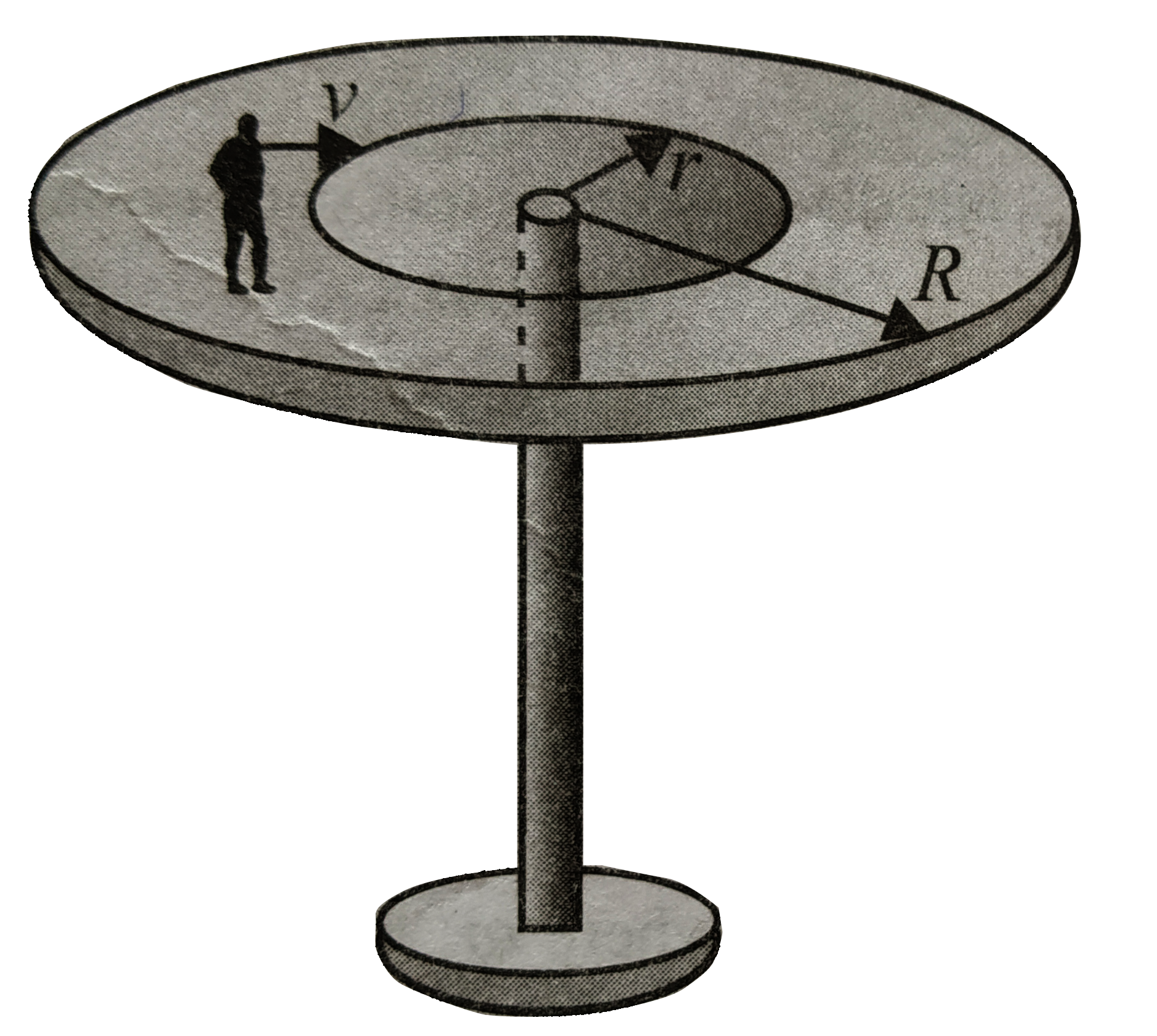
View SolutionA man of mass m stands on a horizontal platform in the shape of a disc of mass m and radius R, pivoted on a vertical axis through its centre about which it can freely rotate. The man starts to move around the centre of the disc in a circle of radius r with a velocity v relative to the disc. Calculate the angular velocity of the disc.
View Solution