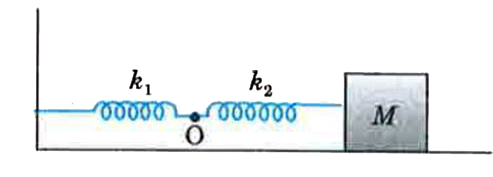
The figure shows a mass M attached to a series arrangement of two springs of spring constants $k_{1} and k_{2}$ where $k_{2} = 2 k_{1}$ . If the mass M oscillates in simple harmonic motion with amplitude A, the amplitude of the point O is $\frac{α A}{β}$ . Find $β - α$ .

Video Solution

Text Solution

Verified by Experts

The correct Answer is:1

Let F be the force by which mass M is stretched to the right and let A be the distance moved by it. When the mass is released, it executes S.H.M. of amplitude A. Let the extensions produced in the springs be $x_{1} and x_{2}$ in springs of spring constant $k_{1} and k_{2}$ , respectively.
So, $F = k_{1} x_{1} = k_{2} x_{2}$
$\Rightarrow x_{1} = \frac{F}{k_{1}} and x_{2} = \frac{F}{k_{2}}$
$\Rightarrow A = x_{1} + x_{2} = F (\frac{1}{k_{1}} + \frac{1}{k_{2}}) = F \frac{k_{1} + K_{2}}{k_{1} k_{2}}$
$∴ F = \frac{k_{1} k_{2} A}{(k_{1} + k_{2})}$
The amplitude of point O = amplitude of oscillation of spring $k_{1}$ which is $(k_{2} = 2 k_{1})$
$x_{1} = \frac{F}{k_{1}} = \frac{k_{2} A}{(k_{1} + k_{2})} = \frac{2 k_{1}}{3 k_{1}} A = \frac{2}{3} = \frac{α}{β} A$
$∴ β = 3$
$α = 2$
$\Rightarrow β - α = 1$

|

Updated on:21/07/2023

Class 11 PHYSICS SIMPLE HARMONIC MOTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Two springs of constants $k_{1}$ and $k_{2}$ have equal maximum velocities, when executing simple harmonic motion. The ratio of their amplitudes (masses are equal) will be
A $\frac{k_{1}}{k_{2}}$
B $\frac{k_{2}}{k_{1}}$
C ${(\frac{k_{1}}{k_{2}})}^{1 / 2}$
D ${(\frac{k_{2}}{k_{1}})}^{1 / 2}$
Question 2 - Select One
A mass m oscillates with simple harmonic motion with frequency $f = \frac{ω}{2 π}$ and amlitude A on a spring with constant K. therefore
Athe total energy of the system is $\frac{1}{2} K A^{2}$
BThe frequency is $\frac{1}{2 π} \sqrt{\frac{K}{M}}$
CThe maximum velocity occurs, when x=0
Dall the above are correct
Question 3 - Select One
Two particles A and B of equal masses are suspended from two massless springs of spring constants $k_{1}$ and $k_{2}$ , respectively, If the maximum velocities, during oscillation, are equal, the ratio of amplitude of A and B is
A $\sqrt{\frac{k_{1}}{k_{2}}}$
B $\frac{k_{2}}{k_{1}}$
C $\sqrt{\frac{k_{2}}{k_{1}}}$
D $\frac{k_{1}}{k_{2}}$

MODERN PUBLICATION-OSCILLATIONS -Competition (INTEGER TYPE QUESTIONS)

Home
Ask Doubt
Profile