If the diagonals of a quadrilateral are equal and bisect each other at right angles, then the quadrilateral is a
reactangle
square
rhombus
trapezium
The correct Answer is:B
To determine the type of quadrilateral where the diagonals are equal and bisect each other at right angles, we can analyze the properties of different quadrilaterals.
1. Understanding the Properties of Quadrilaterals:
- A quadrilateral is a four-sided polygon. The properties of its diagonals can help us identify its type.
2. Given Conditions:
- The diagonals of the quadrilateral are equal.
- The diagonals bisect each other at right angles (90 degrees).
3. Analyzing Each Option:
- Rectangle:
- In a rectangle, the diagonals are equal, but they do not bisect each other at right angles. Thus, a rectangle cannot be the answer.
- Rhombus:
- In a rhombus, the diagonals bisect each other at right angles, but they are not equal. Hence, a rhombus cannot be the answer.
- Trapezium:
- In a trapezium, the diagonals are not necessarily equal unless it is an isosceles trapezium, and even then, they do not bisect each other at right angles. Therefore, a trapezium cannot be the answer.
- Square:
- In a square, the diagonals are equal and they bisect each other at right angles. This satisfies both conditions given in the question.
4. Conclusion:
- Since the only quadrilateral that meets both conditions (equal diagonals and diagonals bisecting at right angles) is a square, we conclude that the quadrilateral is a square.
Final Answer:
The quadrilateral is a square.
---
- A quadrilateral is a four-sided polygon. The properties of its diagonals can help us identify its type.
- The diagonals of the quadrilateral are equal.
- The diagonals bisect each other at right angles (90 degrees).
- Rectangle:
- In a rectangle, the diagonals are equal, but they do not bisect each other at right angles. Thus, a rectangle cannot be the answer.
- Rhombus:
- In a rhombus, the diagonals bisect each other at right angles, but they are not equal. Hence, a rhombus cannot be the answer.
- Trapezium:
- In a trapezium, the diagonals are not necessarily equal unless it is an isosceles trapezium, and even then, they do not bisect each other at right angles. Therefore, a trapezium cannot be the answer.
- Square:
- In a square, the diagonals are equal and they bisect each other at right angles. This satisfies both conditions given in the question.
- Since the only quadrilateral that meets both conditions (equal diagonals and diagonals bisecting at right angles) is a square, we conclude that the quadrilateral is a square.
The quadrilateral is a square.
Topper's Solved these Questions
- GEOMETRYBOOK - ARIHANT SSCCHAPTER - GEOMETRYEXERCISE - INTRODUCTORY EXERCISE - 12.1
45 Videos
- GEOMETRYBOOK - ARIHANT SSCCHAPTER - GEOMETRYEXERCISE - INTRODUCTORY EXERCISE - 12.2
100 Videos
- GEOMETRYBOOK - ARIHANT SSCCHAPTER - GEOMETRYEXERCISE - EXERCISE(LEVEL 2)
52 Videos
- FUNDAMENTALS BOOK - ARIHANT SSCCHAPTER - FUNDAMENTALS EXERCISE - TEST OF YOU - LEARNING - 2
40 Videos
- HCF AND LCMBOOK - ARIHANT SSCCHAPTER - HCF AND LCMEXERCISE - Exercise Higher Skill Level Questions
18 Videos
Similar Questions
Knowledge Check
If the diagonals of a quadrilateral bisect each other at right angles, then this quadrilateral is
Aa rectangleBa rhombusCa kiteDnone of these
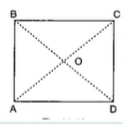
In figure, Show that if the diagonals of a quadrilateral are equal and bisect each other at right angle, then it is a square.
View SolutionIf the diagonals of a quadrilateral bisect each other at right angle, then the quadrilateral is a parallelogram (b) rectangle (c) rhombus (d) kite
View SolutionShow that the diagonals of a square are equal and bisect each other at right angles.
View SolutionShow that the diagonals of a square are equal and bisect each other at right angles.
View SolutionIf the diagonals of a quadrilateral bisect each other at right angles, then this quadrilateral is a
View SolutionIf the diagonals of a quadrilateral bisect each other at right angle, prove that the quadrilateral is a rhombus.
View SolutionThe diagonals of a rectangle are equal and bisect each other
View Solution
