Consider the following statements
I. The tangent of a circle is a line that meets the circle in one and only one point.
II. The tangent of a circle at the end point of the diameter is perpendicular to the diameter.
Which of the above statements is/are correct?
Only I
Only II
Both I and II
Neither I nor II
The correct Answer is:C
To solve the problem, we need to analyze the two statements regarding the tangent of a circle.
Step 1: Analyze Statement I
- Statement I states: "The tangent of a circle is a line that meets the circle in one and only one point."
- By definition, a tangent to a circle is indeed a line that touches the circle at exactly one point. This is a fundamental property of tangents. Therefore, Statement I is correct.
Step 2: Analyze Statement II
- Statement II states: "The tangent of a circle at the end point of the diameter is perpendicular to the diameter."
- To understand this, consider a circle with a diameter. If we take one endpoint of the diameter and draw a tangent line at that point, the tangent line will be perpendicular to the radius of the circle at that point. Since the radius at the endpoint of the diameter is also part of the diameter, the tangent will indeed be perpendicular to the diameter at that endpoint. Thus, Statement II is also correct.
Conclusion:
Both statements I and II are correct.
Final Answer:
Both statements are correct.
---
- Statement I states: "The tangent of a circle is a line that meets the circle in one and only one point."
- By definition, a tangent to a circle is indeed a line that touches the circle at exactly one point. This is a fundamental property of tangents. Therefore, Statement I is correct.
- Statement II states: "The tangent of a circle at the end point of the diameter is perpendicular to the diameter."
- To understand this, consider a circle with a diameter. If we take one endpoint of the diameter and draw a tangent line at that point, the tangent line will be perpendicular to the radius of the circle at that point. Since the radius at the endpoint of the diameter is also part of the diameter, the tangent will indeed be perpendicular to the diameter at that endpoint. Thus, Statement II is also correct.
Both statements I and II are correct.
Both statements are correct.
Topper's Solved these Questions
- GEOMETRYBOOK - ARIHANT SSCCHAPTER - GEOMETRYEXERCISE - INTRODUCTORY EXERCISE - 12.1
45 Videos
- GEOMETRYBOOK - ARIHANT SSCCHAPTER - GEOMETRYEXERCISE - INTRODUCTORY EXERCISE - 12.2
100 Videos
- GEOMETRYBOOK - ARIHANT SSCCHAPTER - GEOMETRYEXERCISE - EXERCISE(LEVEL 2)
52 Videos
- FUNDAMENTALS BOOK - ARIHANT SSCCHAPTER - FUNDAMENTALS EXERCISE - TEST OF YOU - LEARNING - 2
40 Videos
- HCF AND LCMBOOK - ARIHANT SSCCHAPTER - HCF AND LCMEXERCISE - Exercise Higher Skill Level Questions
18 Videos
Similar Questions
Consider the following statements :
1. The tangent of a circle is a line that meets the circle in one and only one point-
2. The tangent of a circle at the end point of the diameter is perpendicular to the diameter.
Which of the above statements is/are correct ?
View SolutionComplete the following activity to draw a tangent to a circle at a point on the circle
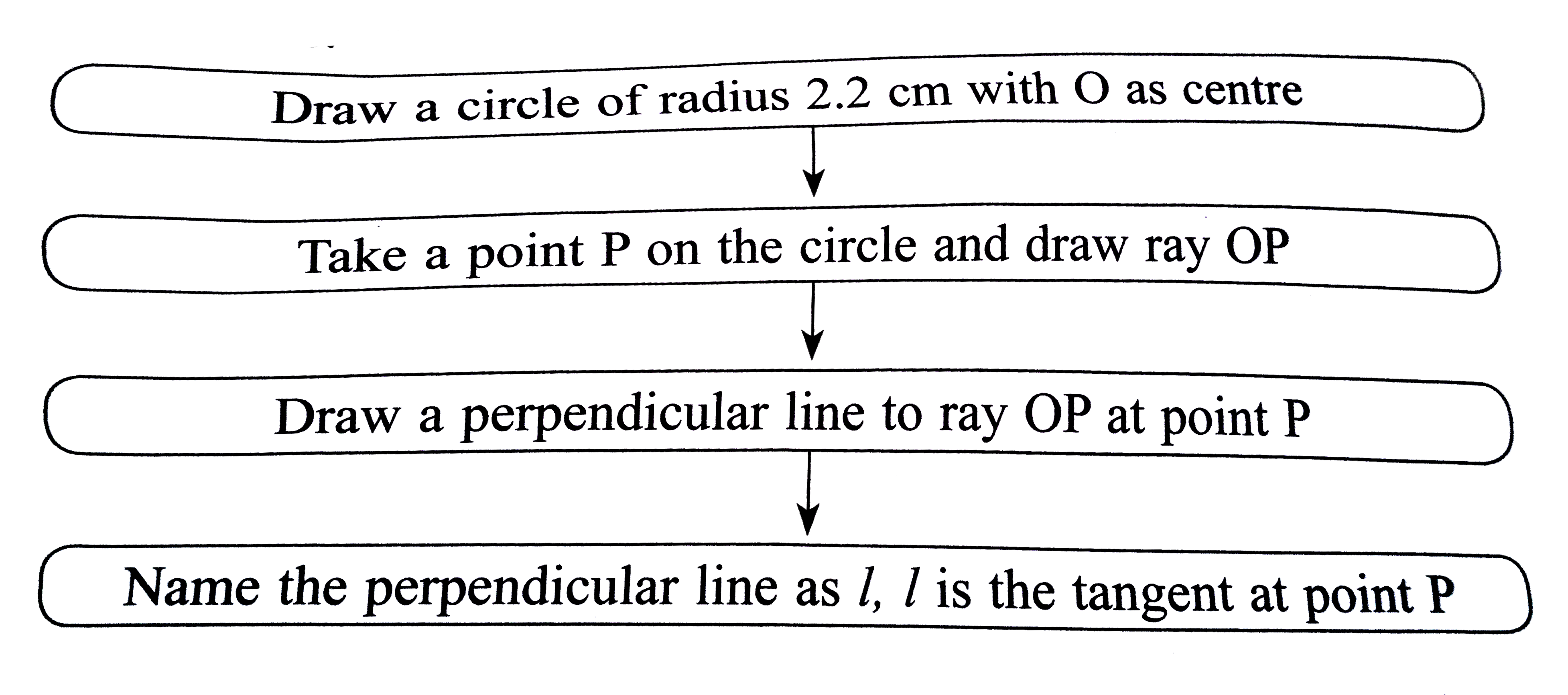
View Solution
Knowledge Check
The tangents drawn at the end points of the diameter of a circle will be
Aperpendicular to each otherBparallel to each otherCinclined to each other at 45∘Dinclined to each other at 60∘Tangents drawn at the end points of a diameter are
APerpendicularBParallelCIntersectingDNone of theseA tangent at any point of a circle is perpendicular to the radius through the _____.
AchordBdiameterCpoint of contactDall of the above
Show that tangent lines at the end points of a diameter of a circle are parallel.
View SolutionA line which bisects the diameter of a circle is perpendicular to the diameter. [True / False]
View SolutionA tangent to a circle intersects it in _________ points.
View SolutionDraw the tangent to a circle of diameter 4 cm at a point P on it.
View SolutionProve that the tangent at any point of circle is perpendicular to the radius through the point of contact.
View Solution
