Question

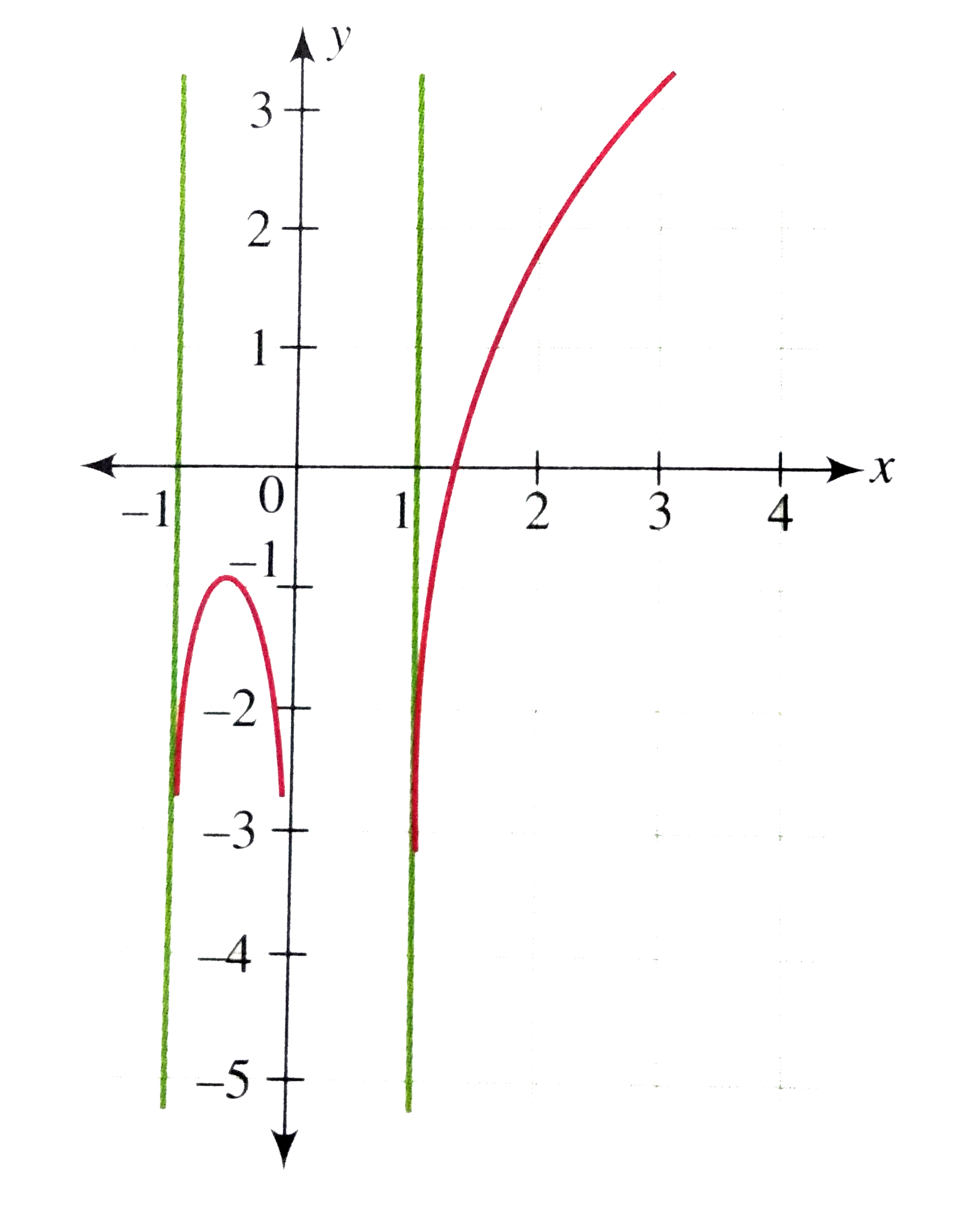
Draw the graph of $y={\log }_e(x^3-x)$

Explanation

`y=f(x)=log_(e)(x^(3)-x)`
Here the function is defined if `x^(3)-x ge0`
or `x(x-1)(x+1) ge0`
or `x in (-1,0) cup (1,infty)`
`underset(xto 1^(+))"lim"log_(e)(x^(3)-x)=log_(e)0^(+)=-infty`
`underset(x to 0^(-)) "lim"log_(e)x(x^(2)-1)=log_(e)0^(+)=-infty`
`underset(xto -1^(+)) log_(e)x(x^(2)-1) = log_(e)0^(+)=-infty`
For `x gt 1, x^(3)-x` increases, so `log_(e)(x^(3)-x)` increases till infinity.
Also for `f^(')(x) = (3x^(2)-1)/(x^(3)-1)=0, x=-1/sqrt(3)` [as domain is `(-1,0) cup (1, infty)]`
Clearly, `x=-1/sqrt(3)` is the point of maxima.
The graph of the function is as shown in the following figure.