Question

Draw the graph of $f\left(x\right)=e^{-x^2}$. Discuss the concavity of the graph.

Explanation

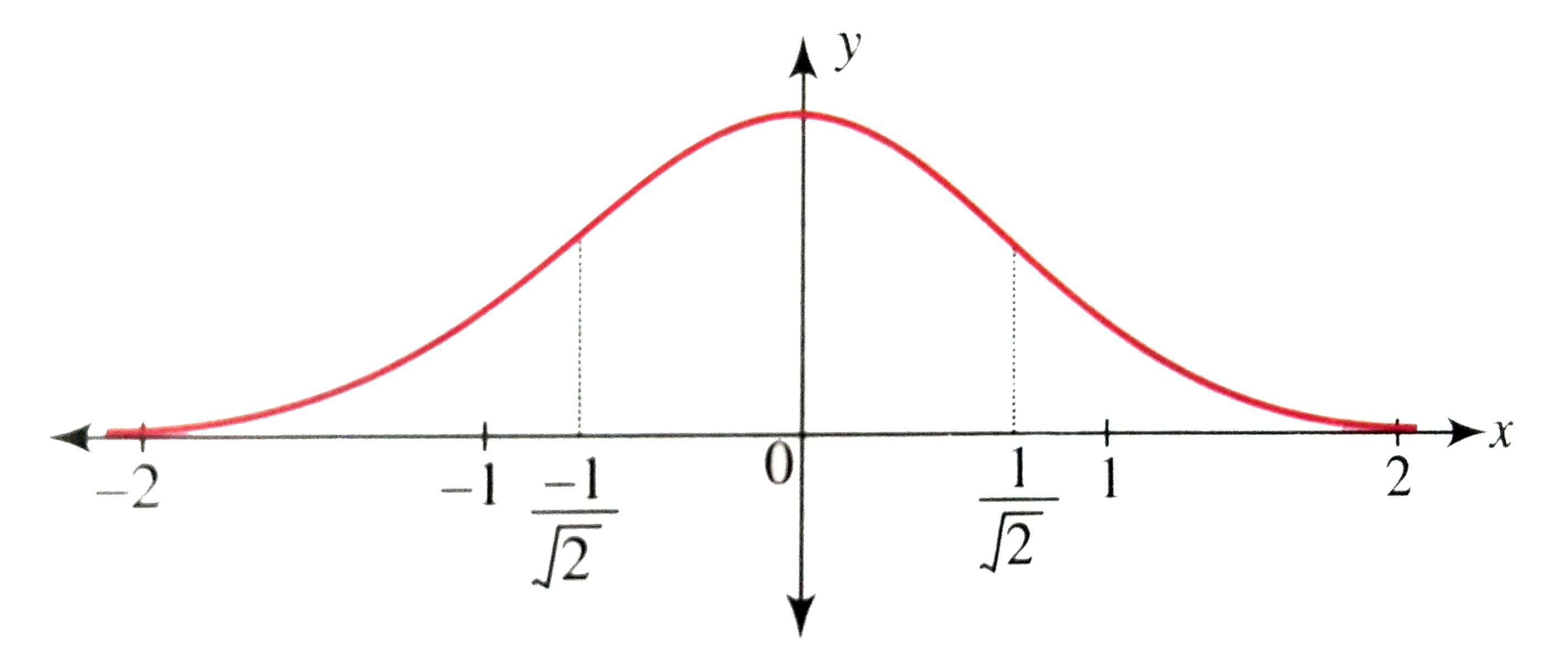
We have `y=f(x) = e^(-x^(2))`
Since, `-infty lt -x^(2) le0`, we have `0 lt e^(-x^(2)) le1`
`f^(x) gt 0` for `x lt0` where `f(x)` increases and `f^(')(x) lt 0` for `x gt 0` where `f(x)` decreases.
`f^('')(x)=-2e^(-x^(2)) + 4x^(2)e^(-x^(2))=2e^(-x^(2))(2x^(2)-1)`
`f^('')(x) therefore x= +- 1/sqrt(2)`, where the graph changes concavity.
`|x| gt 1/sqrt(2)` where the graph is concave upward.
The graph of the function is as shown in the figure.