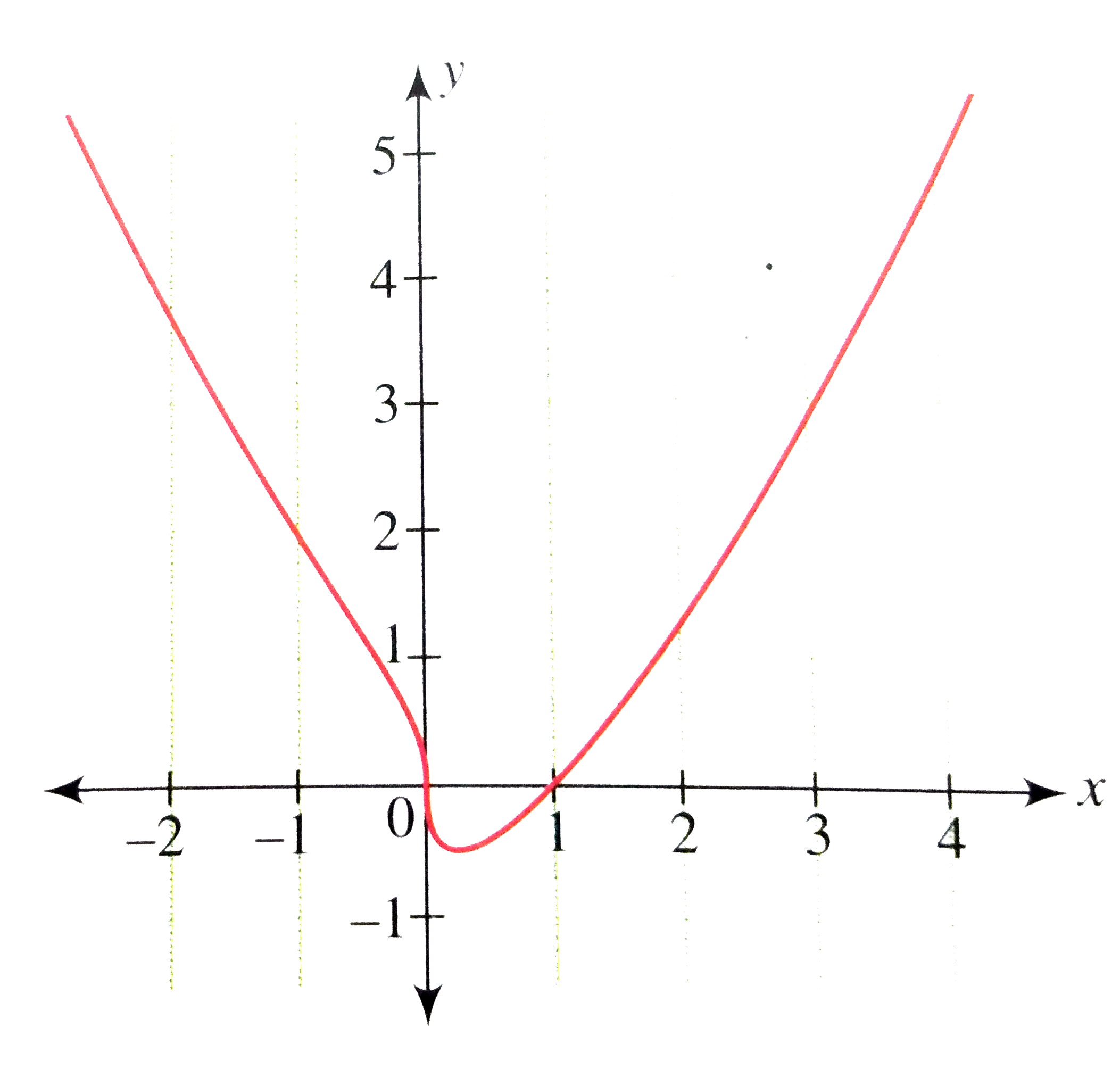
The function $f (x) = x^{\frac{1}{3}} (x - 1)$ has two inflection points has one point of extremum is non-differentiable has range $[- 3 x 2^{- \frac{8}{3}}, \infty)$

Video Solution

Text Solution

Verified by Experts

$y = x^{1 / 3} (x - 1)$
$\frac{d y}{d x} = \frac{4}{3} x^{1 / 3} - \frac{1}{3.1} / x^{2 / 3} = \frac{1}{3 x^{2 / 3}} [4 x - 1]$
Hence f is increasing for $x > \frac{1}{4}$ and decreasing for $x < \frac{1}{4}$
Thus, $x = 1 / 4$ is the point of minima.
$f^{'} (0)$ does not exist as $f (x)$ is a vertical tangent here.
$f^{''} (x) = \frac{4}{9} . \frac{1}{x^{2 / 3}} + \frac{1}{3} . \frac{2}{3} . \frac{1}{x^{5 / 3}}$
$= \frac{2}{9 x^{2 / 3}} [2 + \frac{1}{x}] = \frac{2}{9 x^{2 / 3}} [\frac{2 x + 1}{x}]$
$∴ f^{''} (x) = 0$ at $x = - \frac{1}{2}$
Thus, $x = - \frac{1}{2}$ is the point of inflection.
From the above discussion, the graph of function is as shown in the following figure.

Updated on:21/07/2023

which of the folloiwng function has point of extremum at x =0?
Af(x) = $e^{- | x |}$
Bf(x) = sin |x|
Cf(x)= ${\begin{matrix} x^{2} + 4 x + 3 & x < 0 - x & x \geq 0 \end{matrix}$
Df(x)= ${\begin{matrix} x^{2} + 4 x + 3 & x < 0 - x & x \geq 0 \end{matrix}$
$f (x) = 2 x^{3} + 3 x^{2} - 12 x + 7$ has maximum at the point
A $(1, 0)$
B $(2, 9)$
C $(- 2, 9)$
D $(- 2, 27)$