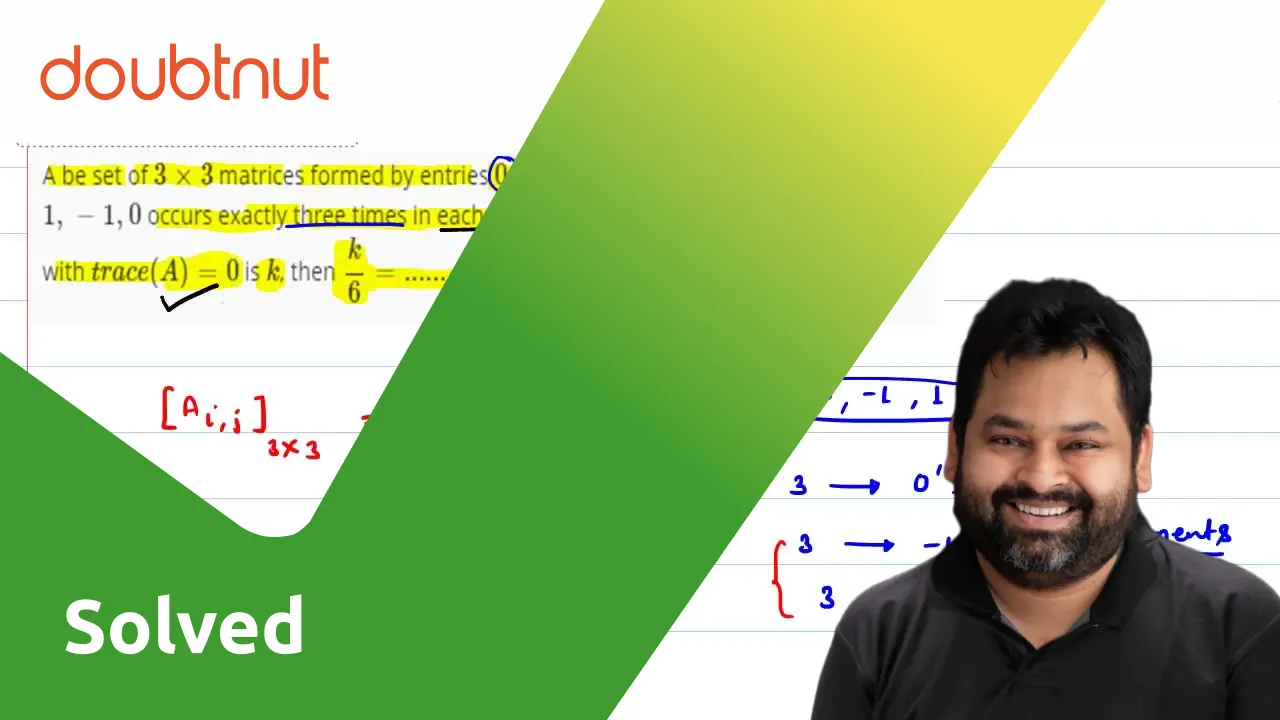
A be set of 3×3 matrices formed by entries 0,−1, and 1 only. Also each of 1,−1,0 occurs exactly three times in each matrix. The number of symmetric matrices with trace(A)=0 is k, then k6=......
Step by step video & image solution for A be set of 3times3 matrices formed by entries 0 , -1, and 1 only. Also each of 1,-1,0 occurs exactly three times in each matrix. The number of symmetric matrices with trace(A)=0 is k, then (k)/(6)=...... by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Similar Questions
Let A be the set of all 3 xx 3 symmetric matrices all of whose entries are either 0 or 1. Five of these entries are 1 and four of them are 0. The number of matrices in A is
10:10View SolutionA such 3xx3 Is a set of symmetric matrices whose all entries () are 0 or 1. Five of these entries are 1 and four are 0. The number of matrices A in A for which an exponential equation body A[(x),(y),(z)]=[(1),(0),(0)] Unique solution of is
07:06View SolutionA such 3xx3 Is a set of symmetric matrices whose all entries () are 0 or 1. Five of these entries are 1 and four are 0. A has matrices
06:20View SolutionA such 3xx3 Is a set of symmetric matrices whose all entries () are 0 or 1. Five of these entries are 1 and four are 0. The number of matrices A in A for which an exponential equation body A[(x),(y),(z)]=[(1),(0),(0)] Unique solution of is
Text SolutionView SolutionA such 3xx3 Is a set of symmetric matrices whose all entries () are 0 or 1. Five of these entries are 1 and four are 0. The number of matrices A in A for which an exponential equation body A[(x),(y),(z)]=[(1),(0),(0)] Is incompatible
08:16View SolutionLet A be the set of all 2×2 matrices whose determinant values is 1 and B be the set of all 2×2 matrices whose determinant value is -1 and each entry of matrix is either 0 or 1, then
03:48View SolutionLet A be the set of all 3 xx 3 symmetric matrices all of whose entries are either 0 or 1. Five of these entries are 1 and four of them are 0. The number of matrices in A is
05:28View SolutionLet A be the set of all 3 xx 3 symmetric matrices all of whose entries are either 0 or 1. Five of these entries are 1 and four of them are 0. The number of matrices in A is
05:42View Solution