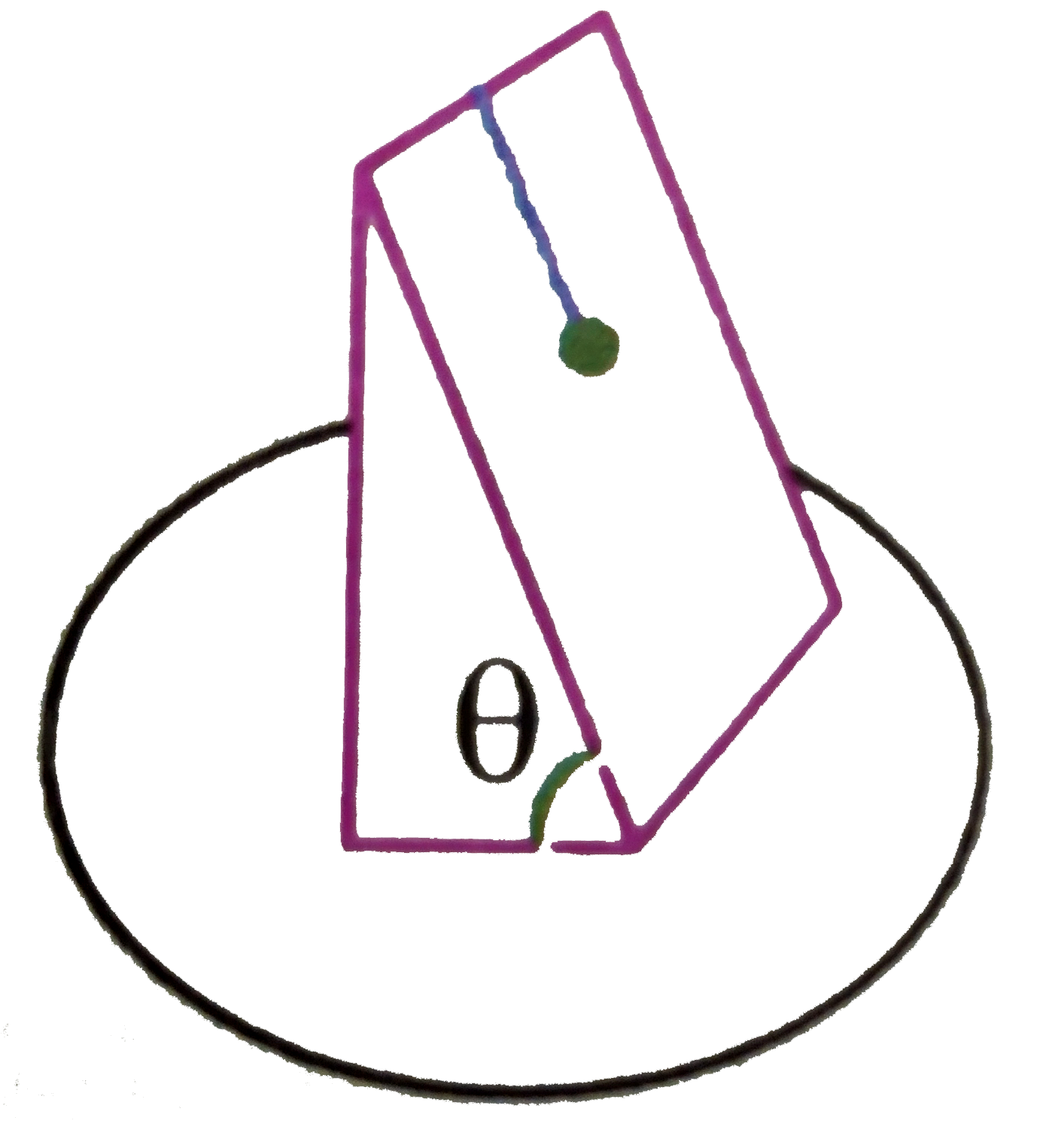
A simple pendulum is suspnded from the ceilignof a car accelerating uniformly on a horizontal road.If the acceleration is $a_{0}$ and the length of the pendulum is l, find the time period of small oscillations about the mean position.

Video Solution

Text Solution

Verified by Experts

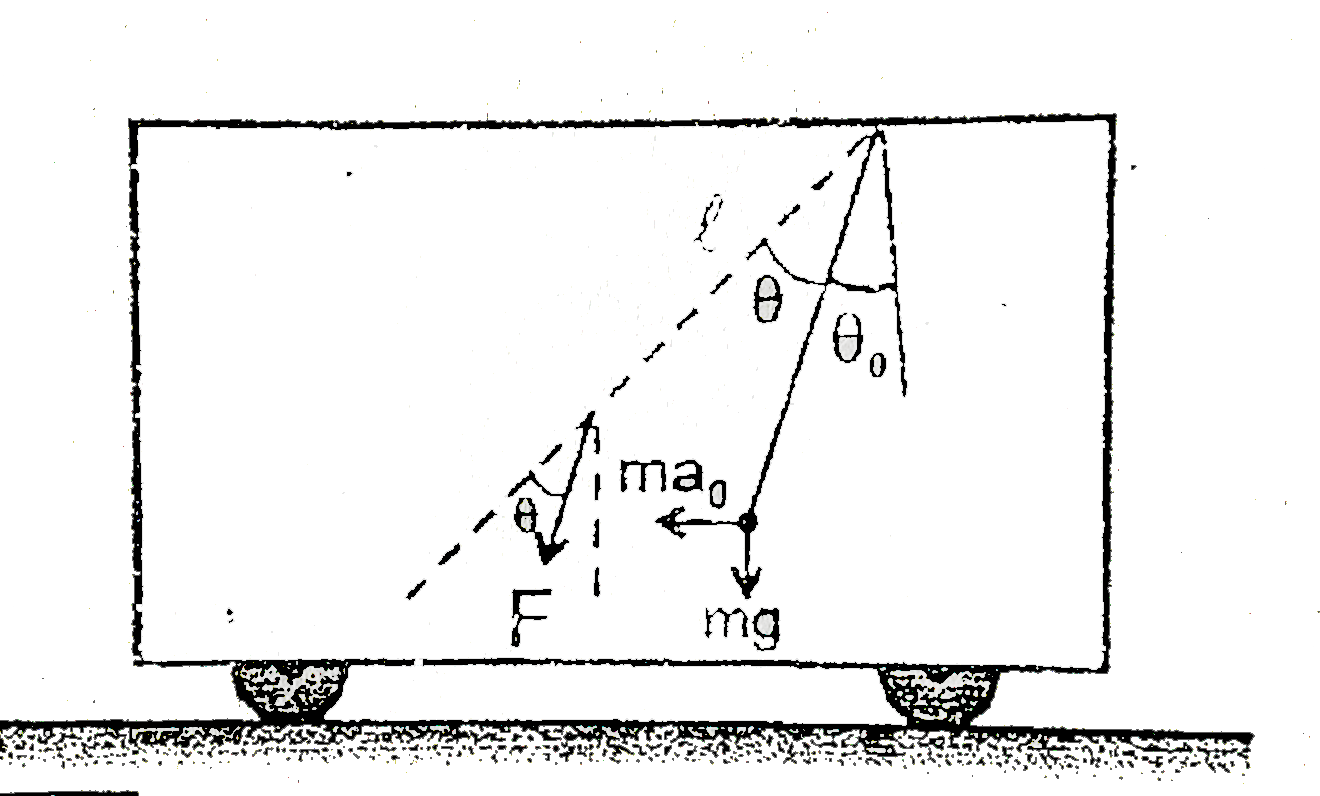
We shall work in the car frame. As it is accelerated with respect to the road, we shall have to apply a pseudo force $m a_{0}$ on the bob of mass $m$ .
For mean position, the acceleration of the bob with respect to the car should be zero. If $θ_{0}$ be the angle made by the string with the vertical, the tension, weight and the pseudo force will add to zero in this position.
Hence, resultant of $m g$ and $m a_{0}$ (say $F = m \sqrt{g^{2} + a_{0}^{2}}$ ) has to be along the sting.
$∴ {\tan θ}_{0} = \frac{m a_{0}}{m g} = \frac{a_{0}}{g}$
Now, suppose the string is further deflected by an angele $θ$ as shown in figure.
Now, restoring torque can be given by
$(F \sin θ) l = - m l^{2} α$
Substituting $F$ and using $\sin θ = θ$ , for small $θ$ .
$(m \sqrt{g^{2} + a_{0}^{2}}) l θ = - m l^{2} α$
or, $α = - \frac{\sqrt{g^{2} + a_{0}^{2}}}{l} θ$ so, $ω = \frac{\sqrt{g^{2} + a_{0}^{2}}}{l}$
This is an equation of simple harmonic motin with time period
$T = \frac{2 π}{ω} = 2 π \frac{\sqrt{l}}{{(g^{2} + a_{0}^{2})}^{1 / 4}}$

Updated on:21/07/2023

A simple pendulum with a bob of mass m is suspended from the roof of a car moving with a horizontal acceleration a.
AThe string makes an angle of ${tan}^{- 1} (a / g)$ with the vertical
BThe string makes an angle of ${tan}^{- 1} (1 - \frac{a}{g})$ with the vertical
CThe tension in the string is $m \sqrt{a^{2} + g^{2}}$
DThe tension in the string is $m \sqrt{g^{2} - a^{2}}$
A simple pendulum $50 c m$ long is suspended from the roof of a acceleration in the horizontal direction with constant acceleration $\sqrt{3} g m / s^{- 1}$ . The period of small oscillations of the pendulum about its equilibrium position is $(g = π^{2} m / s^{2})$ ltbRgt
A $1.0 sec$
B $1.25 sec$
C $1.53 sec$
D $1.68 sec$
A simple pendulum of length L is suspended from the roof of a train. If the train moves in a horizontal direction with an acceleration 'a' then the period of the simple pendulum is given by
A $T = 2 π \sqrt{\frac{l}{g}}$
B $T = 2 π \sqrt{\frac{l}{g + a}}$
C $T = 2 π \sqrt{\frac{l}{(g^{2} + a^{2})}}$
D $T = 2 π \sqrt{\frac{l}{g - a}}$