Figure shows a circuit consisting of an ideal cell, an inductor L, and a resistor R, connected in series. Let switch S be closed at t=0. Suppose at t=0, the current in the inductor is io, then find out the equation of current as a function of time.

Let an instant t current in the circuit is i which is increasing at the rate di/dt
Writing KVL along the circuit, we have ε−Ldidt−iR=0
⇒Ldidt=ε−iR⇒i∫i0diε−iR=t∫0dtL
⇒ln(ε−iRε−i0R)=−RtL
⇒ε−iR=(ε−i0R)e−Rt/L⇒i=ε−i(ε−i0R)eRt/LR
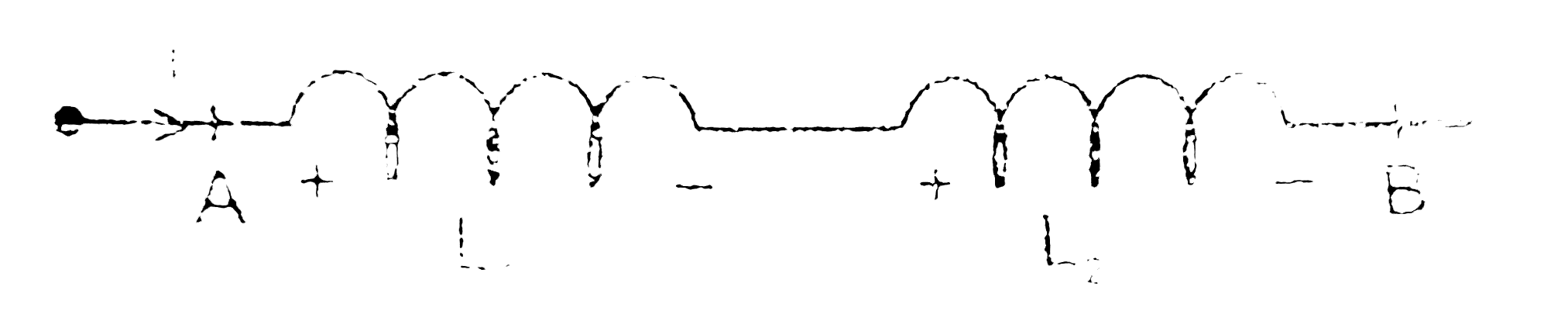
Equivalent self inductance:
Series combination:
from (1) and (2) L=L1+L2(neglecting mutual inductance)
Parallel Combination:
From figure VA−VB=L1dt1dt=L2dt2dt ...(3)
also i=i1+i2
or didt=di1dt+di2dt
or VA−VBL=VA−VBL1+VA−VBL2 (neglecting mutual inductance)
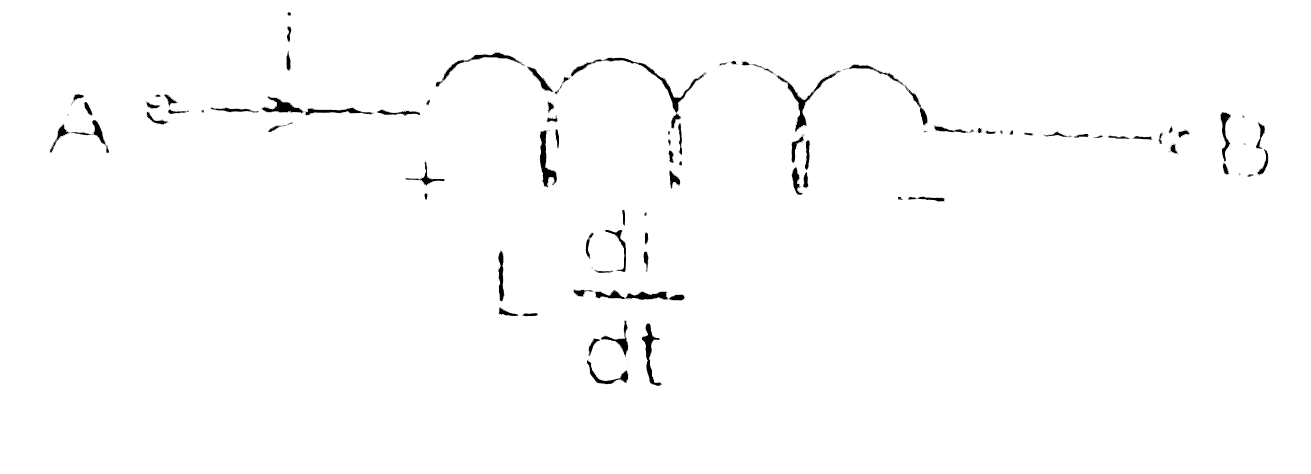
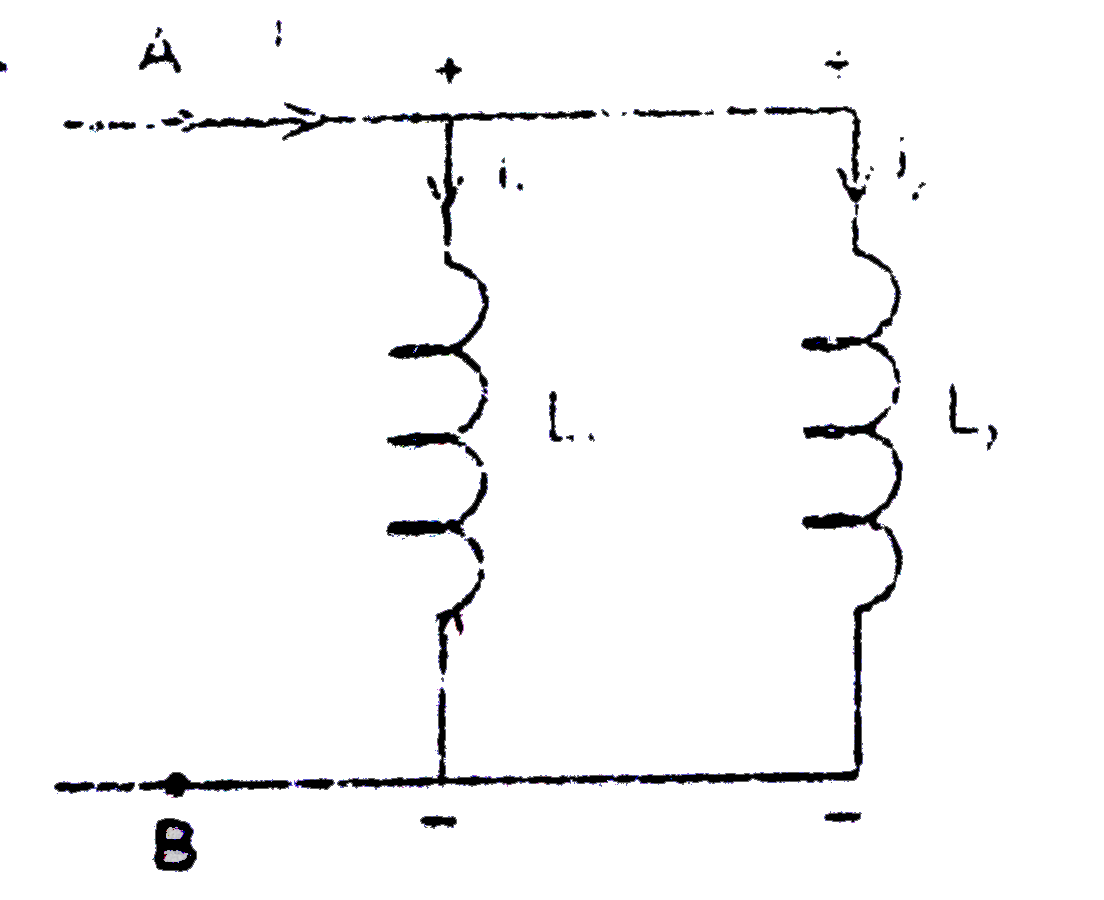
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - RESONANCECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Problem
12 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - RESONANCECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercis-1
63 Videos
- ELECTRODYNAMICSBOOK - RESONANCECHAPTER - ELECTRODYNAMICSEXERCISE - Advanced level problems
31 Videos
- ELECTROSTATICSBOOK - RESONANCECHAPTER - ELECTROSTATICSEXERCISE - HLP
39 Videos
Similar Questions
In the following circuit (Fig.)the switch is closed at t=0. Intially, there is no current in inductor. Find out the equation of current in the inductor coil as s function of time.
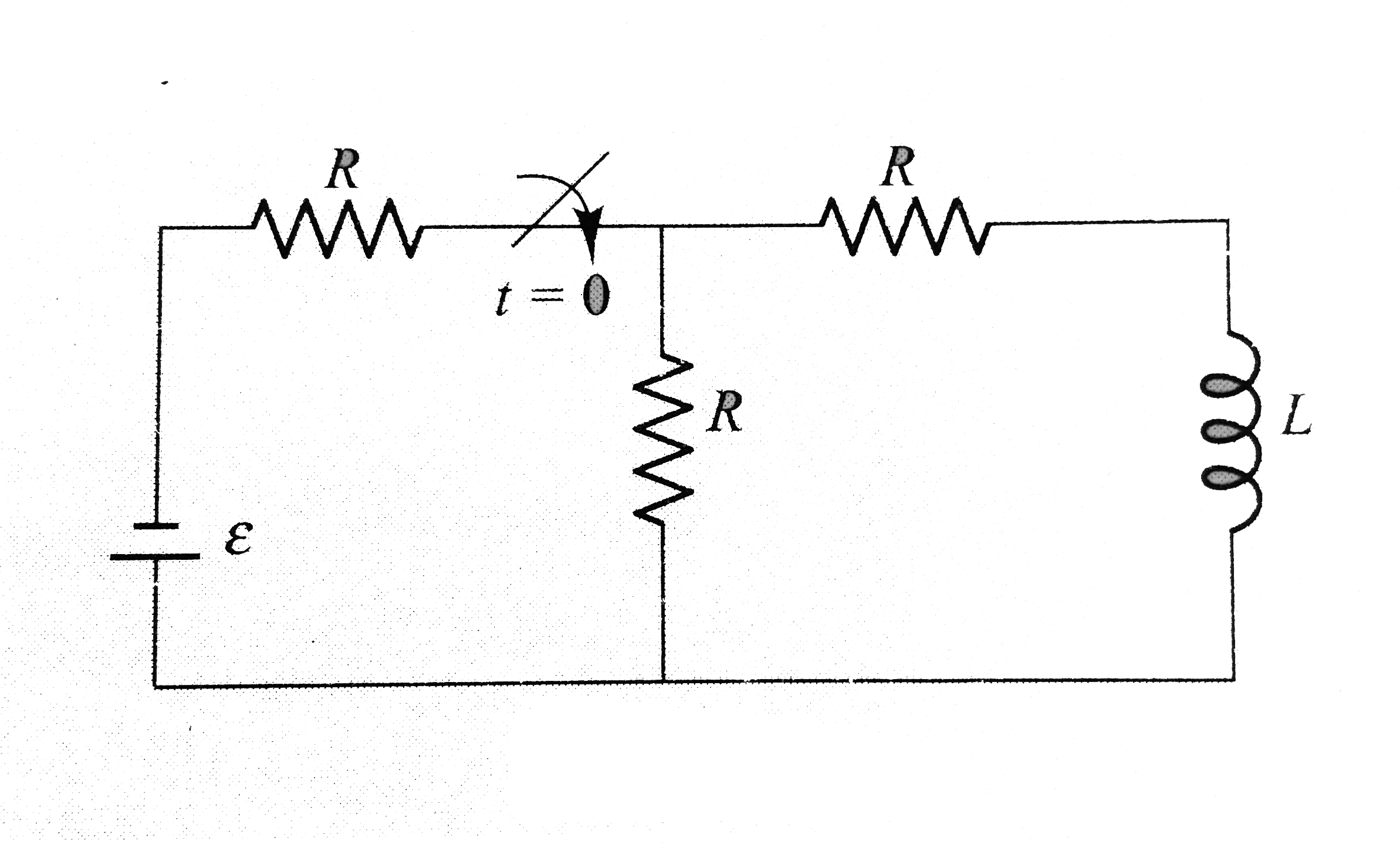
View SolutionIn the circuit shown, switch S is closed at time t=0. Find the current through the inductor as a function of time t.

View Solution
Knowledge Check
In adjacent circuit, switch S is closed at t = 0. The time at which current in the circuit becomes half of the steady current is
 Aτln2Bln2τC2τln2Dτ2ln2
Aτln2Bln2τC2τln2Dτ2ln2
The switch in figure is closed at time t=0. Find the current in the inductor and the current through the switch as functions of time thereafter.
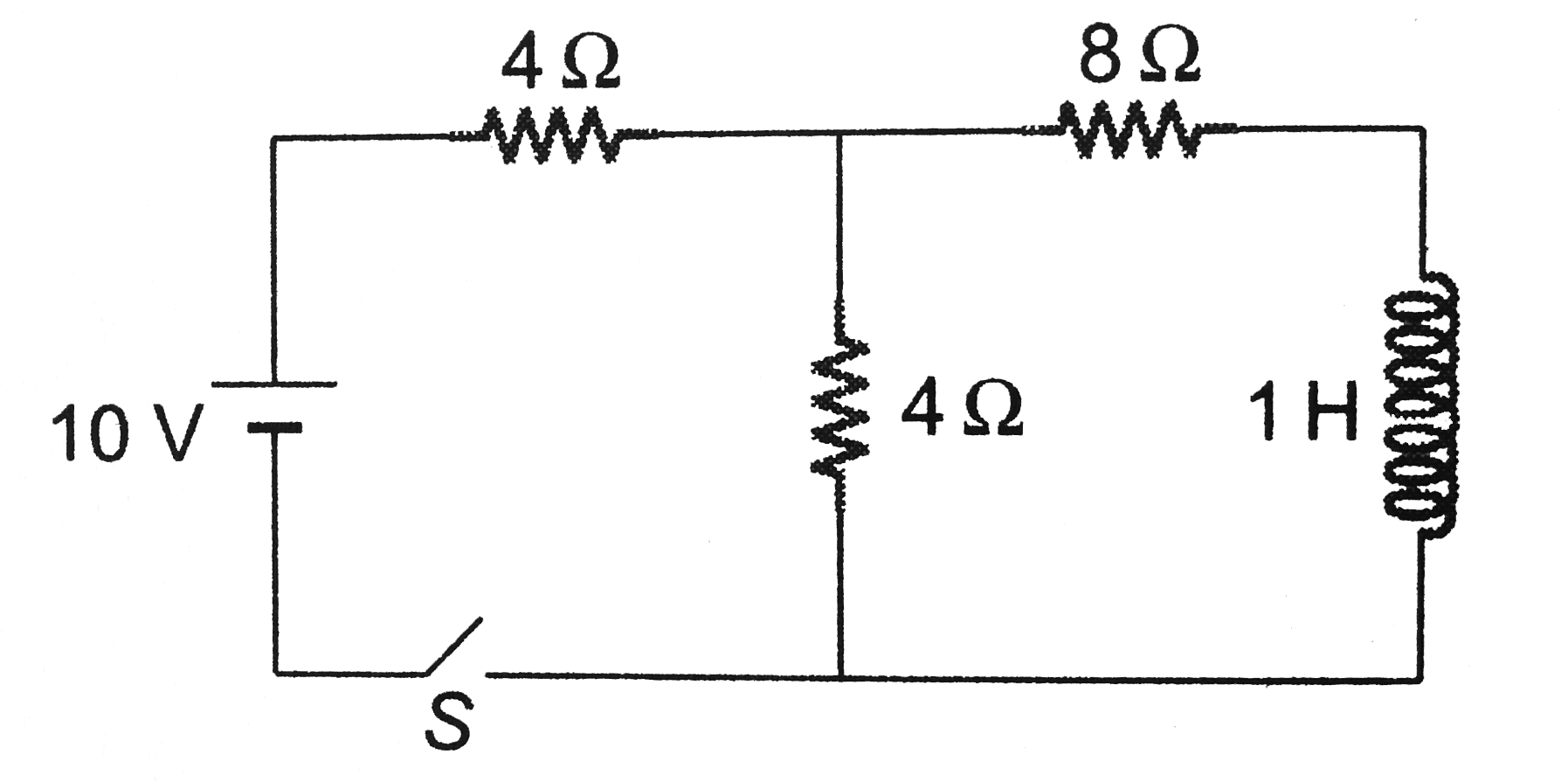
View SolutionSwitch is closed at t=0 then the current in the circuit at t=L2R is
_PHY_E06_157_Q01.png)
View SolutionA circuit contains an ideal cell and an inductor with a switch. Initially, the switch is open. It is closed at t=0. Find the current as a function of time.
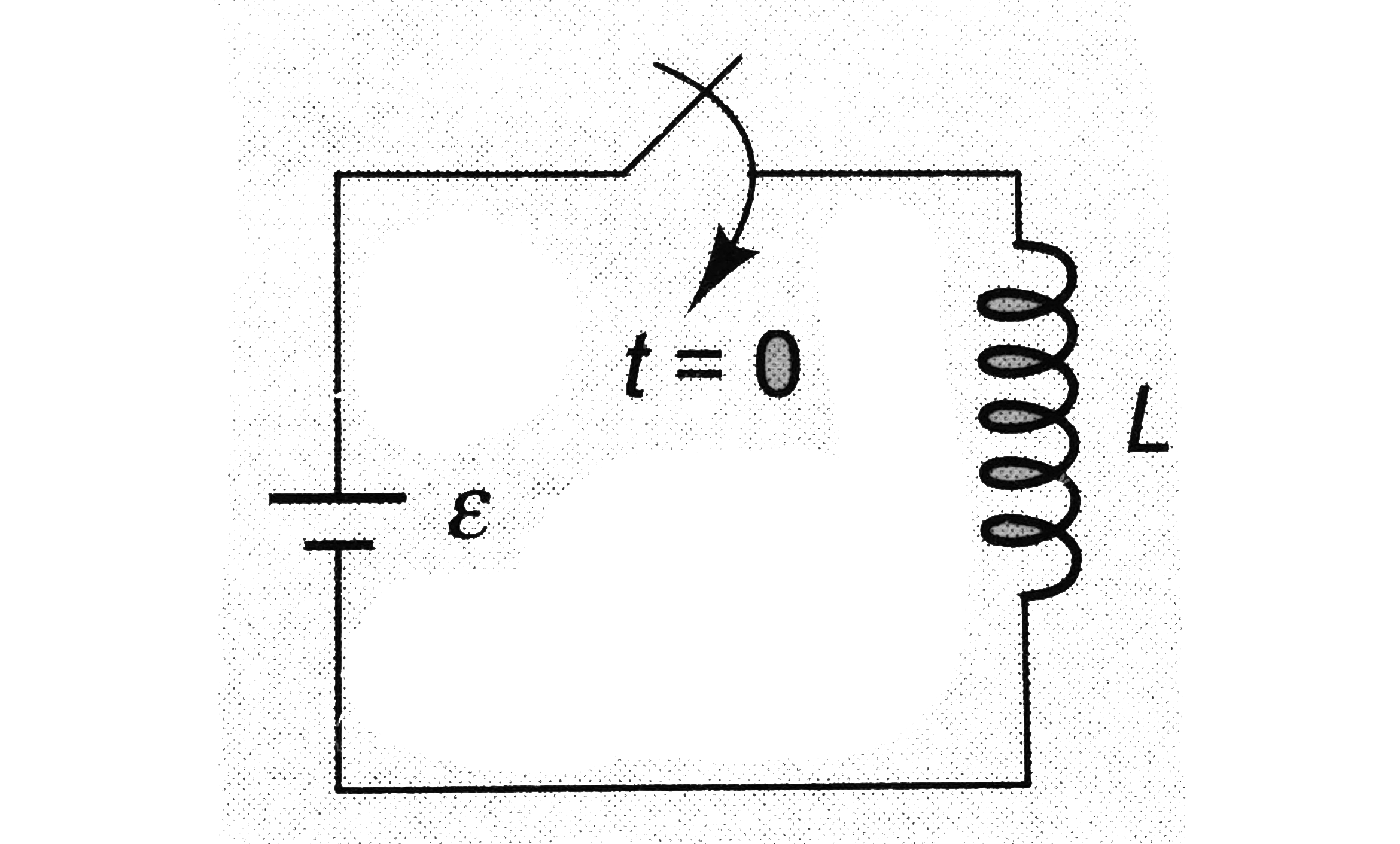
View SolutionAt t=0 , switch S is closed. Find time constant of the circuit and current through inductor as a function of time t .

View SolutionA circuit consisting of a capacitor with capacitance C and a resistance R connected in series was connected at the moment t=0 to a source of ac voltage V=Vmcosωt . Find the current in the circuit as a function of time t.
View SolutionIn the following circuit the switch is closed at t=0.Intially there is no current in inductor.Find out current the inductor coil as a function of time.
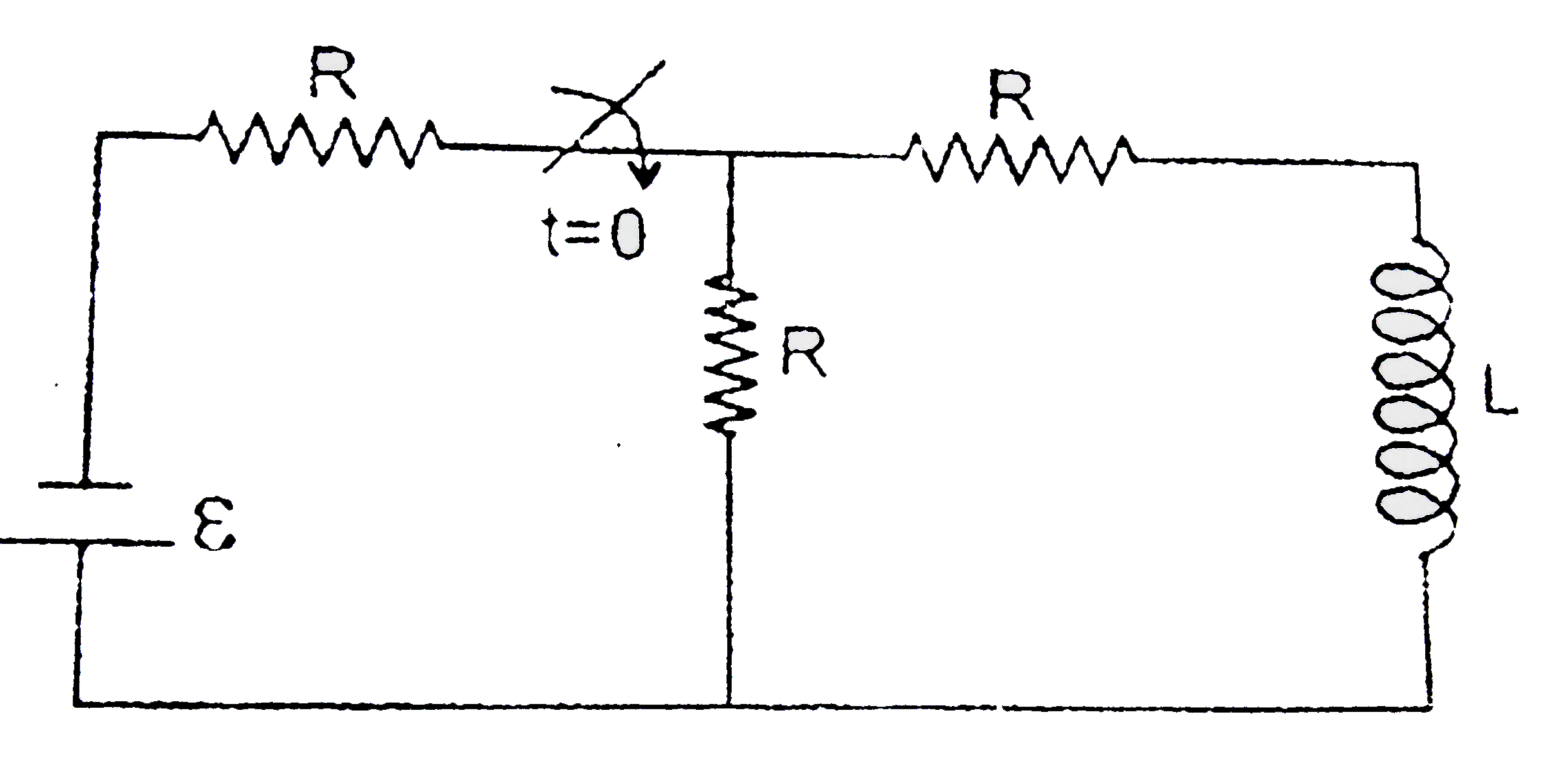
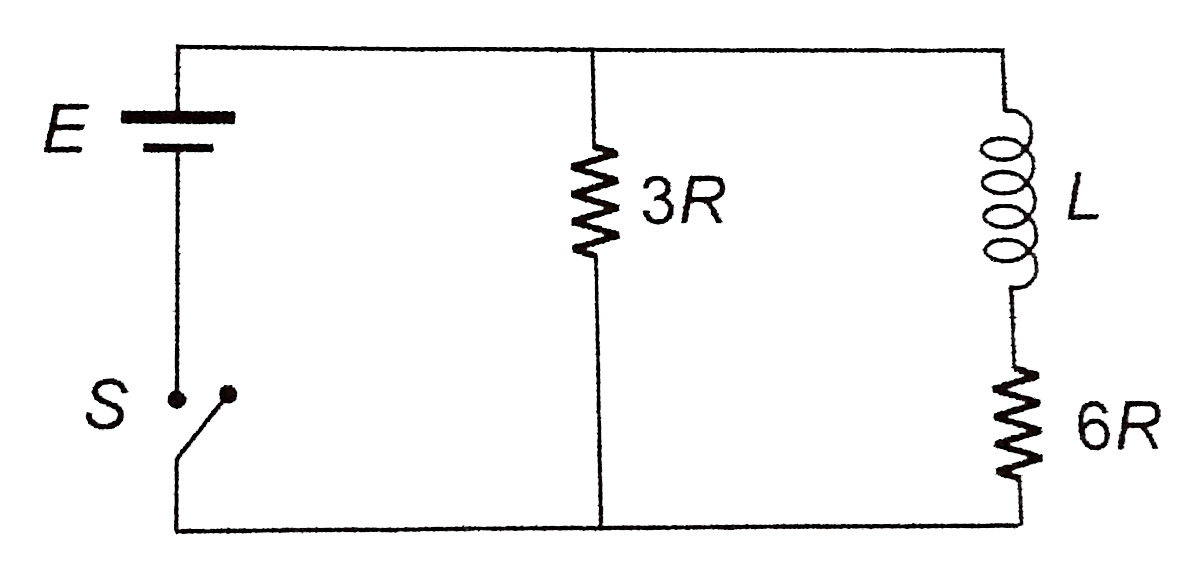
View SolutionSwitch S is cloesd in the circuit at time t=0. Find the current through the capacitor and the inductor at any time t
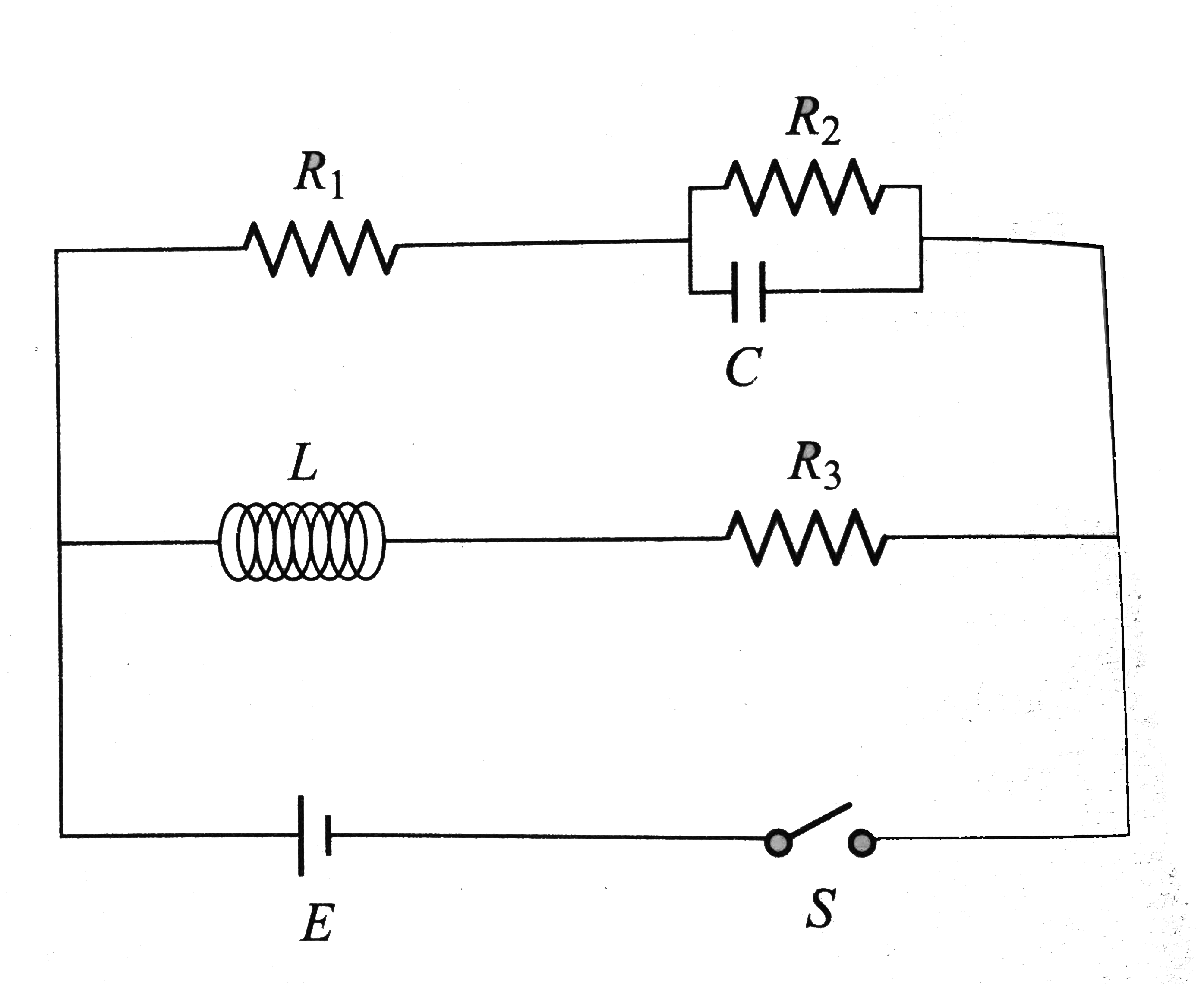
View Solution