A mass m is tied to a string of length l and is rotated in a vertical circle with centre at the other end of the string.
(a) Find the minimum velocity of the mass at the top of the circle so that it is able to complete the circle.
(b) Find the minimum velocity at the bottom of the circle corresponding to the above condition.

Video Solution

Text Solution

Verified by Experts

At all positions, there are two forces acting on the mass :
its own weight and the tension in the string.
Here radius of the circle = l
(a) At the top : Let $v_{t} =$ velocity at the top
Net force towards centre = $\frac{m v_{t}^{2}}{l}$ (centripital force)
$T + m g = \frac{m v_{t}^{2}}{l} \Rightarrow T = \frac{m v_{i}^{2}}{l} - m g$

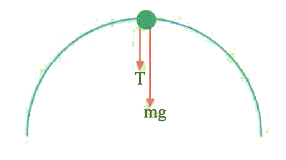
For the movement in the circular path, the string should remain tight i.e. the tension must be positive at all positions. As the tension is minimum at the top, $T_{⊤} \geq 0$
$\Rightarrow (m \frac{v_{t}^{2}}{l} - m g \geq 0$ $\Rightarrow v_{i} \geq \sqrt{l g}$
$\Rightarrow$ minimum or critical velocity at the top
$= \sqrt{l} g$ (b) Let $v_{b}$ be the velocity at the bottom. As the particle goes up, its KE decreases and GPE increases.
$(# # N A R_{N} \exists T_{P} H Y_{X} I_{P} 2_{C} 06_{S} L V_{034}_S 02 . p n g width= 80 % > < b r >$ Rightarrow $l o s s \in K E = g a \in \in G P E < b r >$ Rightarrow 1/2(mv_(b)^(2) - 1/2 (mv_(t)^(2) = mg(2l) $< b r >$ v_(b)^(2) = v_(t)^(2) + 4 gl $< b r >$ (v_(b))_(min) = sqrt((v_(t)^(2))_(min) + 4gl ) = sqrt 5gl

Updated on:21/07/2023

A stone of mass m is tied to a string of length l and rotated in a circle with a constant speed v . If the string is released, the stone flies
ARadially outward
BRadialy inward
CTangentially outward
DWith acceleration $\frac{m v^{2}}{l}$
A stone of mass m tied to a string of length l is rotated in a circle with the other end of the string as the centre. The speed of the stone is v. If the string breaks, the stone will move
Amove towards the centre
Bmove away from the centre
Cmove along a tangent
Dstop
A point mass of 2 kg tied to a string of 1 m length is rotated in a vertical circle with uniform speed of 4m/s. The tension in the string is nearly 32 N when mass is at
Abotton
Bmid- way
Chighest point
Dbetween bottom and mid way