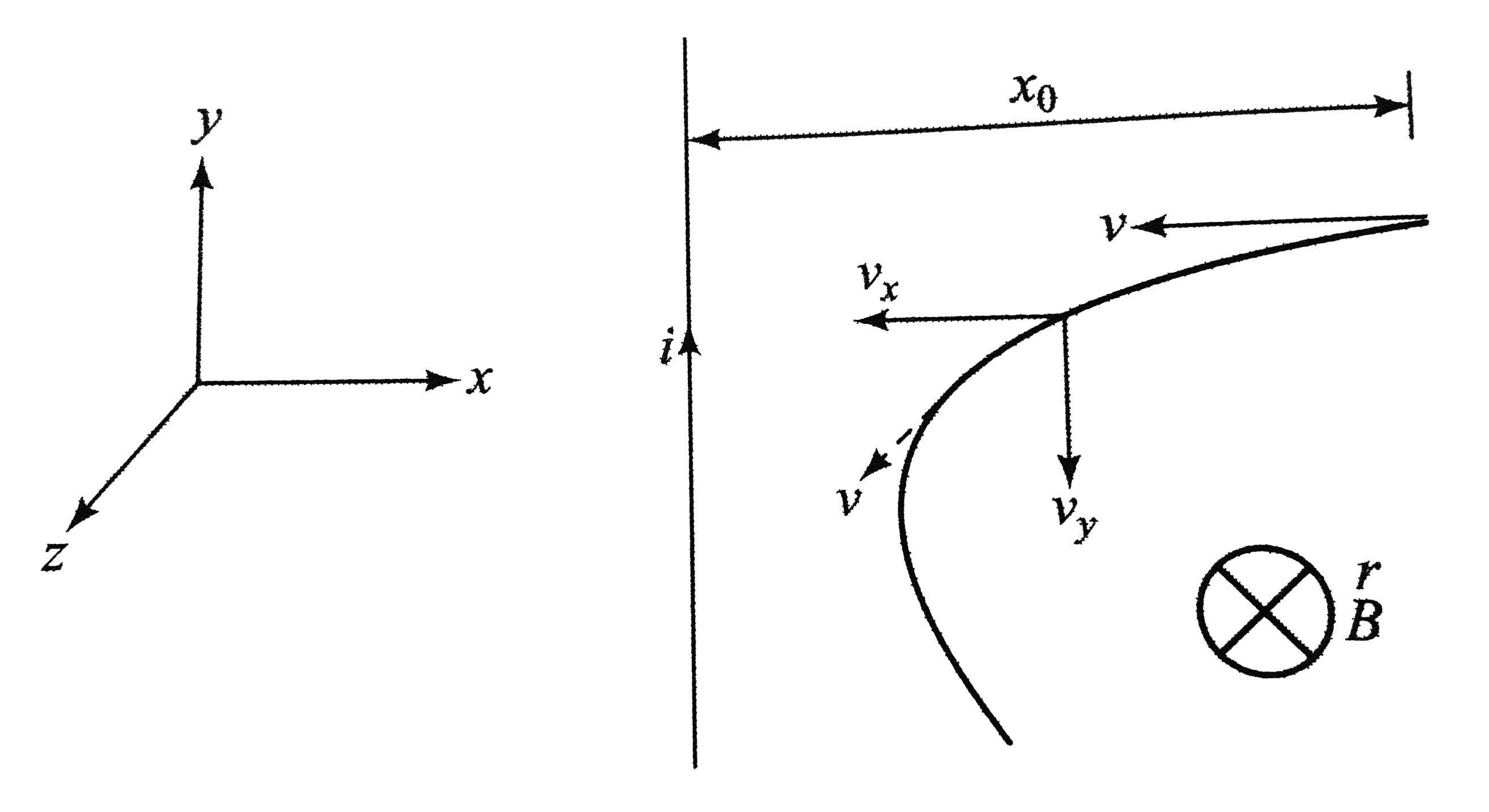
A positive point charge q of mass m, kept at a distance x0(in the same plane) from a fixed very long straight current is projected normally away from it with speed v.Find the maximum separation between the wire and the particle.
Since the magnetic field is not unifrom, the particle doesn't follws circular path but the speed (v) of the particle is constant. Here the magnetic field set-up by the staight current is along the negative z-axis, the initially velocity of the particle is along x-axis and the froce F is in this x−y plane. The force at time t after starting from point P is
F=q(v×B)
or F=q[(vxˆi+vyˆj)×(μ0I2πx(−k))]=μ0qI2πx(−vy+vxˆj)
So, Fx=−μ02πqlvyx,∴ax=−μ0qlvy2πmx
or vxdvxdx=μ0qlvy2πmx..(i)
But v2x+v2y=v2
∴2vxdvx+2vydvy=0orvxdvx=−vydvy ..(ii)
From Eqs. (i) and (ii)
2πmμ0qI=dvy=dxxor2πmμ0qIv∫0dvy=t>x0g(x)∫dxxor2πmμ0qIv=lnxx0
∴x=x0e2πmv/μql

Topper's Solved these Questions
- ELECTRODYNAMICSBook:RESONANCEChapter:ELECTRODYNAMICSExercise:Exercise-3 PART-3
Explore 29 Videos
- ELECTRO MAGNETIC WAVESBook:RESONANCEChapter:ELECTRO MAGNETIC WAVESExercise:Exercise 3
Explore 27 Videos
- ELECTROMAGNETIC INDUCTIONBook:RESONANCEChapter:ELECTROMAGNETIC INDUCTIONExercise:A.l.P
Explore 19 Videos
Similar Questions
A long , straight wire carries a current i. A particle having a positive charge q and mass m, kept at a distance x_0 from the wire is projected towards it with a speed v. Find the minimum separation between the wire and the particle.
View SolutionA long straight wire carries a current I0=100amp , a particle having a positive charge q=100C and mass m=10gms is kept at a distance x0=10m from the axis of the wire is projected away from it with a speed v0=10m/sec. Find the maximum separation between particle and wire is found to be y×10m Find y.
View Solution
Knowledge Check
Two point charges q1 and q2 are released from rest in a gravity free hall when distance between them is a. Find the maximum speeds of charged particle. The mass of each charged particle is m.
Aq1q24πε0aB√(q1q2)4πε0ma)C√(2q1q24πε0ma)Dnone of these
A particle of mass m is kept on the axis of a fixed circular ring of mass M and radius R at a distance x from the centre of the ring. Find the maximum gravitational force between the ring and the particle.
View SolutionA long straight wire carries a current i. A particle having a positive charge q and mass m, kept at distance x0 from the wire is projected towards it with speed v. Find the closest distance of approach of charged particle to the wire.

View SolutionA charge "Q" of mass "m" is at rest on a smooth horizontal plane.Another identical particle is projected with a velocity v towards it from long distance then
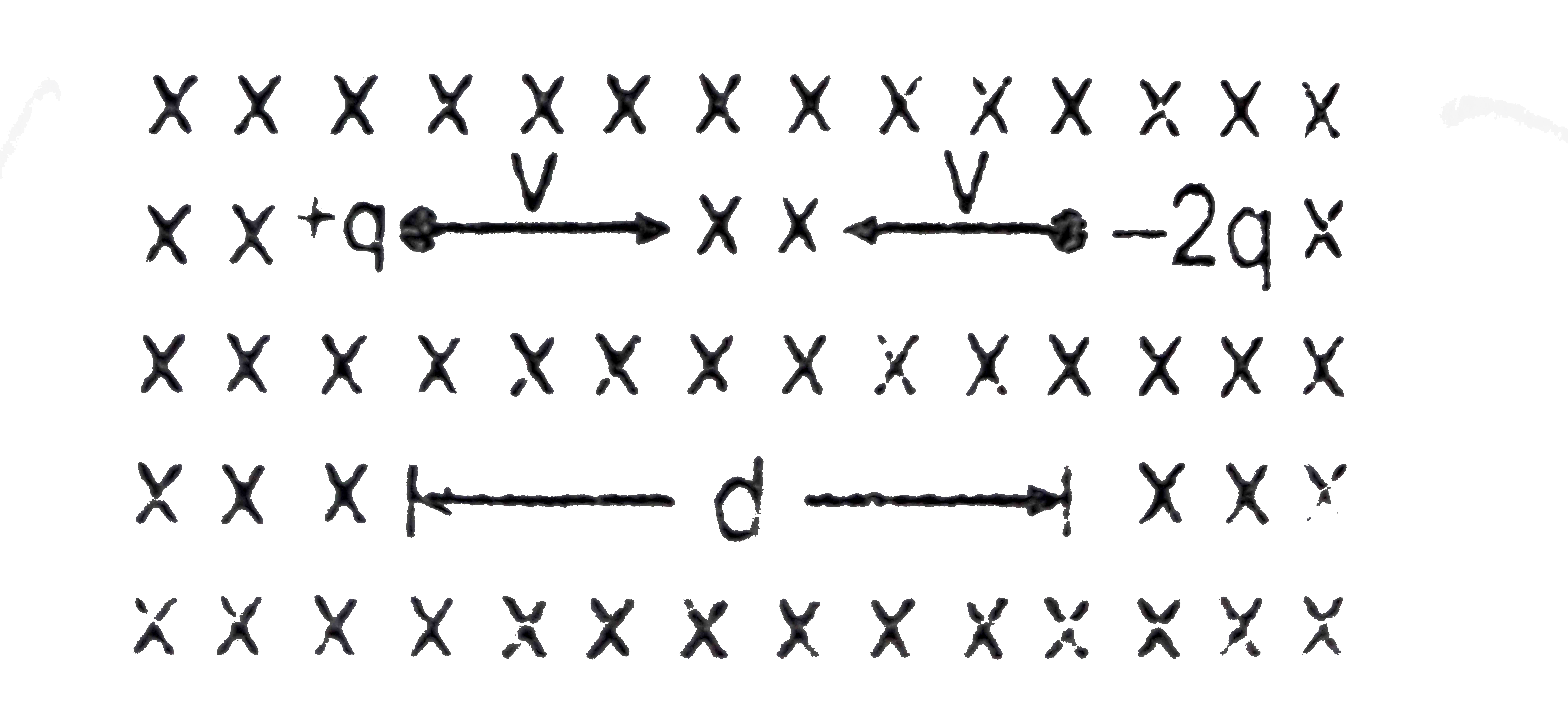
View SolutionA charged particle +q of mass m is placed at a distanced from another charged particle -2q of mass 2m in a uniform magnetic field B as shown in Fig. 1.28. If the particles are projected towards each other with same speed v,

a. find the maximum value of projected speed vm so that the two particles do not collide.
b. find the time after which collision occurs between the particles if projection speed equals 2vm.
c. Assuming the collision to be perfectly inelastic, find the radius of the particle in subsequent motion. (Neglect the electric force between the charges.)
View SolutionA charged particle +q of mass m is placed at a distance d from another charge particle −2q of mass 2m in a uniform magnetic field of induction vectro B as shown in the fig.If the particles are projected towards each other with equal speeds v.(Neglect the interaction between the particles)
(a)Find the maximum value of the projection speed Vmax so that the two particles do not collide.
b)Find the time interval after which collision occurs between the particles if projection speed equals 2Vmax
(c)In the previous part,assuming that the particles stick after the collision find the radius of the circular path of the particle in subsequent motion.
View SolutionTwo points mass m and 2m are kept at a distance a. Find the speed of particles and their relative velocity of approach when separation becomes a/2.
View SolutionA particle P of charge q and m , is placed at a point in gravity free to move. Another particle Q, of same charge and mass , is projected from a distance r from P with an initial speed v0 towards P , initial the distance between P and Q decreases and then increases.
The potential energy of the system of particles P and Q, at closest separation is
View Solution
