A square loop of wire of edge a carries a current i.
Magnetic induction B for a point on the axis of the loop at a distance x=a√2 from its centre is Nμ0i3πa,then find value of NB=4μ0ia2π(4x2+a2)(4x2+2a2)1/2
The correct Answer is:N=2
(a)BR=4Bsinθ
=4×μ0i4πrsinα[cosα+cosα]×sinθ
2μ0iπ[x2+a24]12×a2[x2+a22]12×a2[x2+a22]12
4μ0a2iπ[4x2+a2][4x2+2a2]12
(b)x=0
BR=4μ0a2iπ(a2)(√2a)
=2√2μ0iπa
(c) x>>a
B=4μ0a2iπ4x2(2−x)=μ0a2i2πx3=μ0M2πx3


Topper's Solved these Questions
Similar Questions
A square loop of side a=6cm carries a current I=1A. Calculate magnetic induction B(μT) at point P, lying on the axis of loop and at a distance x=√7cm from the centre of loop.
View SolutionA square loop of wire, edge length a, carries a current i. Compute the magnitude of the magnetic field produced at a point on the axis of the loop at a distance x from the centre.
View Solution
Knowledge Check
A wire in the form of a square of side '2m' carries a current 2A. Then the magentic induction at the centre of the square wire is ( magnetic permeability of free space =μ0)
Aμ02πBμ0√2πC2√2μ0πDμ0√2πA long straight wire carries an electric current of 2A. The magnetic induction at a perpendicular distance of 5m from the wire is (μ04π×107Hm−1)
A4×10−8TB8×10−8TC12×10−8TD16×10−8TThere is one circular loop of wire of radius a, carrying current i. At what distance from its centre on its axis will the magnetic field intensity become 12√2 times at the centre?
AaB2aC3a/2Da/3
A circular loop of radius R carries a current I. Obtain an expression for the magnetic field at a point on its axis at a distance x from its centre.
View SolutionA square loop of wire of edge a carries a current i.
Does the square loop behave like a dipole for points such that x>>a ? If so, what is its dipole moment?
View SolutionA circular loop of 2 turns carries a current of 5⋅0A. If the magnetic field at the centre of loop is 0⋅40mT, find the radius of the loop.
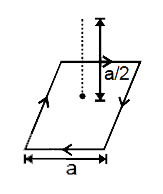
View SolutionThe magnetic field due to a current carrying square loop of side a at a point located symmetrically at a distance of a/2 from its centre (as shown is)
View SolutionA point on line x−y+1=0 at a distance 2√2 from the point (1,2) is (i) (3,4) (ii) (3,0) (iii) (-1,4) (iv) (0,1)
View Solution