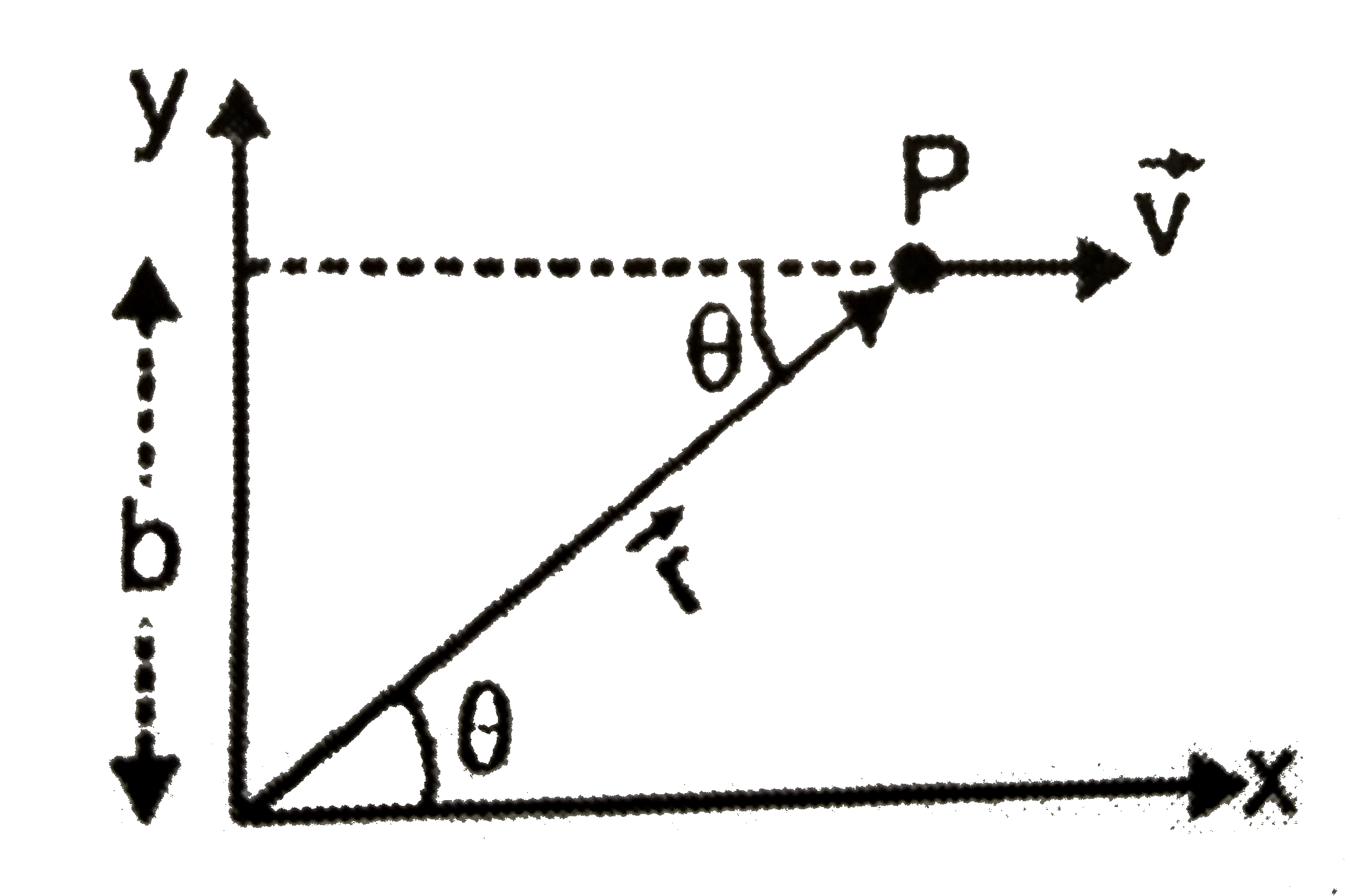
A particle moves parallel to X-axis as shown in the figure such that at all instant the y axis component of its position vector is constant and is equal to 'b'. The angular velocity of the particle about the origin is

vb
The correct Answer is:C
Step by step video, text & image solution for A particle moves parallel to X-axis as shown in the figure such that at all instant the y axis component of its position vector is constant and is equal to 'b'. The angular velocity of the particle about the origin is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- KINEMATICSBook:FIITJEEChapter:KINEMATICSExercise:ASSIGNMENT PROBLEMS (OBJECTIVE) LEVEL-I (ASSERTION REASONING TYPE)
Explore 2 Videos
- KINEMATICSBook:FIITJEEChapter:KINEMATICSExercise:ASSIGNMENT PROBLEMS (OBJECTIVE) LEVEL-II
Explore 20 Videos
- KINEMATICSBook:FIITJEEChapter:KINEMATICSExercise:ASSIGNMENT PROBLEMS (SUBJECTIVE) LEVEL-II & III
Explore 15 Videos
- HEAT AND TEMPERATUREBook:FIITJEEChapter:HEAT AND TEMPERATUREExercise:NUMERICAL BASES QUESTIONS
Explore 1 Video
- LAWS OF MOTIONBook:FIITJEEChapter:LAWS OF MOTIONExercise:COMPREHENSION-III
Explore 2 Videos
Similar Questions
A particle moving parallel to x-axis as shown in fig. such that at all instant the y-axis component of its position vector is constant and is equal to 'b'. Find the angular velocity of the particle about the origin when its radius vector makes angle θ from the axis.
View SolutionA particle is moving in x-y plane as shown in the figure. Angular velocity of the particle with respect to the origin is
View Solution
Knowledge Check
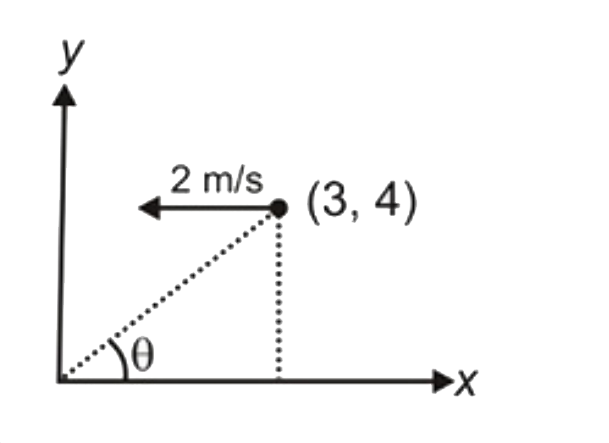
A particle is moving parallel to x-axis as shown in the figure. The angular velocity of the particle about the origin is
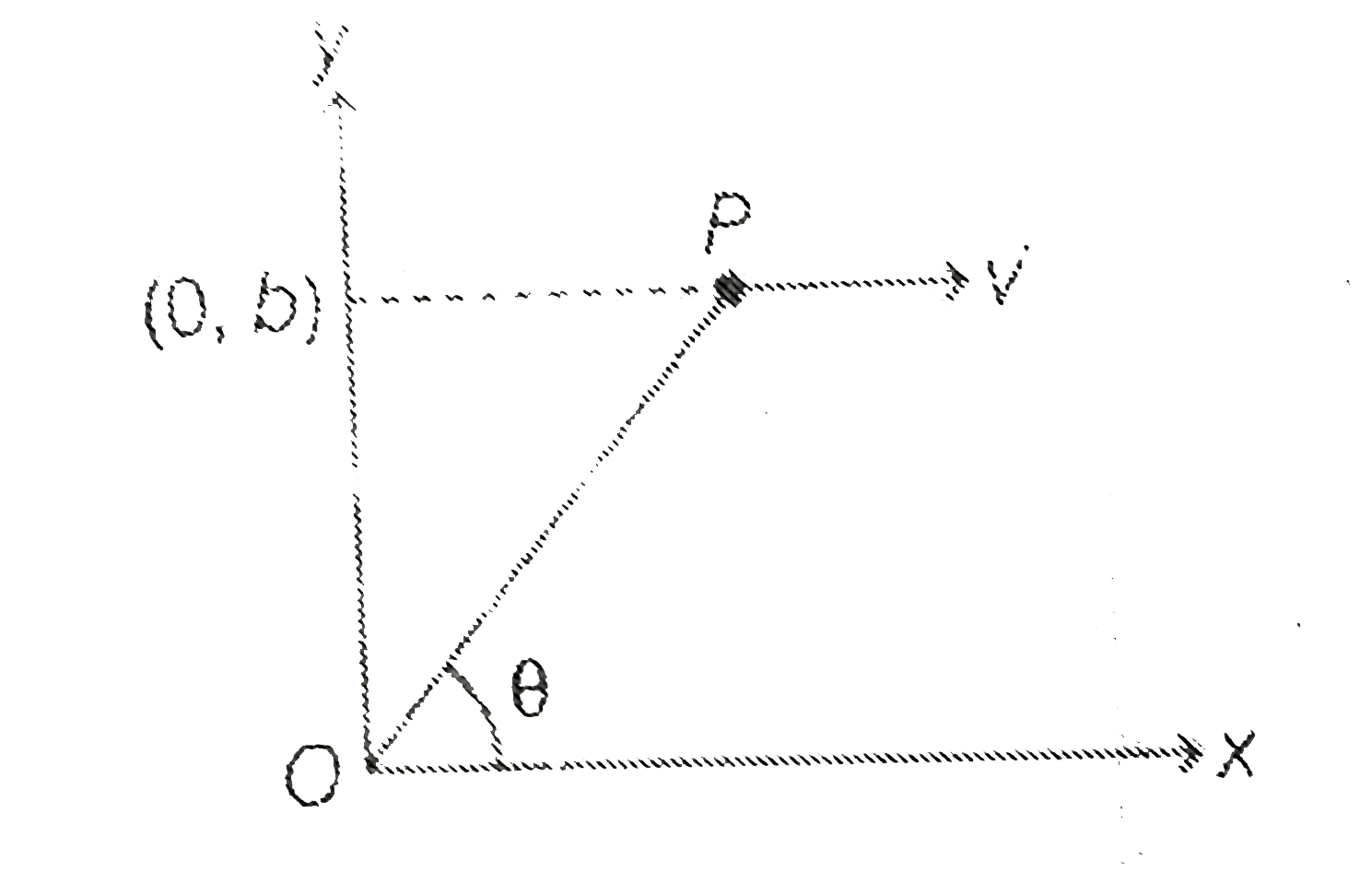 A(v/b)sin2θB(v/b)C(v/b)sinθDvbsinθ
A(v/b)sin2θB(v/b)C(v/b)sinθDvbsinθ
A particle mass parallel to x-axis with constant velocity v as shown in the figure. The angular velocity of the particle about the origin OAremains constantBcontinuously increasesCcontinuously decreasesDoscillates.A particle of mass m moves in the xy-plane with velocity of →v=vxˆi+vyˆj. When its position vector is →r=x→i+y→j, the angular momentum of the particle about the origin is.
Am(xvy+yvx)ˆkB−m(xvy+yvx)ˆkCm(yvx−xvy)ˆkDm(xvy−yvx)ˆk.
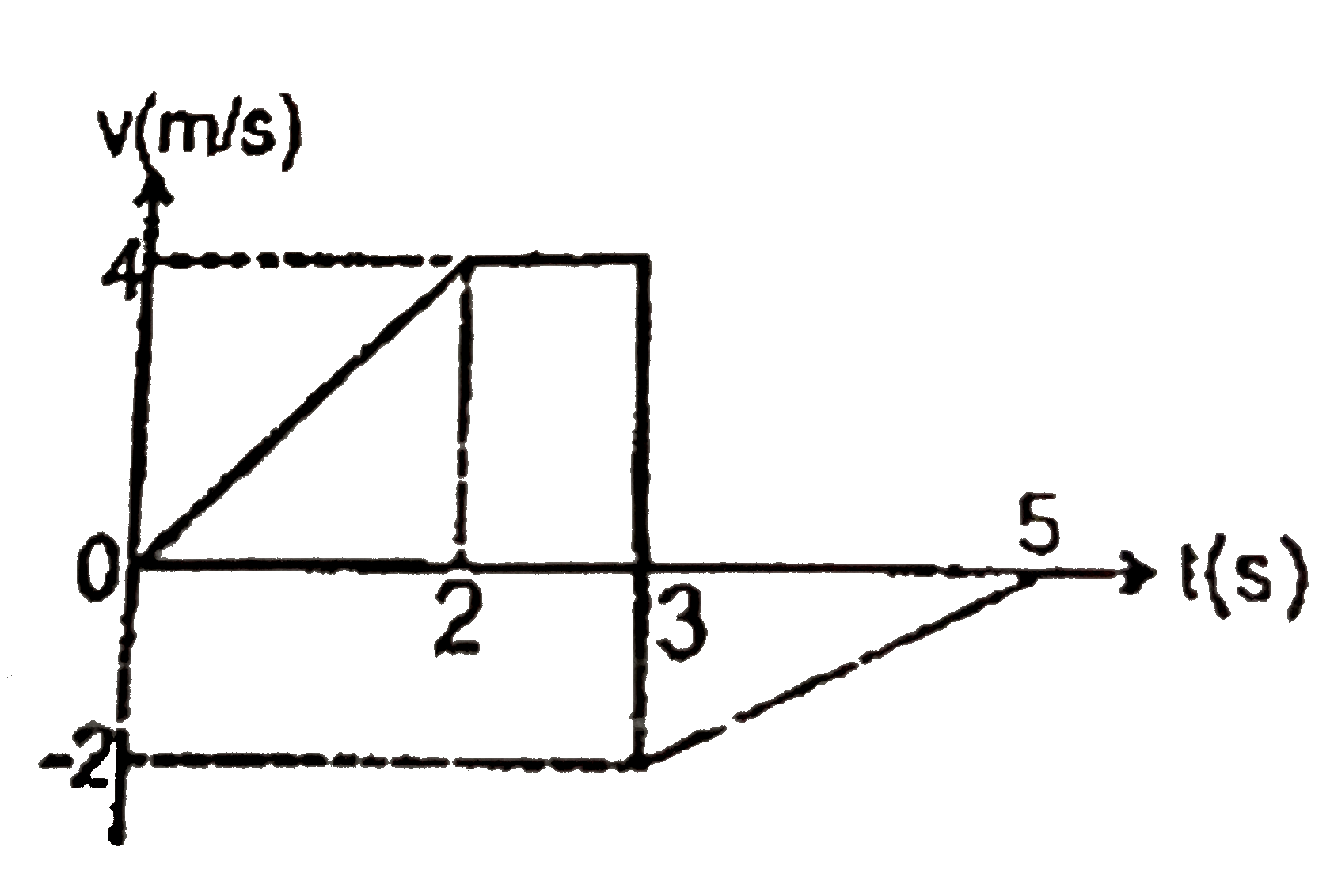
For a particle moving along x-axis, velocity-time graph is as shown in figure. Find the displacement of the particle?
View SolutionA particle of mass m is moving with constant velocity v parallel to the x-axis as shown in the figure. Its angular momentum about origin O is

View SolutionA particle moves in a circle with constant angular velocity ω about a point P on its circumference. The angular velocity of the particle about the centre C of the circle is
View SolutionA particle of mass 0.75 kg is moving in the XY plane parallel to Y-axis, with a uniform speed of 4 m/s. It crosses the X-axis at 3 m from the origin. What is the angular momentum of the particle about the origin?
View SolutionA particle has a linear momentum p and position vector r. the angular momentum of this particle about the origin will not be zero under the conditions
View Solution