Find the centre of mass of a uniform :
(a) half-disc, (b) quarter-disc.

A

$(\frac{4 R}{3 π}, \frac{3 R}{3 π})$ , $(\frac{4 R}{3 π}, \frac{3 R}{3 π})$

B

$(0, \frac{4 R}{3 π})$ , $(\frac{4 R}{3 π}, \frac{3 R}{3 π})$

C

$(0, \frac{4 R}{3 π})$ , $(\frac{4 R}{3 π}, \frac{4 R}{3 π})$

D

$(\frac{2 R}{2 π}, \frac{4 R}{3 π})$ , $(\frac{4 R}{3 π}, \frac{4 R}{3 π})$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

Let M and R be the mass and radius of the half - disc mass per unit area unit aera of the half - disc
$m = \frac{M}{\frac{1}{2} π R^{2}} = \frac{2 M}{π R^{2}}$

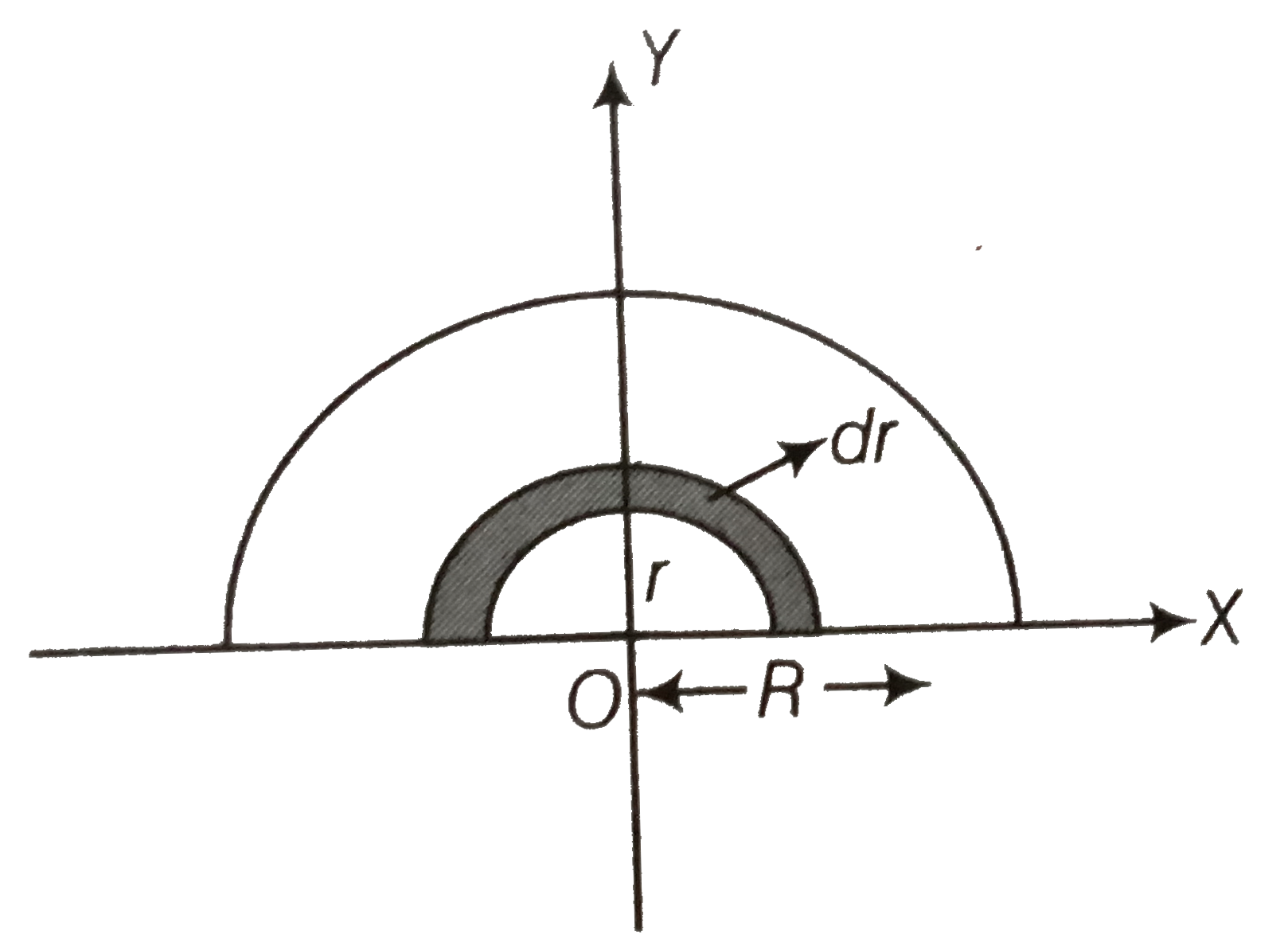
(a) the half -disc can be supposed to be consists of a large number of semicircular rings of mass dm and thickness dr and ranging from r=0 to r=R
Surface area of semicircular ring of radius r and of thickness $d r = \frac{1}{2} 2 π r \times d r = π r d r$
$∴$ Mass of this elemetary ring $d m = π r d r \times \frac{2 M}{π R^{2}}$
$d m = \frac{2 M}{R^{2}} r d r$
If (x,y) are coordinates of centre of mass of this element,
then, $(x, y) = (0, \frac{2 r}{π})$
therefore , $x = 0 and y = \frac{2 r}{π}$
Let $x_{c M}$ and $y_{C M}$ be the coordinates of the centre of the centre of the semicircular disc.
then $x_{C M} = \frac{1}{M} \int_{0}^{R} x d m = \frac{1}{M} \int_{0}^{R} d m = 0$
$y_{C M} = \frac{1}{M} \int_{0}^{R} y d m = \frac{1}{M} \int_{0}^{R} \frac{2 l}{π} \times (\frac{2 M}{R^{2}} r d r)$
$= \frac{4}{π R^{2}} \int_{0}^{R} r^{2} d r = \frac{4}{π R^{2}} {[\frac{r^{3}}{3}]}_{0}^{R}$
$= \frac{4}{π R^{2}} \times (\frac{R^{3}}{3} - 0) = \frac{4 R}{3 π}$
$∴$ Centre of mass of the semicicular disc $= (9, \frac{4 R}{3 π})$
( b) Centre of mass a unifrom quarter disc,
Mass per unit area of the quarter disc $= \frac{M}{\frac{π R^{2}}{4}} = \frac{4 M}{3 Π R^{3}}$
Using symmetry
for a half along y - axis centre of mass will be at $x = \frac{4 R}{3 π}$
For a half - disc along x-axis centre of mass will be at $x = \frac{$ R}{3 π}$
Hence , for the quarter disc centre of mass $= (\frac{4 R}{3 π}, \frac{3 R}{3 π})$

Updated on:21/07/2023

|

Class 11 PHYSICS RIGID BODY DYNAMICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

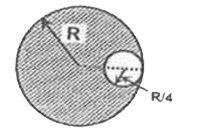
Find the position of centre of mass of a uniform disc of radius R from which a hole of radius is cut out. The centre of the hole is at a distance R/2 from the centre of the disc.

A $\frac{R r^{2}}{2 (R^{2} - r^{2})}$ towards right of O
B $\frac{R r^{2}}{2 (R^{2} - r^{2})}$ towards left of O
C $\frac{2 R r^{2}}{(R^{2} + r^{2})}$ towards right of O
D $\frac{2 R r^{2}}{(R^{2} + r^{2})}$ towards left of O
The acceleration of the centre of mass of a uniform solid disc rolling down an inclined plane of angle $θ$ is
A $\frac{1}{3} g sin θ$
B $\frac{2}{3} g sin θ$
C $g sin θ$
D $\frac{1}{4} g sin θ$

NCERT EXEMPLAR-SYSTEM OF PARTICLES AND ROTATIONAL MOTION -Long answer type questions

Home
Ask Doubt
Profile