If a ladder is not in balance against a smooth vertical wall, then it can be made in balance by :-
Decreasing the length of ladder
Increasing the length of ladder
Increasing the angle of inclination
Decreasing the angle of inclination
The correct Answer is:C
To solve the problem of balancing a ladder against a smooth vertical wall, we can analyze the forces acting on the ladder and how they relate to its angle of inclination. Here’s a step-by-step solution:
Step 1: Understand the Forces Acting on the Ladder
The ladder experiences a gravitational force acting downwards due to its weight (mg), where m is the mass of the ladder and g is the acceleration due to gravity. This force acts at the center of mass of the ladder.
Hint: Identify the forces acting on the ladder and their directions.
Step 2: Identify the Role of the Angle of Inclination
The angle of inclination (θ) is the angle between the ladder and the ground. The gravitational force can be resolved into two components: one perpendicular to the ground (mg cos θ) and one parallel to the ground (mg sin θ).
Hint: Consider how the angle of inclination affects the components of the gravitational force.
Step 3: Analyze the Conditions for Balance
For the ladder to be in balance, the net torque about any point (usually the base of the ladder) must be zero. If the angle of inclination is too steep or too shallow, the ladder will either slide down or fall over.
Hint: Think about how changing the angle of inclination affects the stability of the ladder.
Step 4: Evaluate the Options Given
1. Decreasing the length of the ladder: This does not affect the gravitational force acting on the ladder and will not help in balancing it.
2. Increasing the length of the ladder: Similar to decreasing the length, this will not change the gravitational force and will not help in balancing.
3. Increasing the angle of inclination: This decreases the component of the gravitational force acting parallel to the ground (mg sin θ), which can help in balancing the ladder.
4. Decreasing the angle of inclination: This increases the component of the gravitational force acting parallel to the ground, making it less stable.
Hint: Analyze each option to see how it affects the balance of the ladder.
Step 5: Conclusion
The correct option to make the ladder balance against the smooth vertical wall is to increase the angle of inclination. This reduces the component of the gravitational force that could cause the ladder to slide down.
Final Answer: Increasing the angle of inclination (Option C) will help balance the ladder.
The ladder experiences a gravitational force acting downwards due to its weight (mg), where m is the mass of the ladder and g is the acceleration due to gravity. This force acts at the center of mass of the ladder.
The angle of inclination (θ) is the angle between the ladder and the ground. The gravitational force can be resolved into two components: one perpendicular to the ground (mg cos θ) and one parallel to the ground (mg sin θ).
For the ladder to be in balance, the net torque about any point (usually the base of the ladder) must be zero. If the angle of inclination is too steep or too shallow, the ladder will either slide down or fall over.
1. Decreasing the length of the ladder: This does not affect the gravitational force acting on the ladder and will not help in balancing it.
2. Increasing the length of the ladder: Similar to decreasing the length, this will not change the gravitational force and will not help in balancing.
3. Increasing the angle of inclination: This decreases the component of the gravitational force acting parallel to the ground (mg sin θ), which can help in balancing the ladder.
4. Decreasing the angle of inclination: This increases the component of the gravitational force acting parallel to the ground, making it less stable.
The correct option to make the ladder balance against the smooth vertical wall is to increase the angle of inclination. This reduces the component of the gravitational force that could cause the ladder to slide down.
Topper's Solved these Questions
- NEWTONS LAWS OF MOTIONBook:ALLENChapter:NEWTONS LAWS OF MOTIONExercise:EXERCISE-III
Explore 28 Videos
- NEWTONS LAWS OF MOTIONBook:ALLENChapter:NEWTONS LAWS OF MOTIONExercise:EXERCISE-I
Explore 52 Videos
- NEWTON'S LAWS OF MOTION & FRICTIONBook:ALLENChapter:NEWTON'S LAWS OF MOTION & FRICTIONExercise:EXERCISE (JA)
Explore 4 Videos
- RACEBook:ALLENChapter:RACEExercise:Basic Maths (Wave Motion & Dopplers Effect) (Stationary waves & doppler effect, beats)
Explore 25 Videos
Similar Questions
If a ladder which is 10m long rests against a vertical wall. If the base of ladder slides away from the wall at 1 m/s then how fast is the top of the ladder sliding down along the wall when the base is 6 m from the wall ?
View SolutionA uniform ladder of length 3.25 m and weight 250 N is placed against a smooth vertical wall with its lower end 1.25 m from the wall. If coefficient of friction between the ladder and the floor is 0.3, then, find the frictional force acting on the ladder at the point of contact between ladder and floor.
View Solution
Knowledge Check
A ladder of length l and mass m is placed against a smooth vertical wall, but the ground is not smooth. Coefficient of friction between the ground and the ladder is μ. The angle θ at which the ladder will stay in equilibrium is
Aθ=tan−1(μ)Bθ=tan−1(2μ)Cθ=tan−1(μ2)Dnone of theseA ladder wighing 300 N is placed against a smooth vertical wall having a coefficient of friction between it and the floor of 0.2. What is the maximum force of friction available at the point of contact between the ladder and the floor?
A40 NB50 NC60 ND70 NA ladder, 10 metre long rests with one end against a smooth vertical wall and the other end on the ground which is rough, the coefficient of friction being 12. The foot of the ladder being 2 metre from the wall. A amn whose weight is 4 times that of the ladder can ascend before it be givens to slip is
A34(10√6−1)B54(10√6−1)C23(5√2−1)DNone
A uniform ladder of mass 10 kg leans against a smooth vertical wall making an angle of 530 with it. The other end rests on a rough horizontal floor. Find the normal force and the frictional force that the floor exerts on the ladder
View SolutionA uniform ladder of length l rests against a smooth, vertical wall (figure). If the mass of the ladder is m and the the coefficient of static friction between the ladder and the ground is μs=0.375 and the minimum angle θmin at which the ladder does not slip.
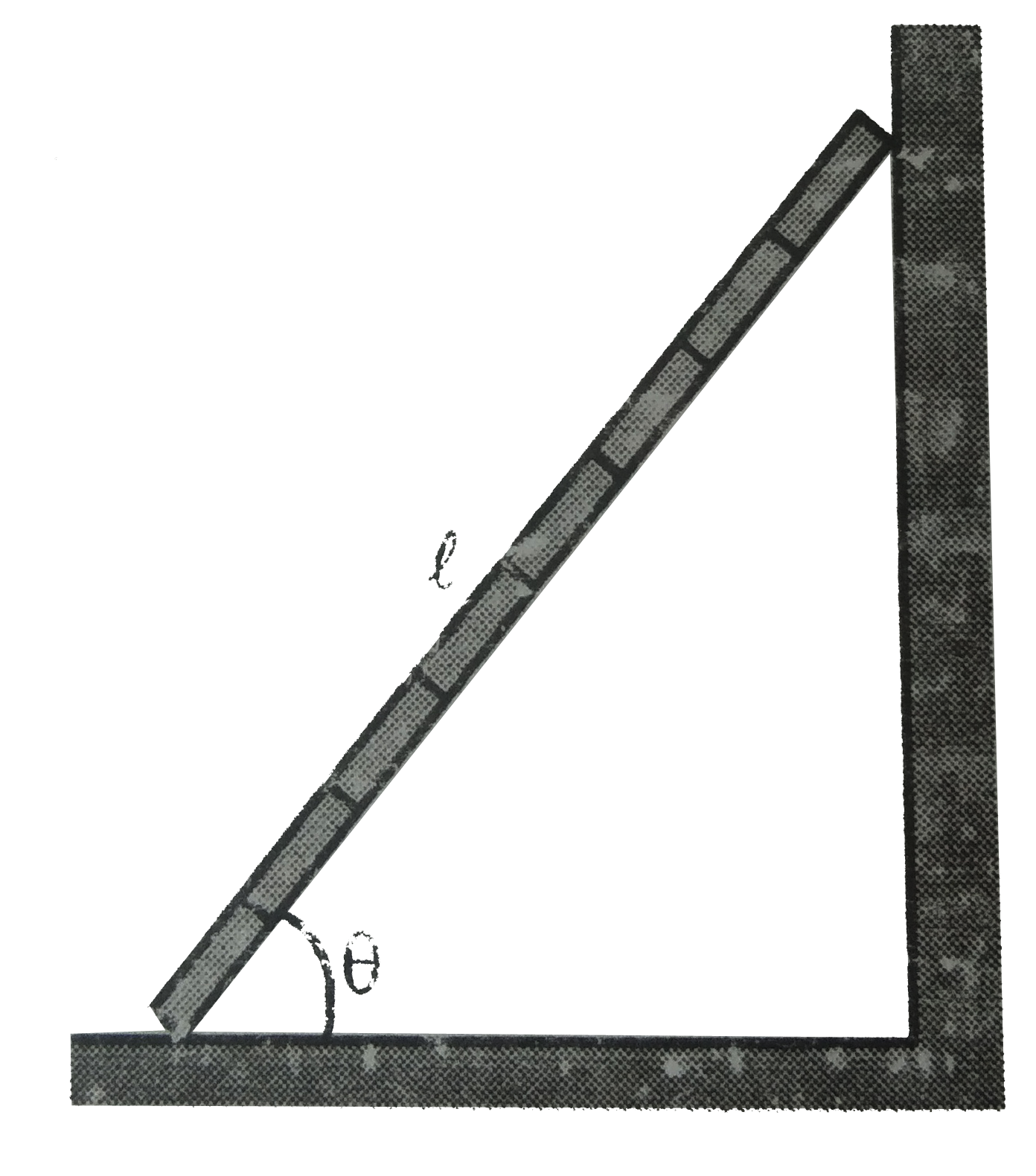
A uniform ladder at rest, leading against a smooth wall. Th ground is rough.
View SolutionA uniform ladder rests in limiting equilibrium, its lower end on a rough horizontal plae and its upper end against a smooth vertical wall. If θ is the engle of inclination of the ladder to the vertical wall and μ is the coefficient of friction then tanθ is equal to
View SolutionA uniform ladder rests in limiting equilibrium with its lower end on a rough horizontal plane with coefficient of friction μ and its upper end against a smooth vertical wall. If θ is the inclination of the ladder with the wall, then θ is equal to
View SolutionA uniform ladder rests in limiting equilibrium with its lower end on a rough horizontal plane with coefficient of friction μ and its upper end against a smooth vertical wall. If θ is the inclination of the ladder with the wall, then θ is equal to
View Solution