A quadrilateral ABCD is such that diagonal BD divides its area in two equal parts. Prove that BD bisects AC. GIVEN : A quadrilateral ABCD in which diagonal BD bisects it. i.e. ar(ABD)=ar(BDC) CONSTRUCTION : Join AC Suppose AC and BD intersect at O . Draw AL⊥BD and CMBD. TO PROVE : AO=OC.
Given: BD is the diagonal of quadrilateral ABCD, which divide it into two equal areas.∴ar(ADB)=ar(DCB)
From A and C draw perpendiculars to the diagonal BD.
AOE=COF (Vertical opposite angles)
In △AOE and △COF,
∠AEO=∠CFO (Both are 90∘)
AE=CF (Bases and areas of both the △ADB and △BDC are same.)
EAO=FCO (∠AEO=∠CFO=90° and ∠AOE=∠COF)
tr∈ag≤AOE≅△COF ( By ASA)
AO=OC (by CPCT)
Thus, BD bisect AC at O.
Hence Proved.
Topper's Solved these Questions
Similar Questions
In Figure, ABCD is a parallelogram. Prove that: ar(BCP)=ar(DPQ) CONSTRUCTION : Join AC.
View SolutionA diagonal of a parallelogram divides it into two triangles of equal area. GIVEN : A parallelogram ABCD in which BD is one of the diagonals. TO PROVE : ar (ABD)=ar(CDB)
View SolutionProve that the diagonals of quadrilateral ABCD,AC and BD intersect in O. Prove that if BO=OD, the triangles ABC and ADC are equal in area.
View SolutionShow that a median of a triangle divides it into two triangles of equal area. GIVEN : A ABC in which AD is the median. TO PROVE : ar(ABD)=ar(ADC) CONSTRUCTION : Draw AL⊥BC
View SolutionShow that the diagonals of a parallelogram divide it into four triangles of equal area. GIVEN : A parallelogram ABCD . The diagonals AC and BD intersect at O. TO PROVE : ar(OAB)=ar(OBC)=ar(OCD)=ar(AOD)
View SolutionIn Figure, ABCD is a trapezium in which AB∣∣CD . Prove that: ar(AOD)=ar(BOC).
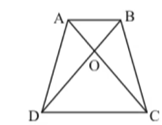
View SolutionDiagonals AC and BD of a quadrilateral ABCD intersect at O in such a way that ar(AOD)=ar(BOC) . Prove that ABCD is a trapezium.
View SolutionIf the diagonals AC,BD of a quadrilateral ABCD, intersect at O, and seqarate the quadrilateral into four triangles of equal area, show that quadrilateral ABCD is a parallelogram. GIVEN : A quadrilateral ABCD such that its diagonals AC and BD intersect at O and separate it into four parts such that ar(AOB)=ar(BOC)=ar(COD)=ar(AOD) TO PROVE : Quadrilateral ABCD is a parallelogram.
View SolutionDiagonals AC and BD of a quadrilateral ABCD intersect at O in such a way that ar (AOD)=ar ( BOC). Prove that ABCD is a trapezium.
View SolutionProve that If each diagonal of a quadrilateral separates it into two triangles of equal area then show that the quadrilateral is a parallelogram.
View Solution