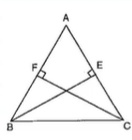
ABC is a triangle is which BE and CF are, respectively, the perpendiculars to the sides AC and AB . If BE=CF , prove that ABC is isosceles.
In order to prove that the △ABC is isosceles, we will prove that ∠B=∠C.
To prove this, we will prove that △BFC≅△CEB.
In these two triangles, we have BE=CF [Given]
BC=BC [common]
So, by RHS congruence criterion, we obtain △BFC≅△CEB
⇒∠FBC=∠ECB
⇒∠ABC=∠ACB
⇒AB=AC [since Sides opposite to equal angles are equal]
So, △ABC is isosceles.
Hence Proved.
Topper's Solved these Questions
Similar Questions
In Figure, BD and CE are two altitudes of a ABC such that BD=CE. Prove that ABC is isosceles.
View SolutionThe bisectors of the angles B and C of a triangle ABC , meet the opposite sides in D and E respectively. If DE||BC , prove that the triangle is isosceles.
View SolutionIn Figure, it is given that AB=BC and AD=EC . Prove that ABE≅CBD

View SolutionIf O is a point in space, ABC is a triangle and D,E,F are the mid-points of the sides BC,CA and AB respectively of the triangle, prove that →OA + →OB+→OC=→OD+→OE+→OF.
View SolutionIn Figure, ABC is an isosceles triangle in which AB=AC.CPparel≤lAB and AP is the bisector of exterior ∠CAD of ABC . Prove that ∠PAC=∠BCA and (ii) ABCP is a parallelogram.
View SolutionIn ΔABC, the incircle touches the sides BC,CA and AB respectively at D,E and F. If the radius of the incircle is 4 units and BD,CE and AF are consecutive integers, then find the perimeter of the A B C.
View SolutionE,F,G,H are respectively, the mid-points of the sides AB,BC,CD and DA of parallelogram ABCD . Show that the quadrilateral EFGH is a parallelogram and that its area is half the area of the parallelogram, ABCD. GIVEN : A quadrilateral ABCD in which L,F,G,H are respectively the mid-points of the sides AB,BC,CD and DA. TO PROVE : (i) Quadrilateral EFGH is a parallelogram ar(^(gm)EFGH)=12ar(^(gm)ABCD) CONSTRUCTION : Join AC and HF
View SolutionD and E are points on sides AB and AC respectively of ΔABCsuch thatar (DBC) = ar (EBC). Prove that DE ∣∣ BC.
View SolutionIn ΔABC, D, E and F are respectively the mid-points of sides AB, BC and CA. Show that ΔABCis divided into four congruent triangles by joining D, E and F.
View SolutionIf in a ΔABC,c(a+b)cosB/2=b(a+c)cosC/2, prove that the triangle is isosceles.
View Solution
