A triangle ABC is right angled at A.AL is drawn perpendicular to BC . Prove that ∠BAL=∠ACB.
In traingle BAC and traingle BLC
∠B=∠B(common)
∠BLA=∠BAC(90∘)
andBA=BA(common side)
so from AAS RULE △BAC≅△BLA
from CPCT ,
∠BAC=∠ACB
Topper's Solved these Questions
Similar Questions
Knowledge Check
ABC is a triangle right angled at B. The hypotenuse (AC) is four times the perpendicular (BD) drawn to it from the opposite vertex and AD<DC.
What is tan(A-C) equal to?AA. 0BB. 1CC. 2DD. none
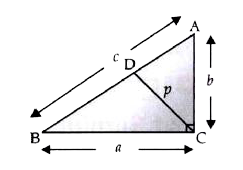
ABC is a right triangles right angled at C . Let BC =a ,CA=b, AB = c, and p be the length of perpendicular from C to AB . Prove that cp = ab .
View SolutionIn a △ABC , right angled at C and ∠A=∠B , is cosA=cosB ?
View SolutionABC is right triangle right angled at B.If BD is the length of the perpendicular drawn from B to AC then
View SolutionIn fig.41, ABC is a right triangle right angled at A. D lies on BA produced and DE⊥BC, intersecting AC at F . if ∠AFE=1300, find ∠BDE (ii) ∠BCA ∠ABC
View SolutionIf ABC is an isosceles triangle such that AB=AC and AD is an altitude from A on BC . Prove that (i) ∠B=∠C (ii) AD bisects BC (iii) AD bisects ∠A
View SolutionABC is a triangle right-angled at C. If p is the length of the perpendicular from C to AB and a, b, c are the sides, then which one of the following is correct ?
View SolutionIn right triangle ABC , right angle at C, M is the mid-point of the hypotenuse AB. C is jointed to M and produced to a point D such that DM=CM. Point D is joined to point B. Show that AMC≅BMD (ii) ∠DBC=90∘ (iii)DBC≅ACB (iv) CM=12AB
View Solution