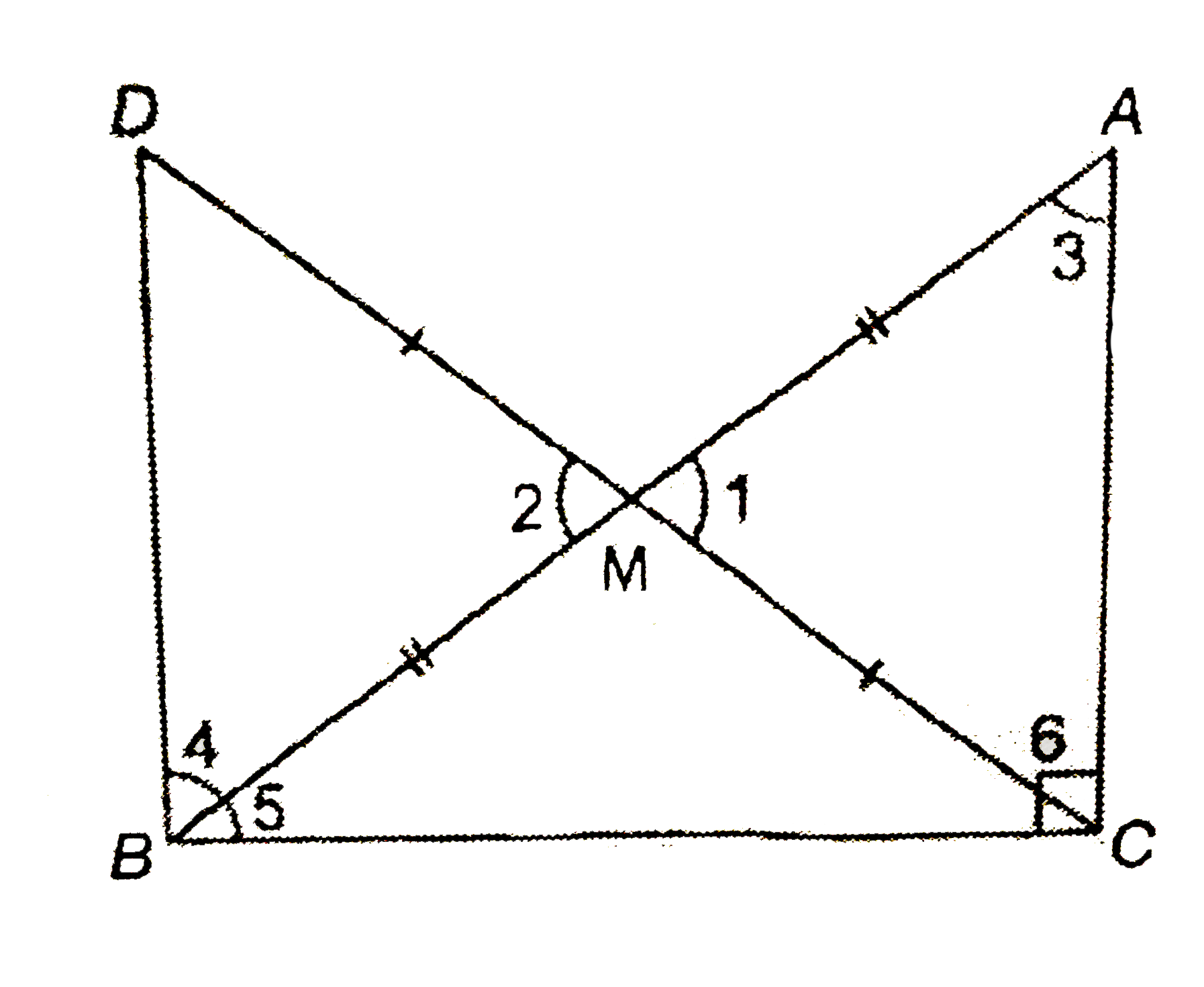
In right triangle ABC , right angle at C, M is the mid-point of the hypotenuse AB. C is jointed to M and produced to a point D such that DM=CM. Point D is joined to point B. Show that AMC≅BMD (ii) ∠DBC=90∘ (iii)DBC≅ACB (iv) CM=12AB
Given: M is the mid-point of hypotenuse AB, ∠C=90° and DM=CM
To Prove:
(i) △AMC≅△BMD
(ii) ∠DBC is a right angle.
(iii) △DBC≅△ACB
(iv) CM=12AB
Proof:
(i) In △AMC and △BMD,
AM=BM (M is the mid - point of AB)
∠AMC=∠BMD (Vertically opposite angles)
CM=DM (Given)
∴△AMC≅△BMD (By SAS congruence rule)
∴AC=BD (By CPCT)
Also, ∠ACM=∠BDM (By CPCT)
(ii) ∠DBC is a right angle.
∠ACM=∠BDM (proved above)
But, ∠ACM and ∠BDM are alternate interior angles. Since alternate angles are equal, it can be said that DB∣∣AC.
∠DBC+∠ACB=180° (Co-interior angles)
∠DBC+90°=180° [Since, △ACB is a right angled triangle]
∴△DBC=90°
Thus, ∠DBC is a right angle.
(iii) In △DBC and △ACB,
DB=AC (proved above)
∠DBC=∠ACB=90° (Proved above)
BC=CB (Common)
∴△DB≅△ACB (By SAS congruence rule)
(iv) CM=12AB
Since △DBC≅△ACB
AB=DC (By CPCT)
⇒12AB=12DC
CM=12DC=12AB
∴CM=12AB
Hence proved.
Topper's Solved these Questions
Similar Questions
In right triangle ABC , right angle at C, M is the mid-point of the hypotenuse AB. C is jointed to M and produced to a point D such that DM=CM. Point D is joined to point B. Show that AMC≅BMD (ii) ∠DBC=90∘ (iii)DBC≅ACB (iv) CM=12AB
View SolutionIn right triangle ABC, right angled at C, Mis the mid-point of hypotenuse AB. C is joined to M and produced to a point D such that DM = CM. Point D is joined to point B (see figure). Show that

∠DBC is right angle.
View Solution
Knowledge Check
In right triangle ABC, right angle at C, M is the mid-point of the hydrotenuse AB. C is joined to M and produced to a point D such that DM=CM. Point D is joined to point B. Show that
(i) ΔAMC≅ΔBMD (ii) ∠DBC=∠ACB
(iii) ΔDBC≅ΔACB (iv) CM=12AB
 AASABRHSCSSSDSAS
AASABRHSCSSSDSAS
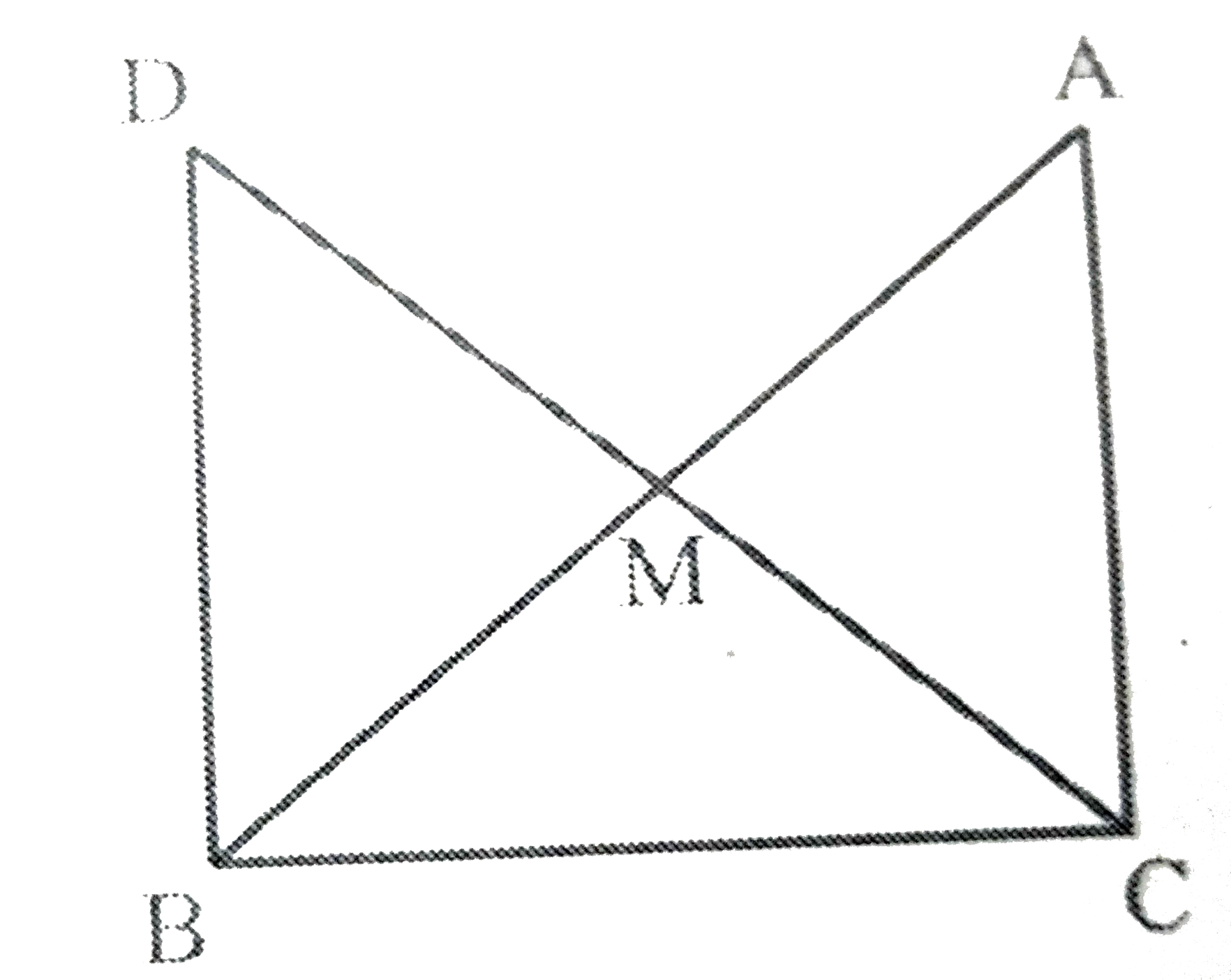
In right triangle ABC, right angled at C, Mis the mid-point of hypotenuse AB. C is joined to M and produced to a point D such that DM = CM. Point D is joined to point B (see figure). Show that

ΔAMC≅ΔBMD
View SolutionIn right triangle ABC, right angled at C, Mis the mid-point of hypotenuse AB. C is joined to M and produced to a point D such that DM = CM. Point D is joined to point B (see figure). Show that

ΔDBC≅ΔACB
View SolutionIn right triangle ABC, right-angled at C, M is the mid-point of hypotenuse AB. C is joined to M and produced to a point D such that DM = CM. Point D is joined to point B (see Fig. 7.23). Show that:
(i) ΔAMC≅ΔBMD
(ii) ∠DBC is a right angle
(iii) ΔDBC≅ΔACB
(iv) CM=12AB
View SolutionIn right triangle ABC, right angle at C, M is the mid-point of the hydrotenuse AB. C is joined to M and produced to a point D such that DM=CM. Point D is joined to point B. Show that
(i) ΔAMC≅ΔBMD (ii) ∠DBC=∠ACB
(iii) ΔDBC≅ΔACB (iv) CM=12AB

View SolutionABC is a triangle right-angled at C. A line through the mid-point M of hypotenuse AB and parallel to BC intersects AC at D. Show that
(i) D is the mid-point of AC
(ii) MD⊥AC
(iii) CM = MA=12AB
View SolutionIn Figure, triangle ABC is right-angled at B. Give that AB=9CM, AC=15CM,andD,E are the mid-points of the sides ABandAC respectively, calculate
The length of BC
(ii) The area of ADE
View SolutionTriangle ABC is right angled at A.AD is perpendicular to BC. If AB=5 cm, BC=13 cm and AC=12 cm. Find the area of ABC. Also, find the length of AD.
View Solution