A weightless rod of length l with a small load of mass m at the end is hinged at point A (Fig.) and occupies a strictly vertical position, touching a body of mass M.
A light jerk sets the system in motion . For what mass ratio M/m will the rod form an angle α=π/6 with the horizontal at the moment of the separation from the body ? What will be the velocity u of the body at this moment ? Friction should be neglected.

Step by step video & image solution for A weightless rod of length l with a small load of mass m at the end is hinged at point A (Fig.) and occupies a strictly vertical position, touching a body of mass M. A light jerk sets the system in motion . For what mass ratio M//m will the rod form an angle alpha = pi//6 with the horizontal at the moment of the separation from the body ? What will be the velocity u of the body at this moment ? Friction should be neglected. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
A weightless rod of length l with a small load of mass m at the end is hinged at point A as shown and occupies a strictly vertical position, touching a body of mass M. A light jerk sets the system in motion.
a. For what mass ratio M/m will the rod form an angle α=π/6 with the horizontal at the moment of the separation from the body?
b. What will he the velocity u of the body at this moment? Friction should be neglected.
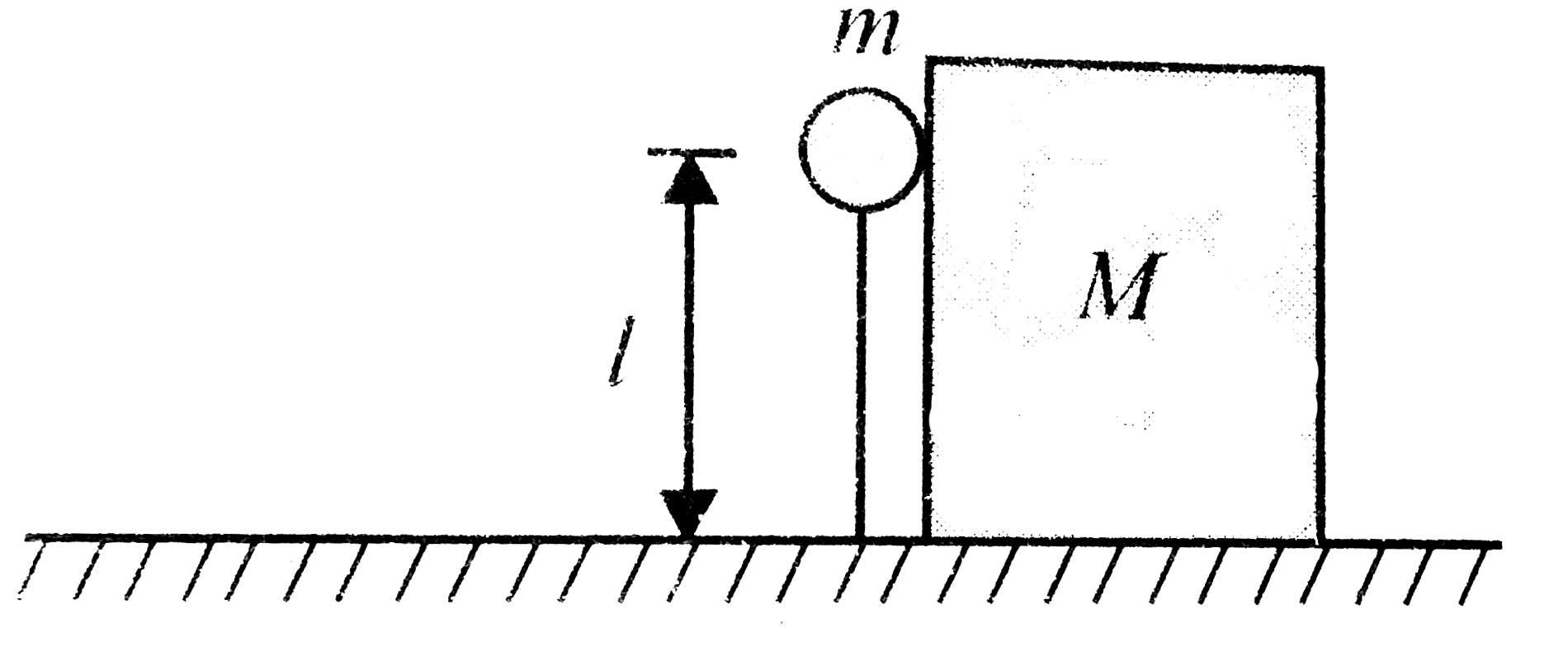
View SolutionA weightless rod of length l with a small load of mass m at one of its end is held vertical with its lower end hinged on a horizontal surface. The load touches a wedge of mass M in this position. A slight jerk towards right sets the system in motion (see figure), with rod rotating freely in vertical plane about its lower end. There is no friction.
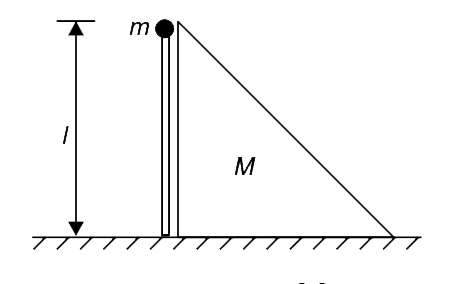
(a) For what mass ratio Mm will the rod form an angleθ=π/3 with the vertical at the moment the load separates from the wedge? (b) What is speed of the wedge at that moment? Neglect friction.
View Solution
Knowledge Check
There is a rod of length l and mass m. It is hinged at one end to the ceiling. The period of small oscillation is
AT=2π√2l3gBT=π√l3gCT=2π√l3gDT=2π√lgA rod of mass m and length l is hinged at 'its' end and released from rest in position shown. Find the force exerted by the hinge when the rod becomes horizontal
 Amg4B3mg2cosθCmg4√9cos2θ+14Dmg2√9cos2θ+14
Amg4B3mg2cosθCmg4√9cos2θ+14Dmg2√9cos2θ+14A rod of mass m and length / is made to stand at an angle of 60∘ with the vertical. Potential energy of the rod in this position is
AmglBmgl2Cmgl3Dmgl4
A uniform rod of mass M and length L is hinged at its end. The rod is released from its vertical position by slightly pushing it. What is the reaction at the hinge when the rod becomes horizontal, again vertical.
View SolutionA weightless rigid rod with a load at the end is hinged at point A to the wall so that it can rotate in all directions (Fig).
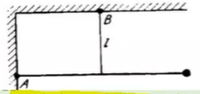
The rod is kept in the horizontal position by a vertical inextensible thread of length 1, fixed at its midpoint. The load receives a momentum in the direction perpendicular to the plane of the figure.
Determine the period T of small oscillations of the system.
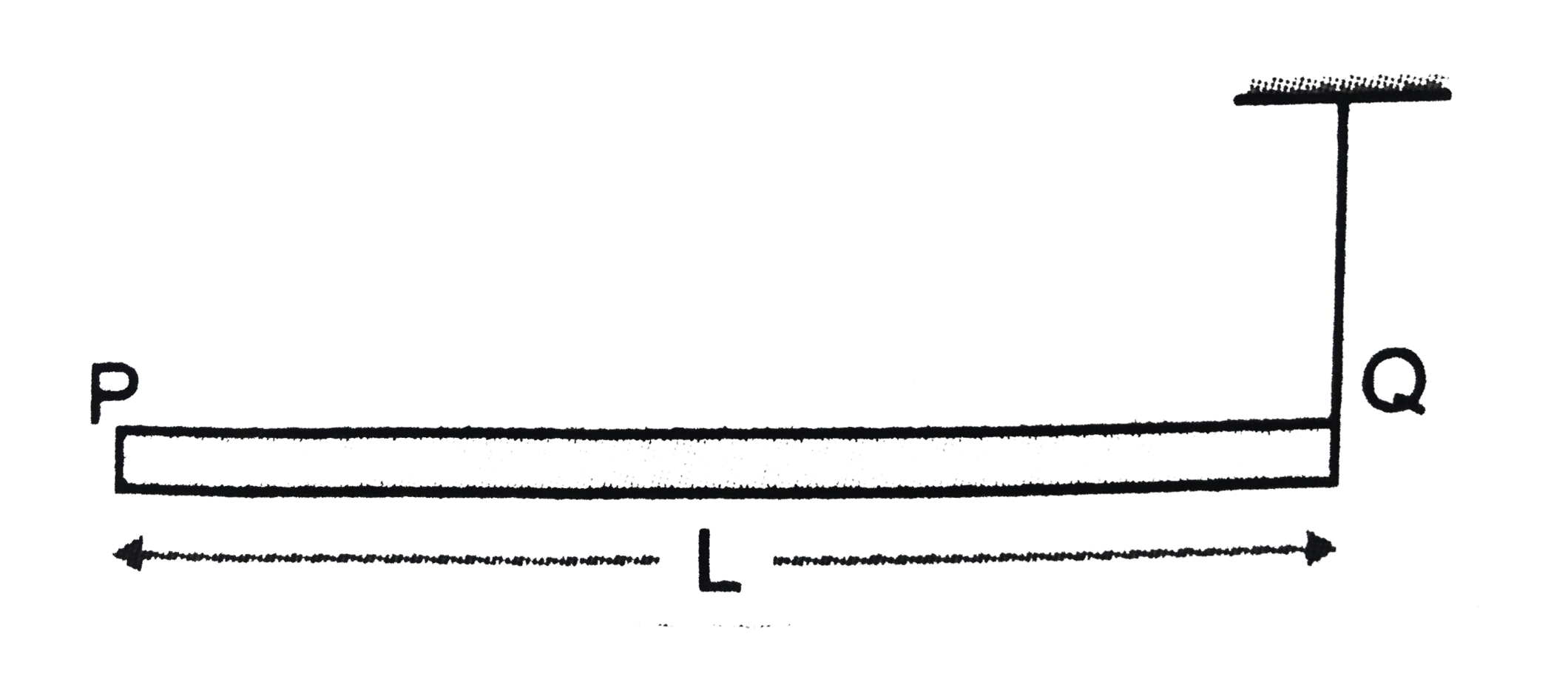
View SolutionA rod PQ of mass M and length L is hinged at end P. The rod is kept horizontal by a masseless string tied to point Q as shown in Fig. When string is cut, the initial angular acceleration of the rod is :
View SolutionA uniform thin rod of length l and mass m is hinged at a distance l/4 from one of the end and released from horizontal position as shown in Fig. The angular velocity of the rod as it passes the vertical position is

View SolutionA uniform thin rod of length l and mass m is hinged at a distance l/4 from one of the end and released from horizontal position as shown in Fig. The angular velocity of the rod as it passes the vertical position is

View Solution