Several identical balls are at rest in a smooth stationary horizontal circular pipe. One of the balls explodes, distintegrating into two fragments of different masses. Determine the final velocity of the body formed as a result of all collisions, assuming that the collisions are perfectly inelastic.
Step by step video & image solution for Several identical balls are at rest in a smooth stationary horizontal circular pipe. One of the balls explodes, distintegrating into two fragments of different masses. Determine the final velocity of the body formed as a result of all collisions, assuming that the collisions are perfectly inelastic. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
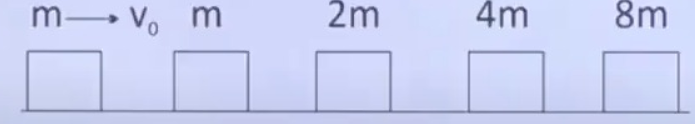
Consider on object of mass m moving with velocity v0 and all other masses at rest initially. Find the % loss in energy after final collision. If all collision are perfectly inelastic.
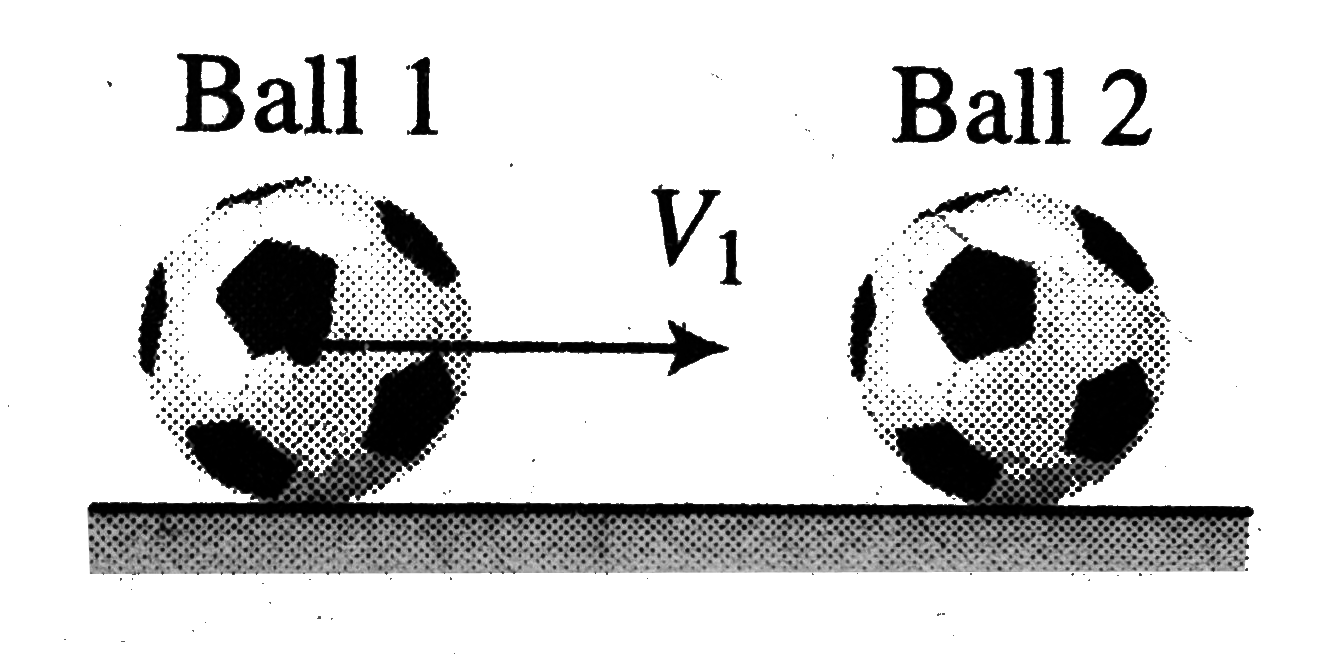
View SolutionA solid spherical ball rolling without slipping collides elastically with an identical ball at rest, as shown in the figure. Assuming that the frictional forces are small enough to have negligible effect during the instant of collision, calculate, the velocity of each ball along enough time after the collision when each ball is again rolling without slipping.
View Solution
Knowledge Check
A body of mass 10kg moving with a velocity of 5ms−1 hits a body of 1gm at rest. The velocity of the second body after collision. Assuming it to be perfectly elastic is
A10ms−1B5ms−1C15ms−1D0.10ms−1Velocity of the ball A after collision with the ball B as shown in the figure is (Assume perfectly inelastic and head - on collision)
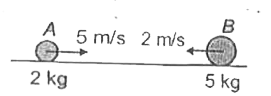 A37m/sB57m/sC17m/sDZero
A37m/sB57m/sC17m/sDZeroA body of mass 'm' moving with certain velocity collides with another identical body at rest. If the collision is perfectly elastic and after the collision both the bodies moves
Ain the same directionBin opposite directionCin perpendicular directionDat 45∘ to each other
Ball 1 collides with another identical ball at rest. For what value of coefficient of restitution e, the velocity of second ball becomes two times that of 1 after collision? _____.
View SolutionFour identical balls A,B,C and D are placed in a line on frictionless horizontal surface. A and D are moved with the same speed u towards the middle as shown. Assuming elastic collisions, find the final velocities.

View SolutionN identical balls are placed on a smooth horizontal surface. An another ball of same mass collides elastically with velocity u with first ball of N balls. A process of collision is thus started in which first ball collides with second ball and the with the third ball and so on. The coefficient of restitution for each collision is e. Find the speed of Nth ball.
View SolutionTwo identical ball bearings in contact with each other and resting on a frictionless table are hit heat-on by another ball bearing of the same mass moving initially with a speed V as shown in figure.
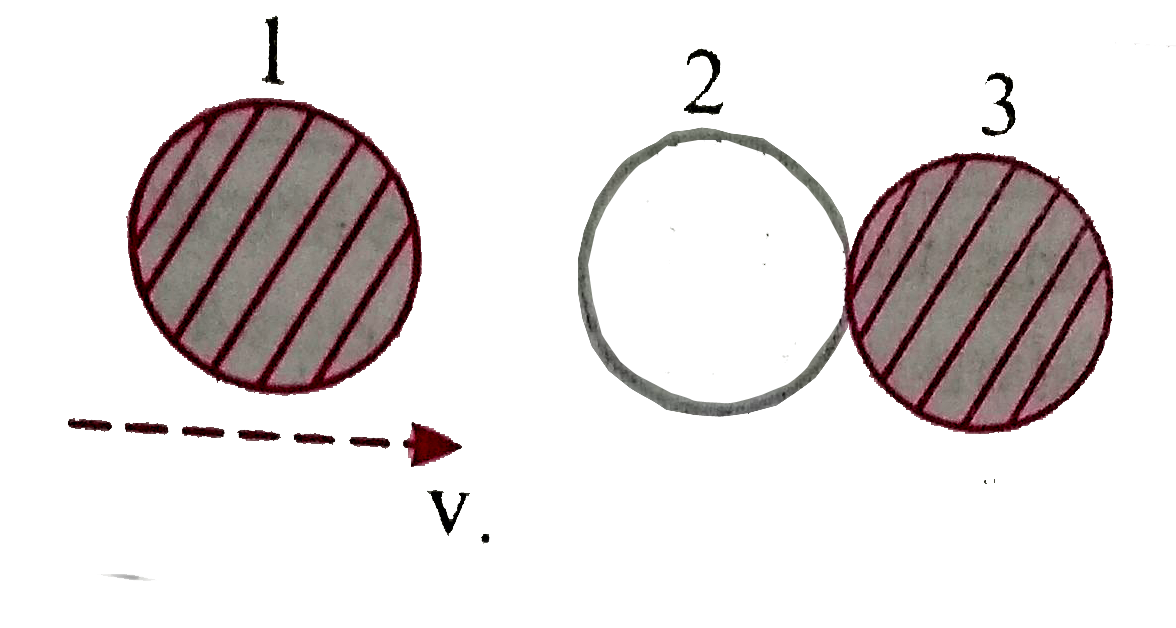
If the collision is elastic, which of the following (figure) is a possible result after collision ?
View SolutionTwo identical ball of radii r are kept on a horizontal plane with their centres d distance apart. A third ball, identical to previous one, collide elastically with both the balls symmetrically as shown in the figure. If the third ball comes to rest after the collision, d should be

View Solution
