In Figure, RENT is a rectangle. Its diagonals meet at O. Find x, if OR=2x+4 and OT=3x+1.
To solve the problem, we need to find the value of x given that OR=2x+4 and OT=3x+1 in rectangle RENT where the diagonals intersect at point O.
1. Understand the properties of a rectangle: In a rectangle, the diagonals bisect each other. This means that the segments from the center of the rectangle to the vertices are equal. Therefore, we can say that OR=OT.
2. Set up the equation: Since OR and OT are equal, we can set the expressions for OR and OT equal to each other:
2x+4=3x+1
3. Rearrange the equation: To solve for x, we need to isolate x. First, we can subtract 2x from both sides:
4=3x−2x+1
This simplifies to:
4=x+1
4. Solve for x: Next, subtract 1 from both sides to isolate x:
4−1=x
Therefore:
x=3
5. Conclusion: The value of x is 3.
2x+4=3x+1
4=3x−2x+1
This simplifies to:
4=x+1
4−1=x
Therefore:
x=3
Topper's Solved these Questions
Similar Questions
Knowledge Check
In the following list of English alphabets, one alphabet has not been used. Identify the same.
X N F A P S R W L T M D E X M G B C X Q J L O P V R C J Z O H S G O D I P T S M R A B E F G N U N EAVBICKDJ
RENT is a rectangle. Its diagonals meets at O. Find x if OR=2x+3 and OT=3x+2.
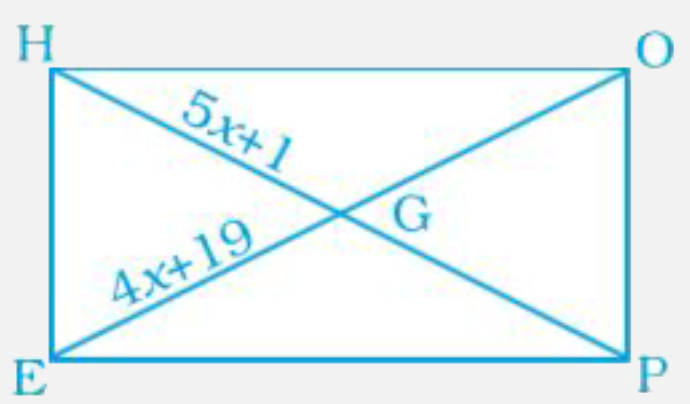
View SolutionIn the figure, HOPE is a rectangle. Its diagonals meet at G. If HG = 5x + 1 and EG = 4x + 19, find x.
View SolutionIn Figure, PQRS is a rhombus in which the diagonal PR is produced to T. If ∠SRT=1520, find x, y and z

View SolutionThe diagonals of a rectangle ABCD meet at O . If ∠BOC=44∘, find ∠OAD
View SolutionABCD is a parallelogram whose diagonals intersect at O. If P is any point on BO, prove that: ar (ADO)=AR (CDO) ar ( ABP)= ar ( CBP)
View SolutionShow that the diagonals of a parallelogram divide it into four triangles of equal area. GIVEN : A parallelogram ABCD . The diagonals AC and BD intersect at O. TO PROVE : ar(OAB)=ar(OBC)=ar(OCD)=ar(AOD)
View SolutionABCD is a parallelogram whose AC and BD intersect at O. A line through O intersects AB at P and DC at Q. Prove that ar ( POA)= ar ( QOC).
View Solution
