A particle having charge +q and mass m is approaching (head on) a free particle having mass M and charge 10 q. Initially the mass m is at large distance and has a velocity V0, whereas the other particle is at rest.
(a) Find the final velocity of the two particles when M=20 m.
(b) Find the final state of the two particles if M=m.
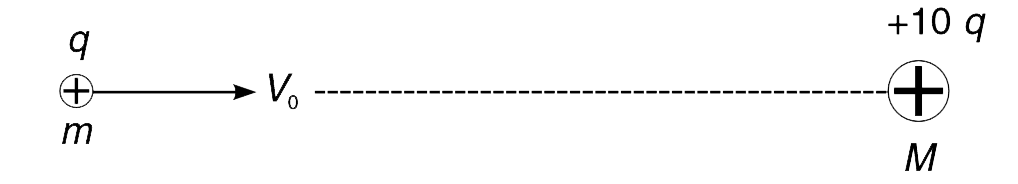
The correct Answer is:(a) The heavier particle moves with a velocity of 2V021 in the direction of →V0
Lighter particle moves with velocity 19V021 opposite to →V0
The incident particle comesn to rest. The other particle moves with →V0
Step by step video, text & image solution for A particle having charge +q and mass m is approaching (head on) a free particle having mass M and charge 10 q. Initially the mass m is at large distance and has a velocity V_(0), whereas the other particle is at rest. (a) Find the final velocity of the two particles when M = 20 m. (b) Find the final state of the two particles if M = m. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
A particle (A) having charge Q and mass m is at rest and is free to move. Another particle (B) having charge q and mass m is projected from a large distance towards the first particle with speed u. (a) Calculate the least kinetic energy of the system during the subsequent motion. (b) Find the final velocity of both the particles. Consider coulomb force only.
View SolutionA particle of mass m1 moves with velocity v1 and collides with another particle at rest of equal mass. The velocity of the second particle after the elastic collision is
View Solution
Knowledge Check
The reduce mass of two particles having masses m and 2 m is
A2 mB3 mC2 m/3Dm/2Two charged particles having charge 1μC and −μC and of mass 50gm each are held ar rest while their separtion is 2m. Find the relative velocity of the particles when their separation is 0.5m.
A15m/sB√35m/sC3√35m/sD3√310m/sIf the material particle is moving with velocity v and the velocity of light is c, then mass of particle is taken as (m0 is rest mass)
Am=m0cBm=m0√(1−v2c2)Cm=m0√(1+v2c2)Dm=m0√(1+v2c2)
Two point particles of mass m and 2m are initially separated by a distance 4a. They are then released to become free to move. Find the velocities of both the particles when the distance between them reduces to a.
View SolutionA particle having mass m and charge q is projected with a velocity v making an angle q with the direction of a uniform magnetic field B. Calculate the magnitude of change in velocity of the particle after time t=πm2qB.
View SolutionA charge particle of mass m and charge q is projected with velocity v along y-axis at t=0.

Find the velocity vector and position vector of the particle →v(t)and→r(t) in relation with time.
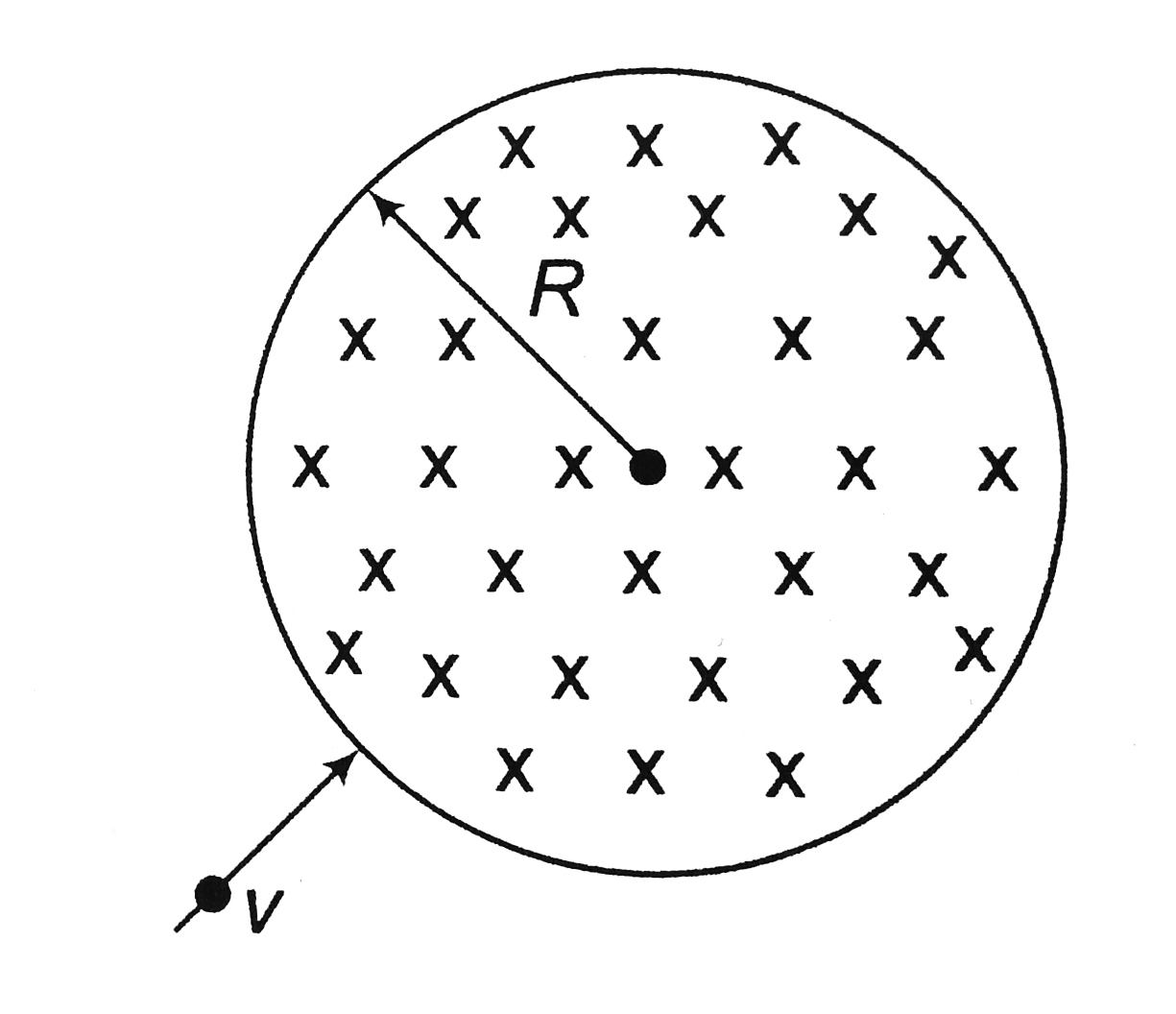
View SolutionA particle of mass m having a charge q enters into a circular region of radius R with velocity v directed towards the centre. The strength of magnetic field is B. Find the deviation in the path of the particle.
View SolutionA particle of mass m and charge q enters a magnetic field B perpendicularly with a velocity v, The radius of the circular path described by it will be
View Solution
