A ball moving with velocity $V_{0}$ , makes a head on collision with another identical ball at rest. The velocity of incident ball and the other ball after collision is $V_{1}$ and $V_{2}$ respectively.
(a) Using momentum conservation write an equation having $V_{1} and V_{2}$ as unknowns. Plot a graph of $V_{1}$ vs $V_{2}$ using this equation.
(b) Assuming the collision to be elastic write an equation for kinetic energy. Plot a graph of $V_{1}$ vs $V_{2}$ using this equation.
(c) The intersection point of the above two graphs gives solution. Find $V_{1} and V_{2}$ .
(d) In a particular collision, the plot of graphs mentioned above is as shown in figure

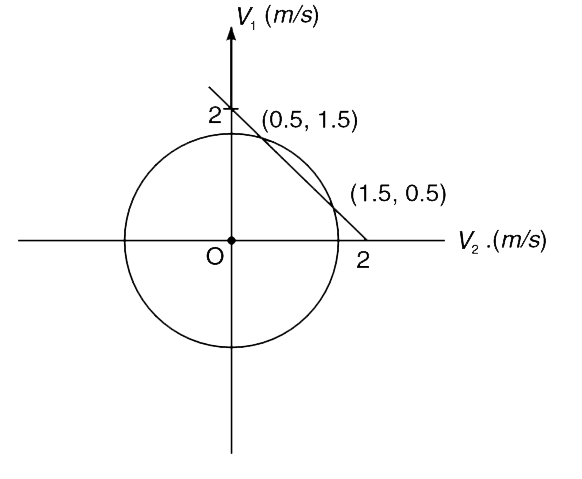
Find $V_{1} and V_{2}$ for this collision. Also write the percentage loss in kinetic energy during the collision.

Video Solution

Text Solution

Verified by Experts

The correct Answer is: $(# # I J A_{P} H Y_{V} 01_{C} 05_{E} 01_{021}_A 01 # #)$
$(# # I J A_{P} H Y_{V} 01_{C} 05_{E} 01_{021}_A 02 # #)$
$V_{1} = 0, V_{2} = V_{0}$
(d) $V_{1} = 0.5 m / s; V_{2} = 1.5 m / s; %$ loss in KE =37.5 %

Answer

Step by step video, text & image solution for A ball moving with velocity V_(0), makes a head on collision with another identical ball at rest. The velocity of incident ball and the other ball after collision is V_(1) and V_(2) respectively. (a) Using momentum conservation write an equation having V_(1) and V_(2) as unknowns. Plot a graph of V_(1) vs V_(2) using this equation. (b) Assuming the collision to be elastic write an equation for kinetic energy. Plot a graph of V_(1) vs V_(2) using this equation. (c) The intersection point of the above two graphs gives solution. Find V_(1) and V_(2) . (d) In a particular collision, the plot of graphs mentioned above is as shown in figure Find V_(1) and V_(2) for this collision. Also write the percentage loss in kinetic energy during the collision. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.

Updated on:21/07/2023

Class 11 PHYSICS CENTRE OF MASS

Topper's Solved these Questions

Knowledge Check

Question 1 - Select One
A ball of mass m moving with a speed v makes a head on collision with an identical ball at rest. The kinetic energy after collision of the balls is three fourth of the original kinetic energy. The coefficient of restitution is
A $\frac{1}{2}$
B $\frac{1}{3}$
C $\frac{1}{\sqrt{2}}$
D $\frac{1}{\sqrt{3}}$
Question 2 - Select One
A particle P moving with speed v undergoes a head -on elastic collision with another particle Q of identical mass but at rest. After the collision the collision
ABoth P and Q move forward with speed $\frac{v}{2}$
BBoth P and Q move forward with speed $\frac{v}{\sqrt{2}}$
CP comes of rest and Q moves forward with speed v
DP and Q move in opposite direction with speed $\frac{v}{\sqrt{2}}$ .
Question 3 - Select One
A ball of mass m moving with velocity v collides head-on which the second ball of mass m at rest. I the coefficient of restitution is e and velocity of first ball after collision is $v_{1}$ and velocity of second ball after collision is $v_{2}$ then
A $v_{1} = \frac{(1 - e) u}{2}, v_{2} = \frac{(1 + e) u}{2}$
B $v_{1} = \frac{(1 + e) u}{2}, v_{2} = \frac{(1 - e) u}{2}$
C $v_{1} = \frac{u}{2}, v_{2} = - \frac{u}{2}$
D $v_{1} = (1 + e) u, v_{2} = (1 - e) u$

ARIHANT-MOMENTUM AND CENTRE OF MASS-Momentum And Center of Mass

Home
Ask Doubt
Profile

The correct Answer is:(##IJAPHYV01C05E01021_A01##) (##IJAPHYV01C05E01021_A02##) V1=0,V2=V0 (d) V1=0.5m/s;V2=1.5m/s;% loss in KE =37.5 %

Topper's Solved these Questions

Similar Questions

Knowledge Check

A ball of mass m moving with a speed v makes a head on collision with an identical ball at rest. The kinetic energy after collision of the balls is three fourth of the original kinetic energy. The coefficient of restitution is

A particle P moving with speed v undergoes a head -on elastic collision with another particle Q of identical mass but at rest. After the collision the collision

A ball of mass m moving with velocity v collides head-on which the second ball of mass m at rest. I the coefficient of restitution is e and velocity of first ball after collision is v1 and velocity of second ball after collision is v2 then

ARIHANT-MOMENTUM AND CENTRE OF MASS-Momentum And Center of Mass

The correct Answer is: $(# # I J A_{P} H Y_{V} 01_{C} 05_{E} 01_{021}_A 01 # #)$
$(# # I J A_{P} H Y_{V} 01_{C} 05_{E} 01_{021}_A 02 # #)$
$V_{1} = 0, V_{2} = V_{0}$
(d) $V_{1} = 0.5 m / s; V_{2} = 1.5 m / s; %$ loss in KE =37.5 %

A ball of mass m moving with velocity v collides head-on which the second ball of mass m at rest. I the coefficient of restitution is e and velocity of first ball after collision is $v_{1}$ and velocity of second ball after collision is $v_{2}$ then