Triangles ABC and +DBC are on the same base BC with A, D on opposite side of line BC, such that ar(⊥ABC)=ar(DBC). Show that BC bisects AD.
Given: Triangles ABC and DBC are on the same base BC with A, D on opposite side of line BC, such that ar(△ABC)=ar(△DBC).
To prove: BC bisects AD
Construction: Draw AL⊥BC and DM⊥BC.
Proof : ar(△ABC)=ar(△DBC) [Given]
⇒BC×AL2=BC×DM2
⇒AL=DM ....(i)
Now, In △OAL and ΔOMD
AL=DM [From (i)]
⇒∠ALO=∠DMO [Each=90∘]
⇒∠AOL=∠MOD [Vert. opp. ∠s]
⇒∠OAL=∠ODM [Third angles of the triangles]
∴△OAL=~△OMD [By ASA]
∴OA=OD [By CPCT]
∴BC bisects AD
Topper's Solved these Questions
Similar Questions
In figure ABC and DBC are two triangles on the same base BC. If AD intersects BC at O, show that ar(ABC)ar(DBC)=AODO.

View SolutionA point D is taken on the side BC of a ABC such that BD=2dC. Prove that ar(ABD)=2ar(ADC).
View SolutionTriangles on the same base and between the same parallels are equal in area. GIVEN : Two triangles ABC and PBC on the same base BC and between the same parallel lines BC and AP. TO PROVE : ar(ABC)=ar(PBC) CONSTRUCTION : Through B, draw BDCA intersecting PA produced in D and through C, draw CQBP, intersecting line AP in Q.
View SolutionIn a parallelogram ABCD,E,F are any two points on the sides AB and BC respectively. Show that ar(ADF)=ar(DCE).
View SolutionD and E are points on sides AB and AC respectively of ΔABCsuch thatar (DBC) = ar (EBC). Prove that DE ∣∣ BC.
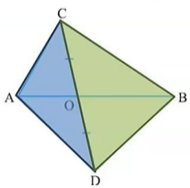
View SolutionDiagonals AC and BD of a quadrilateral ABCD intersect at O in such a way that ar (AOD)=ar ( BOC). Prove that ABCD is a trapezium.
View SolutionIn Fig. 4.179, ABC and DBC are on the same base BC . If AD and BC intersect at O , prove that Area (ABC)Area (DBC)=AODO (FIGURE)
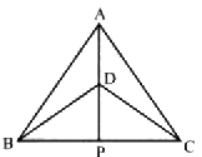
View SolutionABC and DBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC . If AD is extended to intersect BC at P, show that ABD≅ACD (ii) ABP≅ACP AP bisects ∠A as well as ∠D AP is perpendicular bisector of BC.
View Solutionp AND q are any two points lying on the sides DC and AD respectively of a parallelogram ABCD. Show that ar(APB)=ar (BQC).
View SolutionABC and DBC are two isosceles triangles on the same bas BC and vertices A and D are on the same side of BC . If AD is extended to intersect BC at P, show that AP bisects \ ∠A as well as ∠D AP is the perpendicular bisector of BC
View Solution
