XY isa line parallel to side BC of ABC.BEA and CFAB meet XY in E and F respectively.Show that ar(ABE)=ar(ACF).
Given: △ABC, BE∣∣AC and CF∣∣AB
To prove: ar(ABE)=ar(ACF)
Proof: since YE∣∣BC and BE∣∣CY
BCYE is a parallelogram.
Similarly, BCFX is a parallelogram.
Parallelograms BCFX and BCYE are on the same base BC and between same parallels BC and EF,
∴ar(BCYE)=ar(BCFX) …(i)
ar(ABE)=12ar(BCYE) …(ii) (on same base and between same parallels)
Similarly, ar(ACF)=12ar(BCFX) …(iii)
From (i), (ii) and (iii), we get:
ar(ABE)=ar(ACF)
Hence proved.
Topper's Solved these Questions
Similar Questions
XY is a line parallel to side BC of a triangle ABC. If BE ∣∣ ACand CF ∣∣ ABmeet XY at E and F respectively, show that ar (ABE) = ar (ACF)
View SolutionIn a parallelogram ABCD,E,F are any two points on the sides AB and BC respectively. Show that ar(ADF)=ar(DCE).
View SolutionIn Figure, ABCD, ABFE and CDEF are parallelograms. Prove that ar(ADE)=ar(BCF)
View SolutionABCD is a trapezium with AB∣∣DC . A line parallel to AC intersects AB at X and BC at Y. Prove that ar(ADX)=ar(ACY).
View SolutionABCD is a trapezium with ABDC. A line parallel to AC intersects AB at X and BC at Y. Prove that ar(ADX)=ar(ACY).
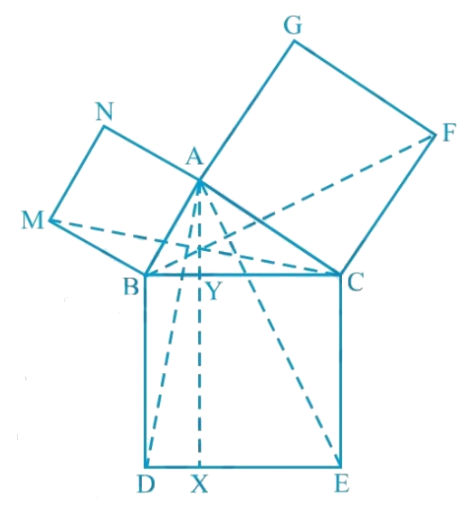
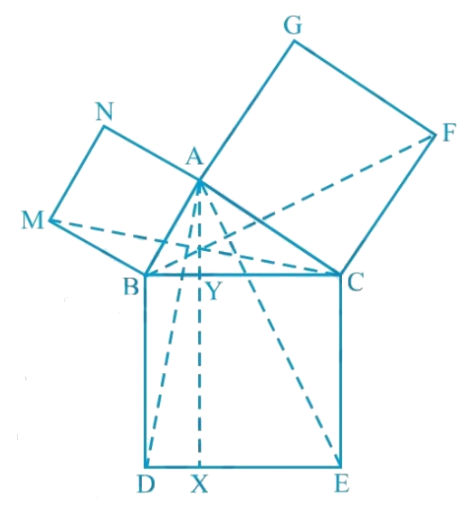
View SolutionIn Figure, ABC is a right triangle right angled at A, BCED, ACFG and AMN are square on the sides BC, CA and AB respectively. Line segment AX ⊥DE meets BC at Y. Show that: ar (CYXE)=2ar( FCB)
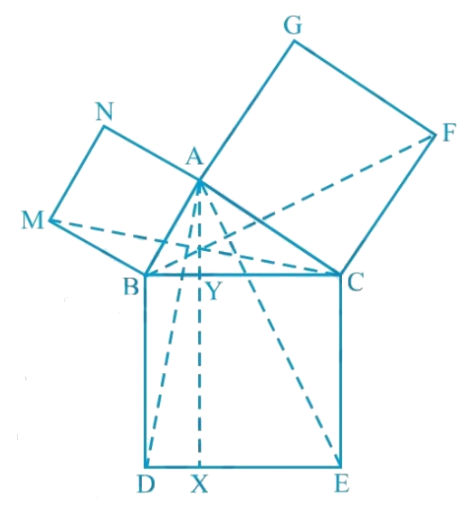
View SolutionIn Figure, ABC is a right triangle right angled at A, BCED, ACFG and AMN are square on the sides BC, CA and AB respectively. Line segment AX ⊥DE meets BC at Y. Show that: ar (CYXE)= ar (ACFG)
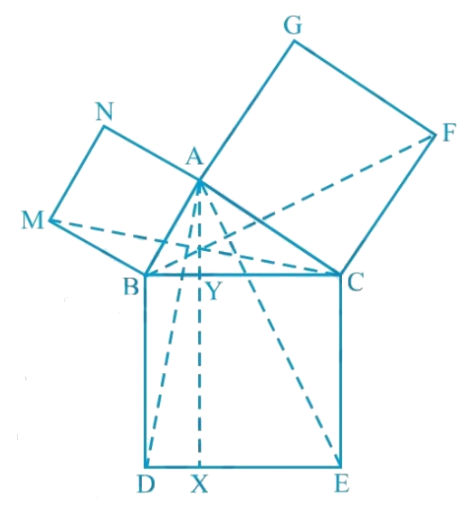
View SolutionIn Figure, ABC is a right triangle right angled at A, BCED, ACFG and AMN are square on the sides BC, CA and AB respectively. Line segment AX ⊥DE meets BC at Y. Show that: ar(CYXE)=ar (ACFG)
View SolutionABCD is a parallelogram in which BC is produced to E such that CE=BC.AE intersects CD at F. Prove that ar ( ADF)= ar ( ECF) If the area of DFB=3 cm2, find the area of ^(gm) ABCD
View SolutionIn Figure, ABC is a right triangle right angled at A, BCED, ACFG and AMN are square on the sides BC, CA and AB respectively. Line segment AX ⊥DE meets BC at Y. Show that: ar (BYXD)=2 ar ( MBC)
View Solution