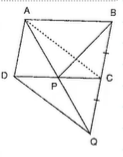
ABCD
is a parallelogram whose diagonals AC
and BD
intersect at O.
A Line through O
intersects AB
at P
and DC
at Q.
Prove that ar(△POA)=ar(△QOC).
Given:
ABCD is a parallelogram
To prove:
ar(△POA)= ar(△GOC)
Proof:
In△POAand△QOC
We have
⇒∠AOP=∠COQ [vertically opposite angle]
⇒AO=OC [ Diagonals of parallalogram bisect each other]
⇒∠PAC=∠OCA [Alternate interior angles]
∴△POA≅△QOC [ By ASA congruece]
We know that congruent figures are equal in areas
∴ar(△POA)=ar(△QOC)
Hence Proved.
Topper's Solved these Questions
Similar Questions
ABCD is a parallelogram whose diagonals AC and BD intersect at O. A Line through O intersects AB at P and DC at Q. Prove that ar(△POA)=ar(△QOC).
View SolutionABCD is a parallelogram whose diagonals AC and BD intersect at O. A line through O intersects AB at P and DC at Q. Prove that ar (ΔPOA)= ar (ΔQOC).
View SolutionABCD is a parallelogram whose AC and BD intersect at O. A line through O intersects AB at P and DC at Q. Prove that ar ( POA)= ar ( QOC).
View SolutionThe diagonals of a parallelogram ABCD intersect at OA line through O intersects AB at XandDC at Y Prove that OX=OY
View SolutionThe diagonals of a parallelogram ABCD intersect at O.A line through O intersects AB at X and DC at Y. Prove that OX=OY.
View SolutionABCD is a trapezium with ABDC. A line parallel to AC intersects AB at X and BC at Y. Prove that ar(ADX)=ar(ACY).
View SolutionThe diagonals of a parallelogram ABCD intersect at O. A line through O meets AB in X and CD in Y. Show that ar(AXYD)=12(ARgmABCD)
View SolutionABCD is a trapezium with AB∣∣DC . A line parallel to AC intersects AB at X and BC at Y. Prove that ar(ADX)=ar(ACY).
View SolutionABCD is a parallelogram whose diagonals intersect at O. If P is any point on BO, prove that: ar (ADO)=AR (CDO) ar ( ABP)= ar ( CBP)
View SolutionABCD is a cyclic quadrilateral whose diagonals AC and BD intersect at P. If AB=DC, prove that:
(i)PAB ≅PDC
(ii)PA=PD and PC=PB (iii) AD∣∣BC
View Solution
