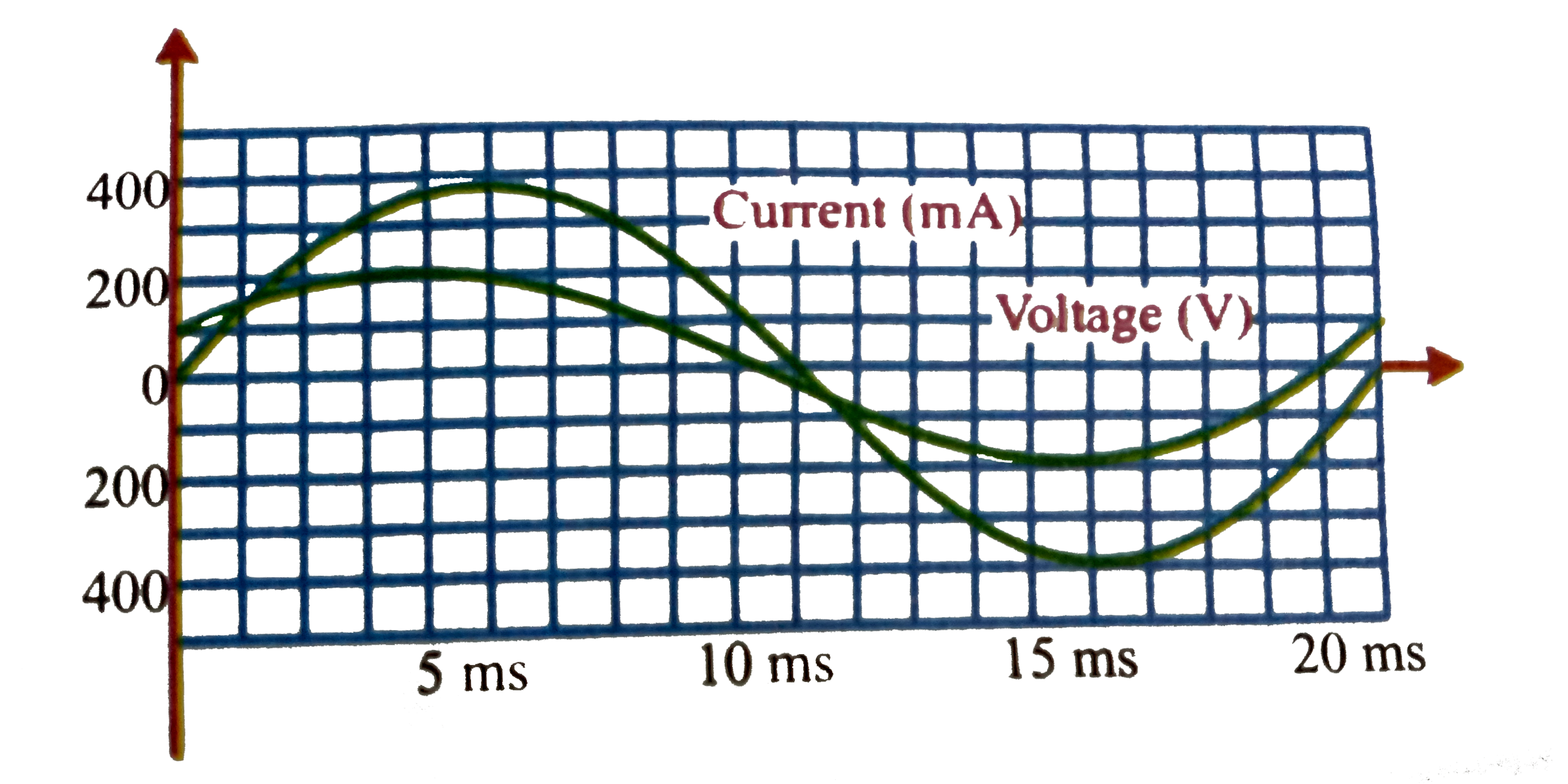
The given graph shows variation with time in the source voltage and steady state current drawn by a series RLC circuit. Given curve through origin is the current variation.
Which of the following statements is/are correct ?
A
current lag the voltage
B
Resistance in the circuits is 250√3Ω
C
If capacitive reactance is 74Ω, inductance in the circuit is approximately 560mH.
D
Average power dissipation in the circuit is 20√3W.
Video Solution
Text Solution
The correct Answer is:A,B,D
From graph i=400sinωt in mA
V=200sin(ωt+ϕ) in volt
At t=0v=100 volt hence sinϕ=12,ϕ=π6
cosϕ=R2, then R=2cosϕ=250√3Ω
Pav=V0I0cosϕ=20√3 watt
|
Topper's Solved these Questions
- ALTERNATING CURRENTBOOK - NARAYNACHAPTER - ALTERNATING CURRENTEXERCISE - LEVEL - I (H.W)
23 Videos
- ALTERNATING CURRENTBOOK - NARAYNACHAPTER - ALTERNATING CURRENTEXERCISE - LEVEL - II(H.W)
13 Videos
- ALTERNATING CURRENTBOOK - NARAYNACHAPTER - ALTERNATING CURRENTEXERCISE - LEVEL - V
83 Videos
- ATOMIC PHYSICSBOOK - NARAYNACHAPTER - ATOMIC PHYSICSEXERCISE - LEVEL-II (H.W)
14 Videos
Similar Questions
Knowledge Check
When current (I) in R-L series circuit becomes constant, where L is a pure inductor, which of the following given statements is/are correct?
 Avoltage across R is RI.BSome part (not 100%) of the energy supplied by the battery will be dissipated in R and remaining will continue to store in L.Cvoltage across L is equal to zero.Dmagnetic energy stored is 12LI2
Avoltage across R is RI.BSome part (not 100%) of the energy supplied by the battery will be dissipated in R and remaining will continue to store in L.Cvoltage across L is equal to zero.Dmagnetic energy stored is 12LI2The variation of the instantaneous current (I) and the instantaneous e.m.f (E) in a circuit is as shown in figure. Which of the following statement is correct?
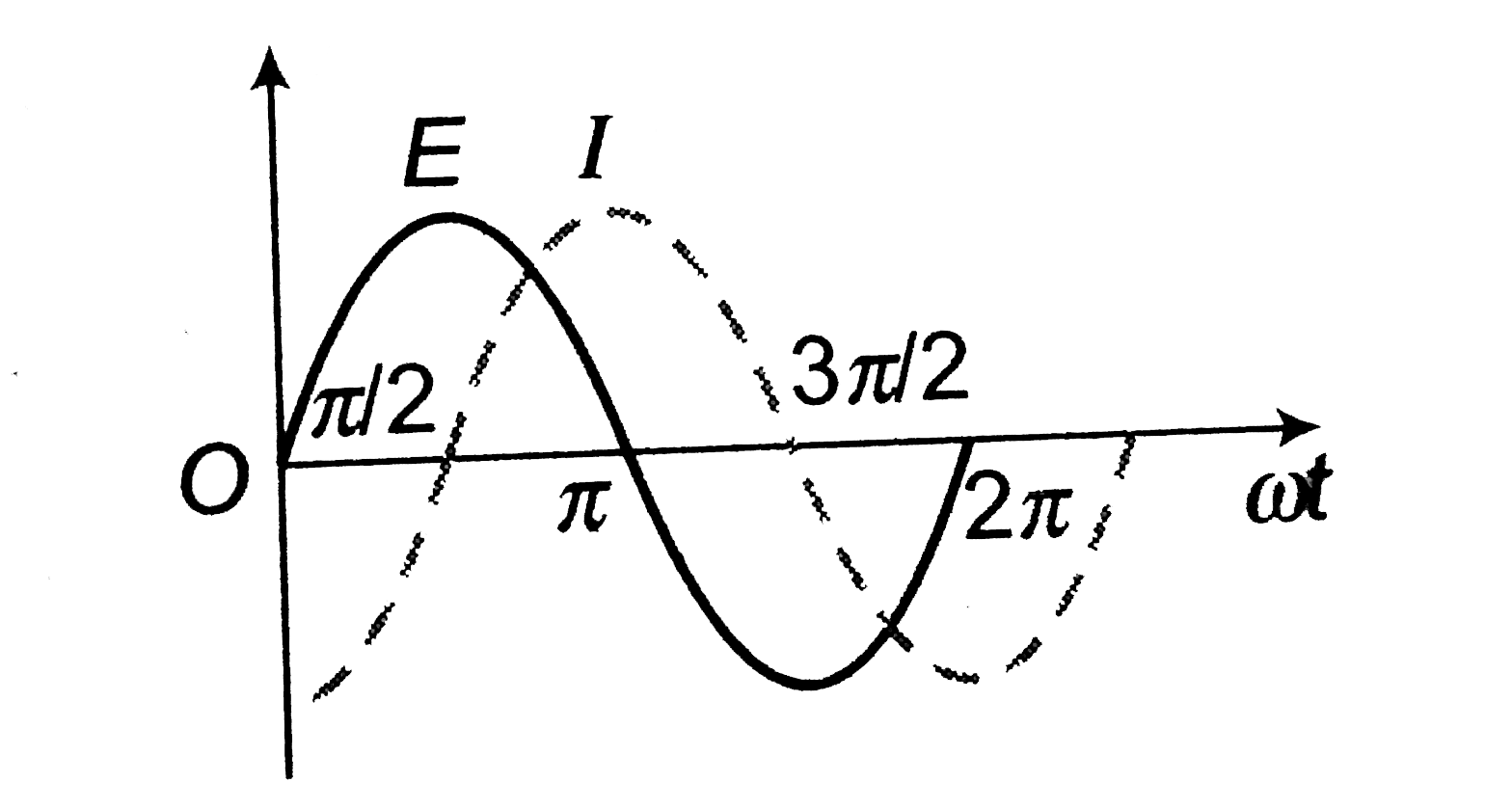 AThe voltage lags behind the current by π/2BThe voltage leads the current by π/2CThe voltage and the current are in phaseDThe voltage leads the current by π
AThe voltage lags behind the current by π/2BThe voltage leads the current by π/2CThe voltage and the current are in phaseDThe voltage leads the current by πIn the given circuit the current drawn from the source is
 .A20AB10AC5AD5√2A
.A20AB10AC5AD5√2A
A series L-C-R circuit is given in figure . The current through the circuit is
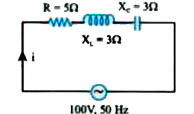
View SolutionShow that the time for attaining half the value of the final steady current in LR-series circuit is 0.693(L/R).
View SolutionFind the current drawn from source in each of the circuits as given below
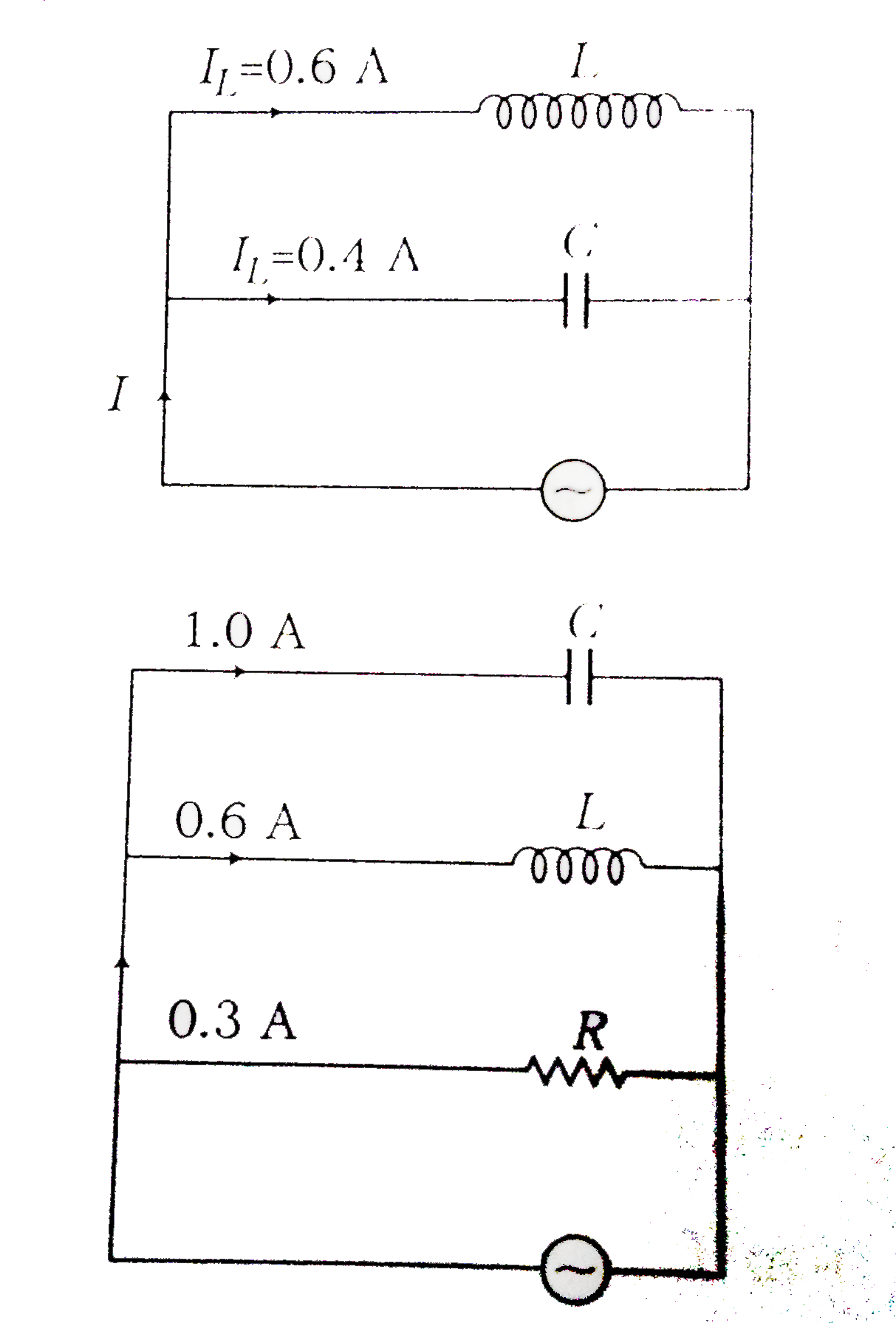
View SolutionIs the graph showing variation of current versus voltage for a diode linear or non-linear?
View SolutionThe phase difference between voltage and current in an LCR series circuit is
View Solution
