If various elements, i.e., resistance capacitance and inductance which are in series and having values 1000Ω,1μF and 2.0H respectively. Given emf as, V=100√2sin1000t volts
voltages across the capacitor is
70.7 Volts
102 Volts
141.4 Volts
270.7 Volts
The correct Answer is:A
P.D. Across capalitor Vc=irmsXc
=0.0707×11×1000×10−6=70.7 volts
Topper's Solved these Questions
- ALTERNATING CURRENTBOOK - NARAYNACHAPTER - ALTERNATING CURRENTEXERCISE - LEVEL - I (H.W)
23 Videos
- ALTERNATING CURRENTBOOK - NARAYNACHAPTER - ALTERNATING CURRENTEXERCISE - LEVEL - II(H.W)
13 Videos
- ALTERNATING CURRENTBOOK - NARAYNACHAPTER - ALTERNATING CURRENTEXERCISE - LEVEL - V
83 Videos
- ATOMIC PHYSICSBOOK - NARAYNACHAPTER - ATOMIC PHYSICSEXERCISE - LEVEL-II (H.W)
14 Videos
Similar Questions
Find the voltage across the various elements, i.e., resistance, capacitance and inductance which are in series and having values 1000Ω,1μF and 2.0 H respectively . Given emf as,
V = 100sqrt2 sin 1000 t V
View SolutionFind the voltage across the various elements, i.e., resistance, capacitance and inductance which are in series and having values 100Ω,1μF and 2.0H, respectively. Given emf is
V=100√2sin1000t volt
View Solution
Knowledge Check
If various elements, i.e., resistance capacitance and inductance which are in series and having values 1000Ω,1μF and 2.0H respectively. Given emf as, V=100√2sin1000t volts
voltage across the inductor isA70.7 VoltsB101 VoltsC141.4 VoltsD270.7 VoltsIf various elements, i.e., resistance capacitance and inductance which are in series and having values 1000Ω,1μF and 2.0H respectively. Given emf as, V=100√2sin1000t volts
Voltage across the resistor isA70.7 VoltsB100 VoltsC141.4 VoltsD270.7 VoltsA 100Ω resistance is connected in series with a 4H inductor. The voltage across the resistor is VR=2sin(1000t)V. The voltage across the inductors is
A80sin(1000t+π2)B40sin(1000t+π2)C80sin(1000t−π2)D40sin(1000π−π2)
Find the potential difference across resistance, capacitance and inductance in series LCR circuit where L=2.0H,C=1μFandR=100Ω. The applied emf has the form V=100√2sin(1000t)
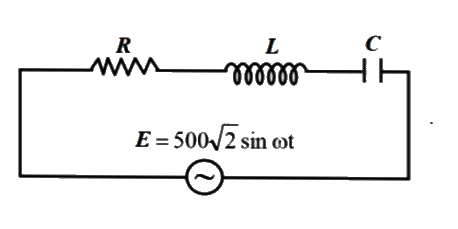
View SolutionIn the series L−C−R circuit shown in the figure, the rms voltage across the resistor and inductor are 400 V and 700 V respectively. If the applied voltage is E=500√2sin(ωt), then the peak voltage across the capacitor is
View SolutionA resistor of 500Ω and an inductance of 0.5 H are in series with an ac source which is given by V=100√2sin(1000t). The power factor of the combination is
View SolutionA choke coil of resistance 5Ω and inductance 0.6 H is in series with a capacitance of 10(μ)F. If a voltage of 200 V is applied and the frequency is adjusted to resonance, the current and voltage across the inductance and capacitance are (I0),(V0)and(V1) respectively. we have
View SolutionAn alternating e.m.f. of 0.1 V is applied across an LCR series circuit having R=2Ω,C=40μFandL=100mH. At resonance, the voltage drop across the inductor is
View Solution
