Two paper screens and are separated by a distance of . A bullet pierces and . The hole in is below the hole in . If the bullet is travelling horizontally at the time of hitting the screen , calculate the velocity of the bullet when it hits the screen . Neglect resistance of paper and air.
To solve the problem, we will analyze the motion of the bullet as it travels horizontally and falls vertically under the influence of gravity.
1. Understanding the Problem:
- The bullet travels horizontally and pierces two screens, A and B, which are 100 m apart.
- The hole in screen B is 10 cm (or 0.1 m) below the hole in screen A.
2. Identify the Vertical Motion:
- The bullet falls 0.1 m vertically while traveling horizontally 100 m.
- The vertical motion can be treated as free fall under gravity, where the initial vertical velocity vy0=0.
3. Using the Second Equation of Motion:
- The vertical displacement s can be described by the equation:
s=vy0t+12gt2
- Here, s=0.1 m (the vertical distance fallen), vy0=0, and g (acceleration due to gravity) is approximately 9.81m/s2.
- Substituting the values:
0.1=0+12⋅9.81⋅t2
- This simplifies to:
0.1=4.905t2
4. Solving for Time t:
- Rearranging gives:
t2=0.14.905
- Calculating t2:
t2≈0.0204⇒t≈√0.0204≈0.142s
5. Horizontal Motion:
- The horizontal distance traveled is 100 m, and the horizontal velocity vx remains constant since there is no horizontal acceleration.
- The relationship between distance, velocity, and time is:
Distance=Velocity×Time
- Thus:
100=vx⋅t
- Substituting t:
100=vx⋅0.142
- Solving for vx:
vx=1000.142≈704.2m/s
6. Final Result:
- The velocity of the bullet when it hits screen A is approximately 704.2m/s.
- The bullet travels horizontally and pierces two screens, A and B, which are 100 m apart.
- The hole in screen B is 10 cm (or 0.1 m) below the hole in screen A.
- The bullet falls 0.1 m vertically while traveling horizontally 100 m.
- The vertical motion can be treated as free fall under gravity, where the initial vertical velocity vy0=0.
- The vertical displacement s can be described by the equation:
s=vy0t+12gt2
- Here, s=0.1 m (the vertical distance fallen), vy0=0, and g (acceleration due to gravity) is approximately 9.81m/s2.
- Substituting the values:
0.1=0+12⋅9.81⋅t2
- This simplifies to:
0.1=4.905t2
- Rearranging gives:
t2=0.14.905
- Calculating t2:
t2≈0.0204⇒t≈√0.0204≈0.142s
- The horizontal distance traveled is 100 m, and the horizontal velocity vx remains constant since there is no horizontal acceleration.
- The relationship between distance, velocity, and time is:
Distance=Velocity×Time
- Thus:
100=vx⋅t
- Substituting t:
100=vx⋅0.142
- Solving for vx:
vx=1000.142≈704.2m/s
- The velocity of the bullet when it hits screen A is approximately 704.2m/s.
Topper's Solved these Questions
- MOTION IN A PLANEBOOK - NARAYNACHAPTER - MOTION IN A PLANEEXERCISE - C.U.Q
36 Videos
- MOTION IN A PLANEBOOK - NARAYNACHAPTER - MOTION IN A PLANEEXERCISE - Level-I(C.W)
43 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NARAYNACHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - LEVEL-II (H.W)
24 Videos
- MOTION IN A STRAIGHT LINEBOOK - NARAYNACHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - Level 2 H.W
29 Videos
Similar Questions
A bullet travels horizontally at 660m/s at a height of 5 m from a man. How far is the bullet from the man when he hears its whistle ? Velocity of sound in air =340m/s.
View SolutionTwo wooden blocks of masses 1kg and 2kg are separated by a certain distance A bullet of mass 50g fired from a gun pierces through the block of mass 1kg and then stopped in the second block After the impact of the bullet both blocks start moving with the same speed Calculate the percentage loss in the initial velocity of the bullet when it is inbetween the two blocks .
View Solution
Knowledge Check
Two paper screens A and B are separated by a distance of 200 m. A bullet pierces A and then B. The hole in B is 40 cm below the hole in A. If the bullet is travelling horizontally at the time of hitting A, then the velocity of the bullet at A is
A200ms−1B400ms−1C600ms−1D700ms−1Two paper screens A and B are separated by 150m. A bullet pierces A and B. The hole in B in 15 cm below the hole in A. If the bullet is travelling horizontally at the time of hitting A, then the velocity of the bullet at A is: (g=10ms−2)
A100√3ms−1B200√3ms−1C300√3ms−1D500√3ms−1Two paper screens A and B are separated by distance 100 m. A bullet penetrates A and B, at points P and Q respectively, where Q is 10 cm below P. If bullet is travelling horizotally at the time of hitting A, the velocity of bullet at A is nearly
A100 m/sB200 m/sC600 m/sD700 m/s
To understand the effect of air resistance on the motion of a bullet let's consider a bullet of the shape shown in the fig. The bullet is flying horizontally. The cross section of cylindrical part is A and the conical part has a semi vertical angle of 45∘. Assume that the bullet is fired with initial velocity u and moves in a gaseous medium in which molecules are at rest (do you think this assumption is necessary?). Collisions of the molecules with the bullet are elastic. Take mass of bullet to be M, density of gaseous medium as ρ and disregard gravity.
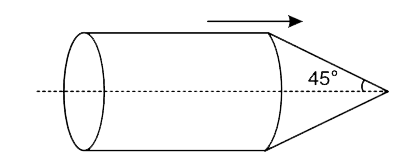
(a) Consider two bullets one small and other large, made of same material. Which will experience larger retardation due to air resistance ?
(b) Write the speed of bullet after time t
(c) Write distance travelled by the bullet in time t.
View SolutionA bullet hits a wall with a velocity of 20 m/s and penetrates it up to a distance of 5 cm. Find the deceleration of the bullet in the wall.
View SolutionPoints A and C are on the horizontal ground and A and B are in same vertical plane at a distance of 1500m. Simultaneously bullets are fired from A,Bll and C and they collide at O. The bullets at B is fired at an angle of 30∘with horizontal towards the ground iat velocity 100m/s. the bullet at C is projected vertically upwards at velocity of 100m/s. the bullet projected from A reaches its maximum height at O.
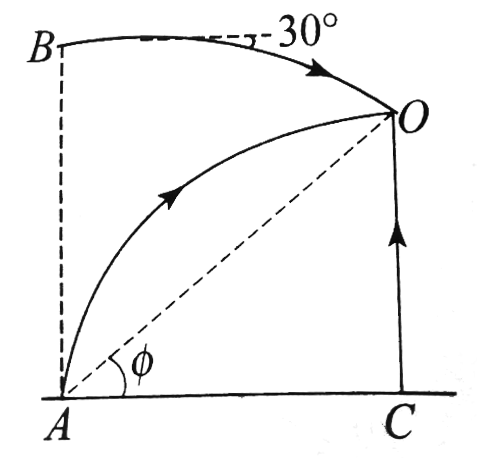
Find the time in which bullets will collide (seconds ):
View SolutionA 10.0-g bullet traveling horizontally at 755 m/s strikes a stationary target and stops after penetrating 14.5 cm into the target. What is the average force of the target on the bullet ?
View SolutionTwo thin wood screens A and B are separated by 200m a bullet travelling horizontally at speed of 600m/s hits the screen A penetrates through it and finally emerges out from B making holes in A and B the resistance of air and wood are negligible the difference of heights of the holes in A and B is.
View Solution
