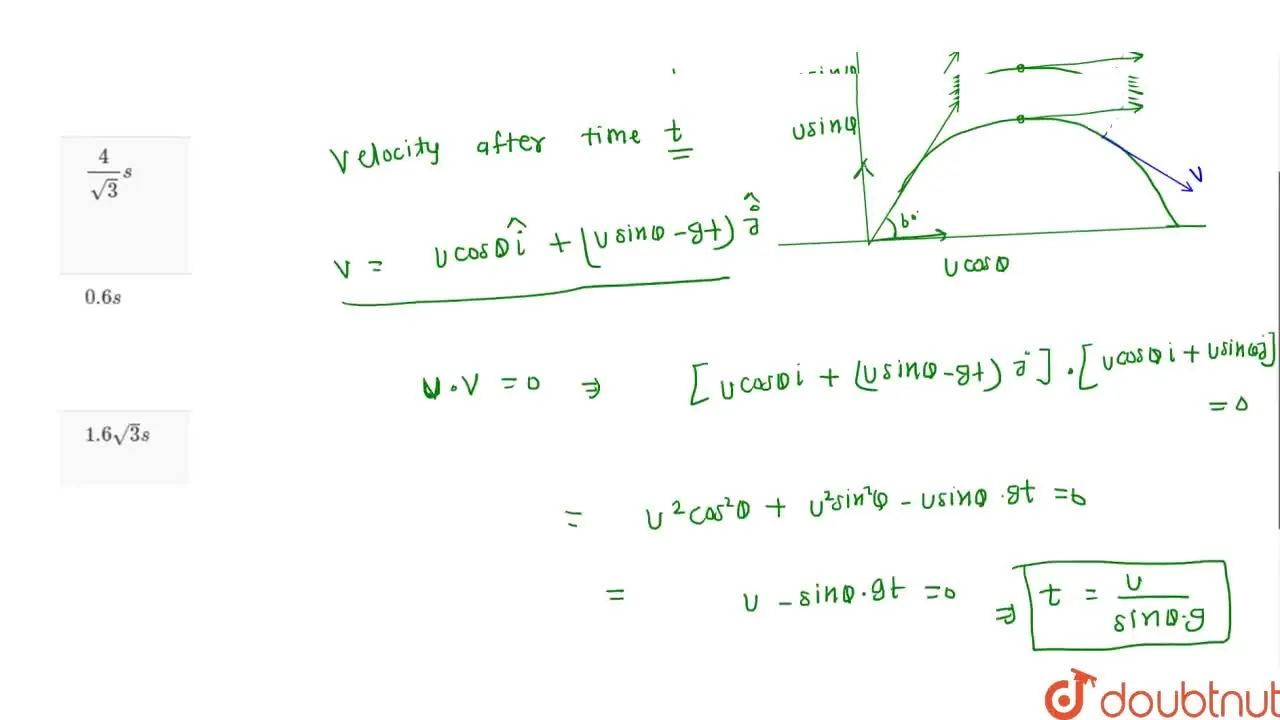A ball is thrown with velocity making an angle with the horizontal.Its velocity will be perpendicular to the direction of initial velocity of projection after a time of
The correct Answer is:A
Related Playlists
- BOOK - NARAYNACHAPTER - MOTION IN A PLANEEXERCISE - Level-I (H.W)41videos
MOTION IN A PLANE
- BOOK - NARAYNACHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - LEVEL-II (H.W)24videos
MECHANICAL PROPERTIES OF SOLIDS
- BOOK - NARAYNACHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - Level 2 H.W29videos
MOTION IN A STRAIGHT LINE
Similar Questions
" A body is projected with velocity u making an angle 300 with the horizontal.Its velocity when it is perpendicular to the initial velocity vector is "
View SolutionA body is thrown with a velocity of 9.8m/s making an angle of 30∘ with the horizontal. It will hit the ground after a time
View SolutionA ball is thrown with a velocity of u making an angle θ with the horizontal. Its velocity vector normal to initial vector (u) after a time interval of
View SolutionA ball is thrown with speed u at an angle θ with horizontally. After how much time it will move perpendicular to the initial direction of motion.
View SolutionIf a particle is projected with a velocity 49m/s making an angle 60∘ with the horizontal, its time of flight is
View SolutionIf a particle is projected with a velocity 49m/s making an angle 60∘ with the horizontal, its time of flight is
View SolutionA particle is projected with a velocity of 20√3ms−1 at an angle of 60∘ to the horizontal.At the moment,the direction of motion of the projectile is perpendicular to the direction of initial velocity of projection, its velocity is?
View SolutionA particle is projected with a velocity u making an angle θ with the horizontal. At any instant its velocity becomes v which is perpendicular to the initial velocity u. Then v is
View SolutionA particle is projected with a velocity of 20√3ms−1 at an angle of 60∘ to the horizontal. At the moment, the direction of motion of the projectile is perpendicular to the direction of initial velocity of projection, its velocity is
View SolutionA body is projected with a velocity 60ms−1at30∘ to horizontal . Its initial velocity vector is
View Solution