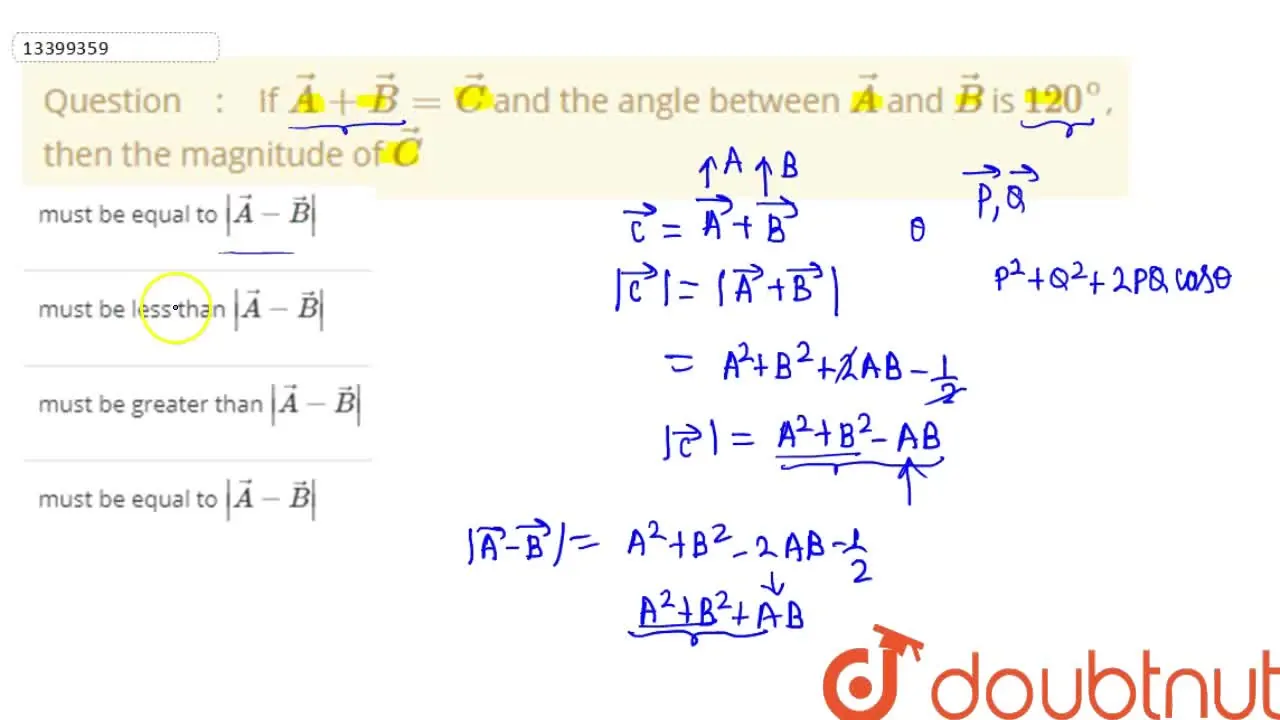
If →A+→B=→C and the angle between →A and →B is 120∘, then the magnitude of →C
must be equal to ∣∣→A−→B∣∣
must be less than ∣∣→A−→B∣∣
must be greater than ∣∣→A−→B∣∣
must be equal to ∣∣→A−→B∣∣
The correct Answer is:B
Step by step video, text & image solution for If vec A+vec B=vec C and the angle between vec A and vec B is 120^(@), then the magnitude of vec C by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Related Playlists
- BOOK - NARAYNACHAPTER - MOTION IN A PLANEEXERCISE - Level-I(C.W)43videos
MOTION IN A PLANE
- BOOK - NARAYNACHAPTER - MOTION IN A PLANEEXERCISE - Level-II(C.W)45videos
MOTION IN A PLANE
- BOOK - NARAYNACHAPTER - MOTION IN A PLANEEXERCISE - Level-II(H.W)31videos
MOTION IN A PLANE
- BOOK - NARAYNACHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - LEVEL-II (H.W)24videos
MECHANICAL PROPERTIES OF SOLIDS
- BOOK - NARAYNACHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - Level 2 H.W29videos
MOTION IN A STRAIGHT LINE
Similar Questions
If →a,→b,→c are unit vectors such that →a.→b=0=→a.→c and the angle between →band→c is π/3 , then the value of ∣∣→a×→b−→a×→c∣∣ is 1/2 b. 1 c. 2 d. none of these
View SolutionIf →A+→B=→C and A+B=C, then the angle between →A and →B is :
View Solution.Vectors →A,→B and →c are shown in figure.Find angle between (i) →A and →B. (ii) →A and →C. ,(iii)vec Bandvec C
View SolutionIf →a.→band→c are unit vectors such that →a is perpendicular to the plane of →b,→c and the angle between →band→c is π3. Then what is ∣∣→a+→b+→c∣∣ ?
View SolutionGiven →A+→B+→+→D=0, can the magnitude of →A+→B+→C be equal to the magnitude of vec D Explain.
View SolutionTwo vectors vec A and vec B have equal magnitudes.The magnitude of (vec A+vec B) is 'n' times the magnitude of (vec A-vec B).The angle between vec A and vec B is:
View SolutionAssertion: If ∣∣→A+→B∣∣=∣∣→A−→B∣∣, then angle between →A and →B is 90∘
Reason: →A+→B=→B+→AView SolutionIf →a,→b,→c are unit vectors such that →a is perpendicular to the plane of →b,→c, and the angle between →band→c is π3
Then, what is ∣∣→a+→b+→c∣∣?View SolutionLet →a,→b,→c are the three vectors such that |→a|=∣∣→b∣∣=|→c|=2and angle between →aand→b is π3,→band→c and π3 and →aand→cπ3.If →a,→band→c are sides of parallelogram
View SolutionIf →A=→B+→C and the values of →A,→Band→C are 13, 12 and 5 respectively, then the angle between →A and →C will be
View Solution