Three identical charges are fixed at the corners of an equilateral triangle . If the force between any two charges is F, then the net electric force on each will be
√2F
2F
√3F
3F
The correct Answer is:3
Let's solve the problem step by step.
Step 1: Understand the Problem
We have three identical charges, Q, placed at the corners of an equilateral triangle. The force between any two charges is F. We need to find the net electric force on each charge.
Step 2: Diagram and Forces
Consider an equilateral triangle with charges Q at vertices A, B, and C. The side length of the triangle is d.
1. The force between charges at A and B is F.
2. The force between charges at B and C is F.
3. The force between charges at A and C is F.
Step 3: Resolve Forces into Components
For a charge at vertex A, the forces due to charges at B and C need to be resolved into components.
1. The force F due to charge at B acts along the line AB.
2. The force F due to charge at C acts along the line AC.
Since AB and AC form a 60∘ angle (equilateral triangle), we resolve these forces into x and y components.
Step 4: Components of Forces
1. The force F along AB can be resolved into:
- Fcos(30∘) along the x-axis
- Fsin(30∘) along the y-axis
2. The force F along AC can be resolved into:
- Fcos(30∘) along the x-axis (opposite direction)
- Fsin(30∘) along the y-axis
Step 5: Net Force Calculation
Since the x-components of the forces along AB and AC cancel each other out, we only need to consider the y-components.
1. The y-component of the force due to charge at B is Fsin(30∘).
2. The y-component of the force due to charge at C is Fsin(30∘).
Both y-components add up because they are in the same direction:
Fnet=2×Fsin(30∘)
Step 6: Substitute Values
We know that sin(30∘)=12:
Fnet=2×F×12=F
Step 7: Final Answer
The net electric force on each charge is:
Fnet=F
We have three identical charges, Q, placed at the corners of an equilateral triangle. The force between any two charges is F. We need to find the net electric force on each charge.
Consider an equilateral triangle with charges Q at vertices A, B, and C. The side length of the triangle is d.
2. The force between charges at B and C is F.
3. The force between charges at A and C is F.
For a charge at vertex A, the forces due to charges at B and C need to be resolved into components.
2. The force F due to charge at C acts along the line AC.
1. The force F along AB can be resolved into:
- Fcos(30∘) along the x-axis
- Fsin(30∘) along the y-axis
- Fcos(30∘) along the x-axis (opposite direction)
- Fsin(30∘) along the y-axis
Since the x-components of the forces along AB and AC cancel each other out, we only need to consider the y-components.
2. The y-component of the force due to charge at C is Fsin(30∘).
Fnet=2×Fsin(30∘)
We know that sin(30∘)=12:
Fnet=2×F×12=F
The net electric force on each charge is:
Fnet=F
Topper's Solved these Questions
Similar Questions
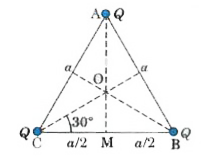
Three point charges are kept at the three corners of an equilateral triangle of edge length a, as shown in the figure. The net electric dipole moment of the system is

View SolutionThree identical point charges Q are placed at the three corners of an equilateral triangle of side length a. Find the electric potential at points O and M due to the three charges.
View Solution
Knowledge Check
Three identical charges are placed at corners of a equilateral triangel of side l. If force between any two charges is F, the work required to double the dimensiions of triangle is
A−3FlB3FlC(−3/2)FlD(3/2)FlThree identical charges are placed at the vertices of an equilateral triangle. The force experienced by each charge, (if k=14πε0) is
A2Kq2r2BKq22r2C√3Kq2r2DKq2√2r2Three charge +Q,+Q and −Q are placed at the corners of an equilateral triangle. The ratio of the force on a positive charge to the force on the negative charge will be equal to
A1B2C√3D1/√3
Charge of 5μC each are placed at the corners of an equilateral triangle of side 10 cm. Then the force on each charge is :-
View SolutionThree point charges are placed at the corner of an equilateral triangle. Assuming only electrostatic forces are acting.
View SolutionThree charges each20μC are placed at the corners of an equilateral triangle of side 0.4m The potential energy of the system is
View SolutionTwo identical charges are placed at the two corners of an equilateral triangle. The potential energy of the system is U. The work done in bringing an identical charge from infinity to the third vertex is
View SolutionThree charges +Q each are placed at the corners A,B and C of an equilateral triangle. At the circumcenre, O the electric field wil be
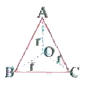
View Solution
