Two particles of mases m and m' moving on parallel straight lines are at the distance 'a' apart with velocities v and v'(v>v'). The particles are connected by a string of length (>a) which was loose in the beginning. Calculate the impluse of tension of the string when it becomes taut.
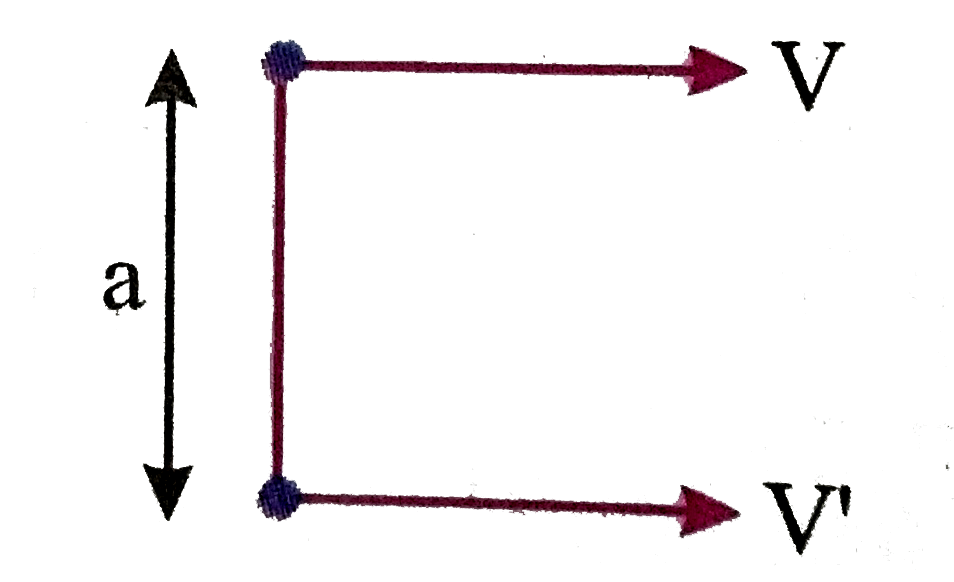
The correct Answer is:mm'(v−v')√l2−a2(m+m')l
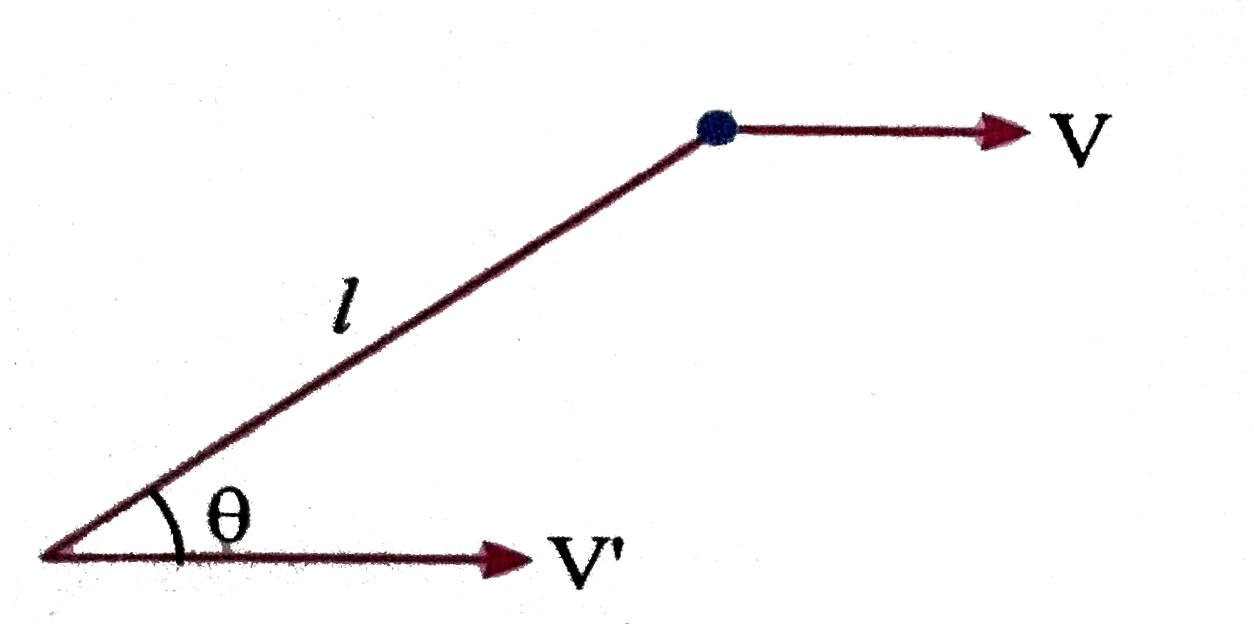
Just before string becomes taut

Just after string becomes taut.
TΔt=m(Vcosθ−V''),TΔt=m'(V''−V'cosθ)
⇒v''=(mv+m'v')cosθ(m+m')
TΔt=J=mm'(V−V')√l2−a2(m+m')l
Topper's Solved these Questions
- COLLISIONBOOK - NARAYNACHAPTER - COLLISIONEXERCISE - Level-I (H.W)
52 Videos
- COLLISIONBOOK - NARAYNACHAPTER - COLLISIONEXERCISE - Level-II (H.W)
54 Videos
- COLLISIONBOOK - NARAYNACHAPTER - COLLISIONEXERCISE - Comprehension type
35 Videos
- CIRCULAR MOTIONBOOK - NARAYNACHAPTER - CIRCULAR MOTIONEXERCISE - LEVEL II(H.W)
51 Videos
- FRICTIONBOOK - NARAYNACHAPTER - FRICTIONEXERCISE - Passage type of questions I
6 Videos
Similar Questions
Two particles of masses m and 2m moving in opposite directions collide elastically with velocity 2v and v, respectiely. Find their velocities after collision.

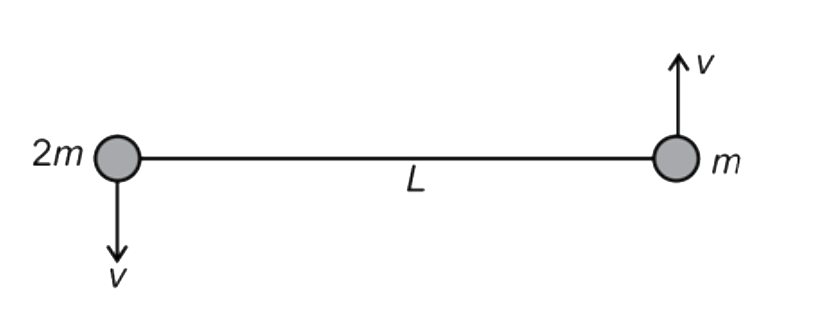
View SolutionTwo particles of mass m and 2m are connected by a string of length L and placed at rest over a smooth horizontal surface. The particles are then given velocities as indicated in the figure shown. The tension developed in the string will be
View Solution
Knowledge Check
Two particles of mass m and 2 m moving in opposite directions collide elastically with velocities v and 2v. Find their velocities after collision.
A0, 3vB1, 3vC0, 1vD0, 4vForce acting on a particle of mass m moving in straight line varies with the velocity of the particle as F=K/V K is constant then speed of the particle in time t
A√ktmB√2ktmC2√ktmDnone of theseA mass m is hanging by a string of length l. The velocity v0 which must be imparted to it to just reach the top is
A√3glB√4glC√5glD√6gl
A particle is moving in a straight line under constant acceleration alpha. If the intial velocity is v0, sketch the v-t and s-t graphs. (v0>0).
View SolutionThe velocity v of a particle at any instant t moving in a straight line is given by v=s +1 where s meter is the distance travelled in t second what is the time taken by the particle to cover a distacne of 9m ?
View SolutionA particle of mass m attached to an inextensible light string is moving in a vertical circle of radius r. The critical velocity at the highest point is v0 to complete the vertical circle. The tension in the string when it becomes horizontal is
View SolutionTwo particles are moving with velocities v1andv2 . Their relative velocity is the maximum, when the angle between their velocities is
View SolutionA particle of mass m is moving with a uniform velocity v1. It is given an impulse such that its velocity becomes v2. The impulse is equal to
View Solution
