Two particles are moving with velocities v1andv2 . Their relative velocity is the maximum, when the angle between their velocities is
zero
π/4
π/2
π
The correct Answer is:D
To solve the problem of finding the angle between the velocities of two particles when their relative velocity is maximum, we can follow these steps:
1. Understanding Relative Velocity:
The relative velocity →vrel of two particles moving with velocities →v1 and →v2 is given by:
→vrel=→v1−→v2
2. Magnitude of Relative Velocity:
The magnitude of the relative velocity can be calculated using the formula:
|→vrel|=|→v1−→v2|=√|→v1|2+|→v2|2−2|→v1||→v2|cos(α)
where α is the angle between the two velocity vectors.
3. Maximizing the Magnitude:
To find the angle α that maximizes the relative velocity, we need to maximize the expression:
|→vrel|=√|→v1|2+|→v2|2−2|→v1||→v2|cos(α)
4. Setting Up the Condition for Maximum:
The expression inside the square root will be maximized when cos(α) is minimized. The minimum value of cos(α) is -1, which occurs when α=180∘.
5. Conclusion:
Therefore, the angle α that maximizes the relative velocity of the two particles is:
α=180∘
Final Answer:
The angle between the velocities v1 and v2 for maximum relative velocity is 180∘.
---
The relative velocity →vrel of two particles moving with velocities →v1 and →v2 is given by:
→vrel=→v1−→v2
The magnitude of the relative velocity can be calculated using the formula:
|→vrel|=|→v1−→v2|=√|→v1|2+|→v2|2−2|→v1||→v2|cos(α)
where α is the angle between the two velocity vectors.
To find the angle α that maximizes the relative velocity, we need to maximize the expression:
|→vrel|=√|→v1|2+|→v2|2−2|→v1||→v2|cos(α)
The expression inside the square root will be maximized when cos(α) is minimized. The minimum value of cos(α) is -1, which occurs when α=180∘.
Therefore, the angle α that maximizes the relative velocity of the two particles is:
α=180∘
The angle between the velocities v1 and v2 for maximum relative velocity is 180∘.
Topper's Solved these Questions
- KINEMATICSBook:MOTIONChapter:KINEMATICSExercise:EXERCISE 2 OBJECTIVE PROBLEMS
Explore 75 Videos
- KINEMATICSBook:MOTIONChapter:KINEMATICSExercise:EXERCISE 2 OBJECTIVE PROBLEMS (Level-2)
Explore 26 Videos
- KINEMATICSBook:MOTIONChapter:KINEMATICSExercise:EXERCISE 4 PREVIOUS YEAR (LEVEL 2)
Explore 6 Videos
- HYDROSTATIC, FLUID MECHANICS & VISCOSITYBook:MOTIONChapter:HYDROSTATIC, FLUID MECHANICS & VISCOSITYExercise:EXERCISE -3 (SECTION-B) PREVIOUS YEAR PROBLEM
Explore 7 Videos
- KINETIC THEORY OF GASES Book:MOTIONChapter:KINETIC THEORY OF GASES Exercise:EXERCISE-3 SECTION-B
Explore 16 Videos
Similar Questions
Two particles 1and2 move with velocities →v1 and →v2 making the angles θ1andθ2 with the line joining them, respectively. Find angular velocity of relative→1.
 .
.
View SolutionTwo particles AandB are moving with constant velocities v1andv2. At t=0, v1 makes an angle θ0 with the line joining AandB and v2 makes an angle θ2 with the line joining AandB. Find their velocity of approach.
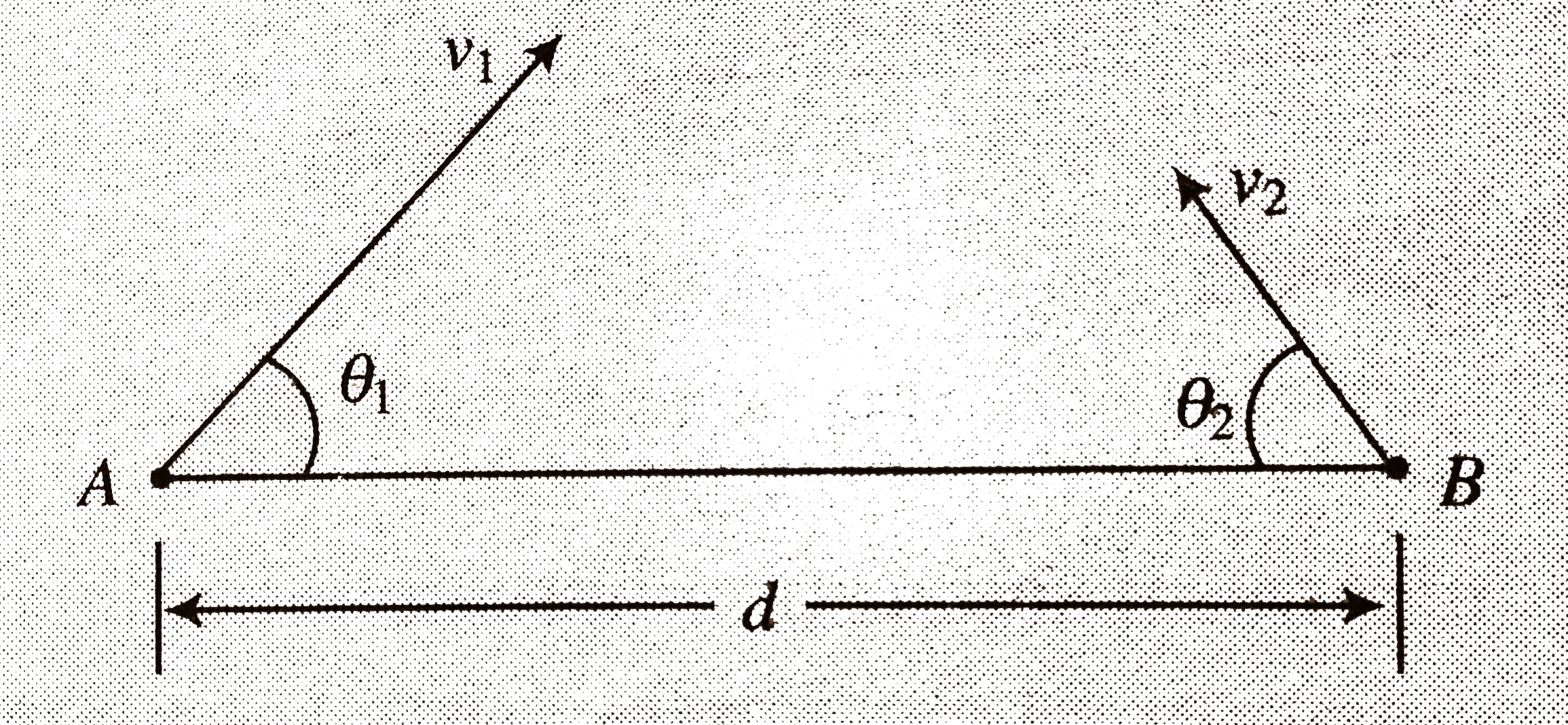 .
.
View Solution
Knowledge Check
Two balls are thrown horizontally from the top of a tower with velocities v1 and v2 in opposite directions at the same time. After how much time the angle between velocities of balls becomes 90∘ ?
A2√v1v2gB√v1v2gC√v1v22gDg√v1v2Statement-I : If two particles, moving with constant velocities are to meet, the relative velocity must be along the line joining the two particles.
Statement-II : Relative velocity means motion of one particle as viewed from the other.AStatement-I is true, Statement-II is true, Statement-II is correct explanation for Statement-IBStatement-I is true, Statement-II is true, Statement-II is NOT a correct explanation for Statement-ICStatement-I is true, Statement-II is falseDStatement-I is false, Statement-II is true.Assertion: If two particles, moving with constant velocities are to meet, the relative velocity must be along the line joining the two particles.
Reason: Relative velocity means motion of one particle as viewed from the other.AIf both assertion and reason are true and reason is the correct explanation of assertion.BIf both assertion and reason are true but reason is not the correct explanation of assertion.CIf assertion is true but reason is false.DIf assersion is false but reason is true.
Two bodies A and B are moving with velocities vA and vB, making an angle θ with each other. Determine the relative velocity of A w.r.t. B. What will be the relative velocity when the two bodies move in opposite directions ?
View SolutionTwo bodies A and B are moving with velocities vA and vB, making an angle θ with each other. Determine the relative velocity of A w.r.t. B. What will be the relative velocity when the two bodies move in same direction
View Solution(A): Relative velocity of A w.r.t. B is greater than the velocity of either, when they are moving in opposite directions.
(R): The relative velocity between any two bodies is equal to the sum of the velocities of the two bodies.
View SolutionTwo bodies of masses m1 and m2 are moving with velocity v1 and v2 respectively in the same direction. The total momentum of the system in the frame of reference attached to the centre of mass is (v is relative velocity between the masses)
View SolutionA particle moves with initial velocity v0 and retardation αv, where v is velocity at any instant t. Then the particle
View Solution