Two bodies A and B are moving with velocities vA and vB, making an angle θ with each other. Determine the relative velocity of A w.r.t. B. What will be the relative velocity when the two bodies move in same direction
To determine the relative velocity of body A with respect to body B, we can follow these steps:
Step 1: Define the velocities
Let:
- →vA be the velocity of body A.
- →vB be the velocity of body B.
- The angle between the velocities →vA and →vB is θ.
Step 2: Write the relative velocity formula
The relative velocity of A with respect to B is given by:
→vAB=→vA−→vB
Step 3: Break down the velocities into components
Assuming →vA is along the x-axis, we can express →vB in terms of its components:
- The x-component of →vB is vBcos(θ).
- The y-component of →vB is vBsin(θ).
Thus, we can write:
→vB=vBcos(θ)^i+vBsin(θ)^j
Step 4: Substitute the components into the relative velocity formula
Now substituting the components into the relative velocity equation:
→vAB=vA^i−(vBcos(θ)^i+vBsin(θ)^j)
This simplifies to:
→vAB=(vA−vBcos(θ))^i−vBsin(θ)^j
Step 5: Calculate the magnitude of the relative velocity
The magnitude of the relative velocity |→vAB| can be calculated using the Pythagorean theorem:
|→vAB|=√(vA−vBcos(θ))2+(vBsin(θ))2
Step 6: Analyze the case when θ=0
When θ=0, both bodies A and B are moving in the same direction. In this case:
- cos(0)=1
- sin(0)=0
Substituting these values into the relative velocity formula:
→vAB=(vA−vB)^i+0^j
Thus, the relative velocity simplifies to:
→vAB=(vA−vB)^i
Final Answer
The relative velocity of A with respect to B is:
|→vAB|=√(vA−vBcos(θ))2+(vBsin(θ))2
And when θ=0:
→vAB=(vA−vB)^i
---
Let:
- →vA be the velocity of body A.
- →vB be the velocity of body B.
- The angle between the velocities →vA and →vB is θ.
The relative velocity of A with respect to B is given by:
→vAB=→vA−→vB
Assuming →vA is along the x-axis, we can express →vB in terms of its components:
- The x-component of →vB is vBcos(θ).
- The y-component of →vB is vBsin(θ).
→vB=vBcos(θ)^i+vBsin(θ)^j
Now substituting the components into the relative velocity equation:
→vAB=vA^i−(vBcos(θ)^i+vBsin(θ)^j)
This simplifies to:
→vAB=(vA−vBcos(θ))^i−vBsin(θ)^j
The magnitude of the relative velocity |→vAB| can be calculated using the Pythagorean theorem:
|→vAB|=√(vA−vBcos(θ))2+(vBsin(θ))2
When θ=0, both bodies A and B are moving in the same direction. In this case:
- cos(0)=1
- sin(0)=0
→vAB=(vA−vB)^i+0^j
Thus, the relative velocity simplifies to:
→vAB=(vA−vB)^i
The relative velocity of A with respect to B is:
|→vAB|=√(vA−vBcos(θ))2+(vBsin(θ))2
And when θ=0:
→vAB=(vA−vB)^i
Topper's Solved these Questions
Similar Questions
Two bodies A and B are moving with velocities vA and vB, making an angle θ with each other. Determine the relative velocity of A w.r.t. B. What will be the relative velocity when the two bodies move in opposite directions ?
View SolutionTwo objects AandB are moving along the directions as shown in (Fig. 5.77). Find the magnitude and direction of the relative velocity of Bw.r.t.A.
 ,
,
View Solution
Knowledge Check
Two objects A and B are moving in opposite directions with velocities vAandvB respectively, the magnitude of relative velocity of A w.r.t. B is
AvA−vBBvA+vBCvB−vADvA/vBTwo particles are moving with velocities v1andv2 . Their relative velocity is the maximum, when the angle between their velocities is
AzeroBπ/4Cπ/2DπBuses A and B are moving in the same direction with speed 20ms−1 and 15ms−1 respectively. Find the relative velcoity of A w.r.t. B and relative velocity of B w.r.t. A.
A(15ms−1)ˆi (−5ms−1)ˆiB(5ms−1)ˆi (−15ms−1)ˆiC(5ms−1)ˆi (−5ms−1)ˆiD(5ms−1)ˆi (15ms−1)ˆi
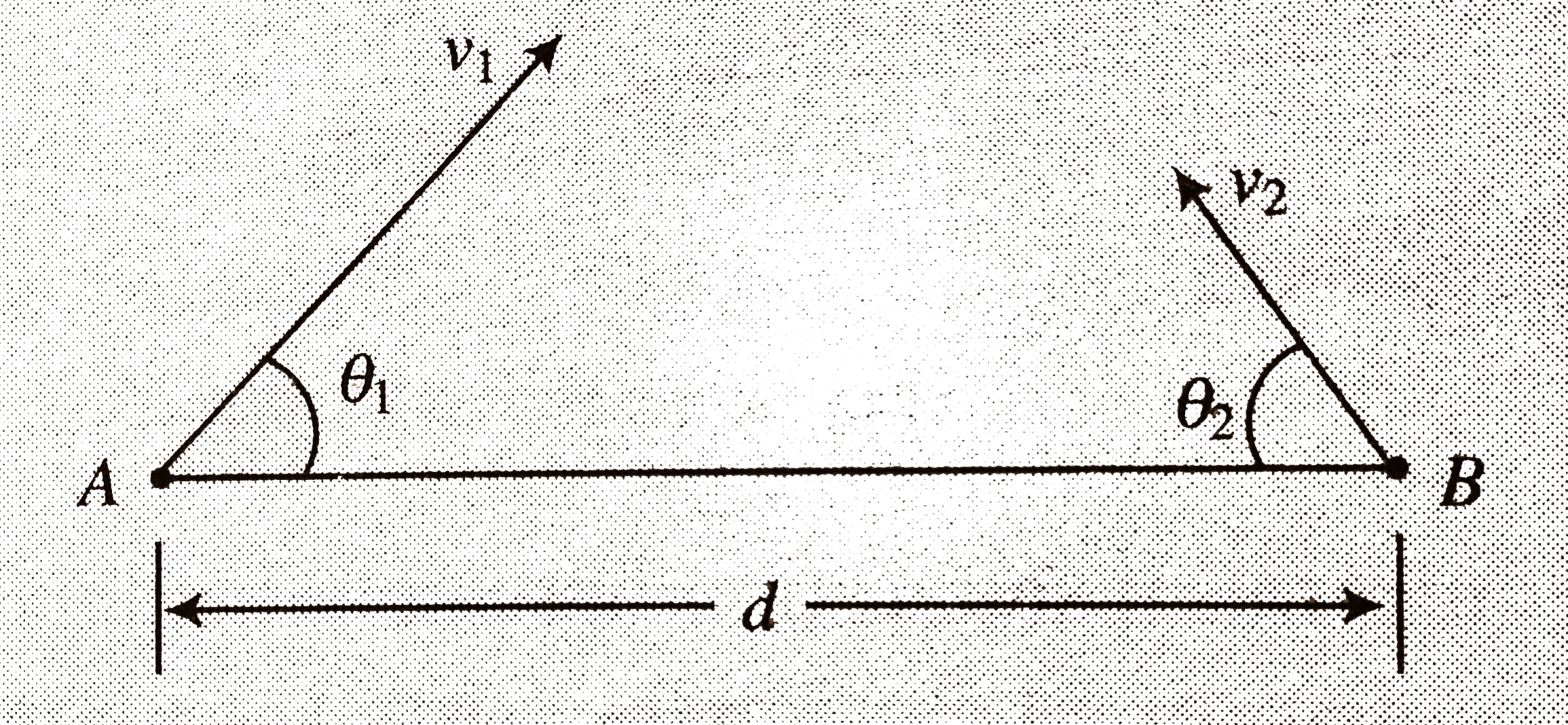
Two particles 1and2 move with velocities →v1 and →v2 making the angles θ1andθ2 with the line joining them, respectively. Find angular velocity of relative→1.
 .
.
View SolutionA and B are moving with the same speed 10m/s in the direction E−30∘−N and E−30∘−S respectively. Find the relative velocity of A w.r.t. B.
View SolutionTwo particles AandB are moving with constant velocities v1andv2. At t=0, v1 makes an angle θ0 with the line joining AandB and v2 makes an angle θ2 with the line joining AandB. Find their velocity of approach.
 .
.
View SolutionA boat is moving with a velocity 3ˆi−4ˆj w.r.t ground. The water in the river is moving with a velocity −3ˆi−4ˆj w.r.t ground. What is the relative velocity of boat w.r.t water.
View SolutionTwo particles A and B start moving with velocities 20 m/s and 30√2 m/s along x-axis and at an angle 45∘ with x- axis respectively in xy-plane from origin the relative velocity of B w.r.t A
View Solution